links
 3D
3D
 4D
4D


| Site Map | Polytopes | Dynkin Diagrams | Vertex Figures, etc. | Incidence Matrices | Index |
Compounds are defined to be multicovers of a single spot by more than just a single polytope. Sure there are infinitely many such possibilities, even continuously related. So the more interesting case comes up, when there is an overall symmetry (not needed to be the same of the used polytopes), under the action of which the total compound is preserved. Sure, there are slightly shifted copies too, which make up such a symmetrical compound: the center of that shift vector serves for inversonal symmetry. Thus, the symmetrical compounds of real interest would require an overall symmetry, which at least is the same (or probably larger) than that of its components. Examples here are all compounds of dual pairs (which by definition have the same overall symmetry as each component – except for selfduals, where symmetry is increased by the exchange of those 2 components) or even the ones given in the lower part of plate 7 (there, in the first 2 cases, componenets are considered under pyritohedral symmetry only, while the compound symmetry comes out to be full cubical).
Compounds moreover are called uniform, if within the definition of polytopal uniformity we replace vertex transivity by vertex figures transivity. This is because for compounds it might be possible to have still no completely coincident elements of subdimension larger than 0, but having coincident vertices none the less. There, this stated demand asks for some symmetry, which interchanges those coincident vertices of the related components as well.
As an aside one might ask whether this uniformity already requires compounds to be isohedral. This can be answered kind of depending: Consider 2 (or more) vertex coincident isohedral compounds (or polytopes), both of the same edge-length, and take the (mere) compound of those. The above stated demand now would require some symmetry which interchanges the vertex figures of the components. This generally would not work. But if we thereafter would identify coincident vertices, the picture changes. We no longer have coincident vertices with single vertex figures each, but we get single vertices with compound vertex figures. Consequently that additional above requirement brakes down, as there is nothing left to be interchanged. (It only remains the question, whether we still have true compounds, or if we would have changed this status, slightly towards mere polytopes, by the application of identification of coinciding vertices.)
Accordingly, more generally we could consider compounds of (possibly) different components, whenever those components have the same circumradius and moreover are inscribed into a common convex hull, while the subset of components of each type already makes up the full overall symmetry of the total compound, and further all those types of components will have the same edge length. (This will brake down in 2D, as different polygons, with the same edge-length, always have different circumradii.) Just to provide examples:
- cid, seen as uniform compound of an ike with a vertex coincident gad
- gacid, seen as uniform compound of a sissid with a vertex coincident gike
- sicdatrid, seen as uniform compound of a sidtid with 5 vertex coincident cubes (or, equivalently, with a rhom)
- gicdatrid, seen as uniform compound of a gidtid with 5 vertex coincident cubes (or, equivalently, with a rhom)
- cadditradid, seen as uniform compound of a ditdid with 5 vertex coincident cubes (or, equivalently, with a rhom)
- badhidy, seen as uniform compound of a tigaghi with a vertex coincident quit sishi
Note that for such uniform compounds (with identified vertices) the demand on uniformity of individual components would not hold! Just consider the (mere) compound of 12 (scaliform) pentagonal pyramids, vertex-inscribed into an icosahedron (which itself will not belong to that compound). That compound thus even qualifies as isohedral. If it additionally is asked to be uniform, from the damand on vertex figure transitivity it becomes clear that vertices need to be identified. – Thus we then constructed a uniform compound (with identified vertices) from non-uniform components! (In fact a hollow and moreover Grünbaumian one, as triagles are completely coincident by 3 each.)
Thus, as long as vertex identification does not take place, uniform compounds need not only be isogonal (by definition), but come out to be isohedral as well. And likewise their components too will be uniform polytopes only.
A compound can be defined to be regular, just like a polytope, when it is transitive on all sub-dimensional elements and thereby does respect their respective incidences, i.e. if it is flag-transitive. An example of a regular compound within every dimension is the compound of 2 dually aranged simplices.
Moreover, there are further qualifiers of that type, introduced by Coxeter, vertex-regular and (dually) face-regular (or rather using a corresponding qualifier for the (n-1)-dimensional element). These are achieved, when the vertices (resp. the facet-planes) belong to a regular polytope. That is, when the encasing convex hull (resp. the common intersection kernel) would be a regular polytope. Or, again stated in an other way, vertex-regular compounds occure as facetings of regular polytopes, while face-regular compounds occure as stellations of regular polytopes.
For (isohedral) vertex-regular compounds and their duals Coxeter introduced the following notation:
a P [b Q] c Rwhere Q denotes the (regular) component polytopes (therefore, like P and R too, usually written as Schläfli symbol), b is the count of components. If vertex-regular, one has an encasing regular polytope P, the vertices of which will be used by a components each. Conversely, if face(t)-regular, there is a common intersection kernel which is a further regular polytope R, again possibly with its facet-planes being used by c components each. If vertex-regular but not face(t)-regular, the final part behind the closing square bracket will be omitted; conversely, if face(t)-regular but not vertex-regular the part before the opening one is omitted. (Examples will be given below, as far as applicable.
Obviously the full splendor of this notation applies when it is both, vertex- and facet-regular, which Coxeter himself then just calls just "regular". However this term here will be attributed as Coxeter-regular, as it clearly differs from the above mentioned regularity wrt. flag-transitivity!
Compounds need some extension to the "normal" incidence matrix description, which is due to their multiple components. The appropriately extended description can be found here, and will be used within the individual compound files linked below.
Some compounds show up an overall symmetry which is the same as that of the individual components too. Then those compounds can be described by a (stacked) Dynkin symbol. Further, compounds of 2 components occasionally might be described as both of the alternated facetings (i.e. compound of snubs). Then they can be described by an holosnub notation.
|
External links |
 3D 3D
 4D 4D


|
Blends
Closely related with the topics of (generall, i.e. not necessarily uniform) compounds is the topic of blends, a concept intoduced by Olshevsky. Likewise it might deal with multicovers of a single spot by more than one polytope (infact, here the number generally will be 2, asked by the dyadicity argument used below). For blends this furthermore asks for having at least one of its facets each completely coincident. The blend then will be set up by the reduction of the component polytopes by these coincident facets, re-adjoining these reductions dyadically at those coincident (open) ridges. Therefore, blends (of polytopes) are still true polytopes. Also there are 2 kinds of blends, internal and external, depending on having the polytopal centers on the same resp. on different sides of the facetal hyperplane. For instance, many of the Johnson solids are external blends of easier components. (The idea of blends sure can also be applied to compounds instead of mere polytopes.) For an explicit example of an internal blend consider the one built from 2 pentagonal prisms (pip), re-adjoined (internally) at a lacing square, having the componental axes arranged orthogonally (tupip). – The withdrawn original doubled up facets clearly still hold their shapes, just having no body any more, therefore those could be spoken of as pseudo facets.
Although because of dyadicity just 2 components blend at a single former facet, that initial blend itself might become subject to a further blending operation at a different facet in turn. For instance in the right picture a central sidtid got blended by 12 stiscues.
It further might occur that subsets of uniform polytopes of the same regiment form a group under the action of blending. Such a group then is called a cohort. For instance co, oho, and cho make up such a cohort of 3. (In fact, here either one can be produced as the blend of the other two.)
External
links
Fissary Polytopes, Complexified Polytopes, and Exotic Polytopes
The higher the dimension the count of possible polytopes increases exponentially. So some restrictions onto what should be considered a "true" polytope might be in place. Dyadicity, i.e. every edge has exactly 2 ends, and, dually, every ridge connects exactly 2 facets, is rather generally accepted. Compounds on the other side are excluded. But even then the realm of uniform polytopes becomes rather huge. Even for 4D the count of known uniform polychora runs way beyond 8000, Grünbaumian figures with completely coincident elements not even included. This situation was the reason for Johnson and Bowers to introduce the following attributes for in a stricter sense further on to be excluded "polychoroids".
One being the attribute fissary, kind as a midway between "true" polytopes and compounds, attributed to non-compound polytopes which have a compound vertex figure.
Dual to fissary polytopes would be figures using compounds for facets (i.e. d-1-faces), but still are not themselves compounds. Those are called complexified polytopes. – In fact this situation happens to occur quite often beyond 3D. Consider facets showing up both, the same symmetry and the same circumradius. Accordingly those would have to occur as facets on the same sub-symmetry axis of the polytope of consideration, and on that axis moreover they have to be placed at the same distance. I.e. those become co-realmic. In case they are not identic sub-polytopes and so would be completely coincident (then could be blended out, for instance), those will become compounds (not necessarily uniform ones, although their components are uniform, confer the semicompounds mentioned above). – Because those complexified polytopes need some multiwrap (more than one facet occupies the same direction) those clearly cannot occur for convex figures. In contrary they come rather close to Grünbaumian figures.
Then the exotic ones, attributed to polytopes with completely coinciding ridges (i.e. d-2-faces). Although those exotic polytopes are well-behaved dyadic abstract polytopes, their Grünbaumian realisation for some authors would give rise to "see" their coincidic elements being identified. This shows their closeness to non-dyadic "polytopes". Examples here are gidisdrid and cid in 3D (with coincident edges), or seedatepthi in 4D (with coincident pentagons).
External
links
fissary
exotic
---- 2D ----
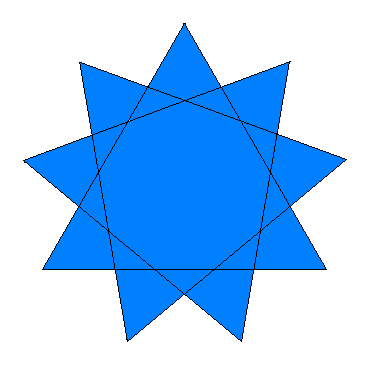
For uniform compounds in 2D we only have 2 possibilities. The first possibility occurs whenever any number k of n/d-gons (k and d free of common divisors) is arranged within an encasing (approprately scaled) kn-gon (vertex-regular); cf. the picture below, where k=n=3 and d=1). By self-duality of polygons one likewise could say, that those are derived as stellations of their common intersection, i.e. a smaller copy of that kn-gon (edge-regular). In general this is what Coxeter denotes as {kn}[k{n/d}]{kn}. Obviously these are the regular compounds of this dimensionality. By definition this type of compound clearly is a static one. Even though, it might occure (for k=2) as limiting case of the following, continuos case. – For the cases with k=2 also a closed Dynkin notation can be provided:
{2n}[2{n/d}]{2n} = β-n/d-β = xo-n/d-ox
The other possibility here contains a continuous parameter, but uses 2 components only. Those compounds are obtained by any specific uniform polygon, which is mirrored at any line which itself is not a mirror symmetry of that first component. Obviously, there is a continuous range of such choices for the angle to be taken between 2 such (neighbouring) mirrors. – Instead of 2 copies of some simple polygon as components, also 2 copies of some regular compound, i.e. one of the first kind, could be used alike. Then, for sure, the total number of components will become accordingly twice the component count of either of those 2 involved component compounds.
 |
 |
| regular polygonal compound {9}[3{3}]{9} within an encasing polygon (resp. around a polygon) | continuously deformable uniform compound of 2 polygons (or of 2 regular compounds) |
| Coxeter | Dynkin | Bowers | name |
{6}[2{3}]{6}
|
xo3ox | shig | stellated hexagon, star of David |
{8}[2{4}]{8}
|
xo4ox | soc | stellated octagon, star of Lakshmi |
{9}[3{3}]{9}
| fen | fissal eneagon | |
{10}[2{5}]{10}
|
xo5ox | sadeg | stellated decagon |
{10}[2{5/2}]{10}
|
xo5/2ox | sadag | stellated decagram |
{12}[2{6}]{12}
|
xo6ox | sedog | stellated dodecagon |
{12}[3{4}]{12}
| trisq | trisquare | |
{12}[4{3}]{12}
| tetri | tetratrigon |
Any 2D regular compound (in Coxeter's reading provided above) clearly is also regular in the sense of flag-transitivity. This sadly does not take over to the other dimensions, so.
---- 3D ----
The listing of uniform compounds of this dimension was done by Skilling in 1976. There are again those 2 possibilities. They all are given within the following pictures of Bowers. For the static ones the encasing polyhedron no longer needs to be uniform itself, its edges might have different sizes. And for the deformable ones, the number of components no longer is bound to be 2.
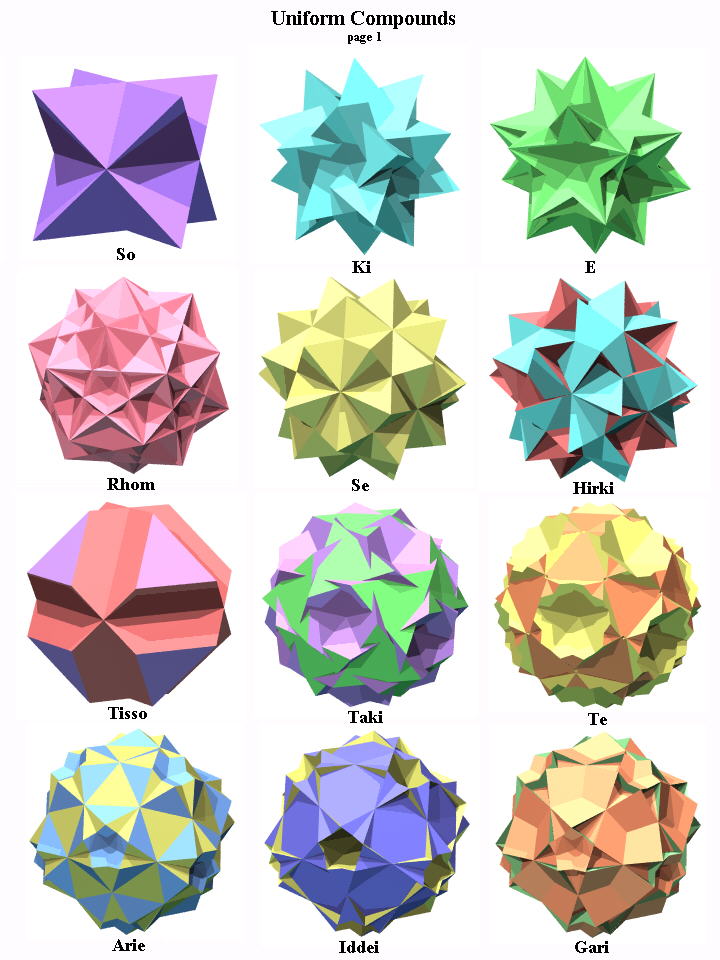
The set of "regular" 3D compounds is contained in so, ki, e, rhom, and se. As detailed below, the first three would be Coxeter-regular (both vertex- and face-regular), while rhom is only vertex-regular, and conversely its dual, se, is just face-regular. However only so is regular within the sense of flag-transitivity.
|
so (stella octangula) - S04 = self-dual compound of 2 tet within an encasing cube common intersection being an oct {4,3}[2{3,3}]{3,4} cf. bisimplex details and bidemicube details single flag-transitive 3D compound ki (chiro-icosahedron) - S05 = self-dual compound of 5 tet within an encasing doe common intersection being an ike {5,3}[5{3,3}]{3,5} e (icosiicosahedron) - S06 = self-dual compound of 10 tet within an encasing doe common intersection being an ike vertices coincide by pairs 2{5,3}[10{3,3}]2{3,5} rhom (rhombihedron) - S09 = compound of 5 cube edge-faceting of sidtid within an encasing doe common intersection being a rhote vertices coincide by pairs 2{5,3}[5{4,3}] se (small icosiicosahedron) - S17 = compound of 5 oct within an encasing id common intersection being an ike [5{3,4}]2{3,5} hirki (hemirhombichiro-icosahedron) - S18 = compound of 5 thah within an encasing id tisso (truncated stella octangula) - S54 = compound of 2 tut within an encasing q3o4x common intersection being an oct [2{;3;3}]{3,4} taki (truncated chiro-icosahedron) - S55 = compound of 5 tut common intersection being an ike [5{;3;3}]{3,5} te (truncated icosiicosahedron) - S56 = compound of 10 tut common intersection being an ike [10{;3;3}]2{3,5} arie (antirhombicosicosahedron) - S59 = compound of 5 co iddei (icosidisicosahedron) - S61 = compound of 5 oho gari (great antirhombicosahedron) - S60 = compound of 5 cho |
|
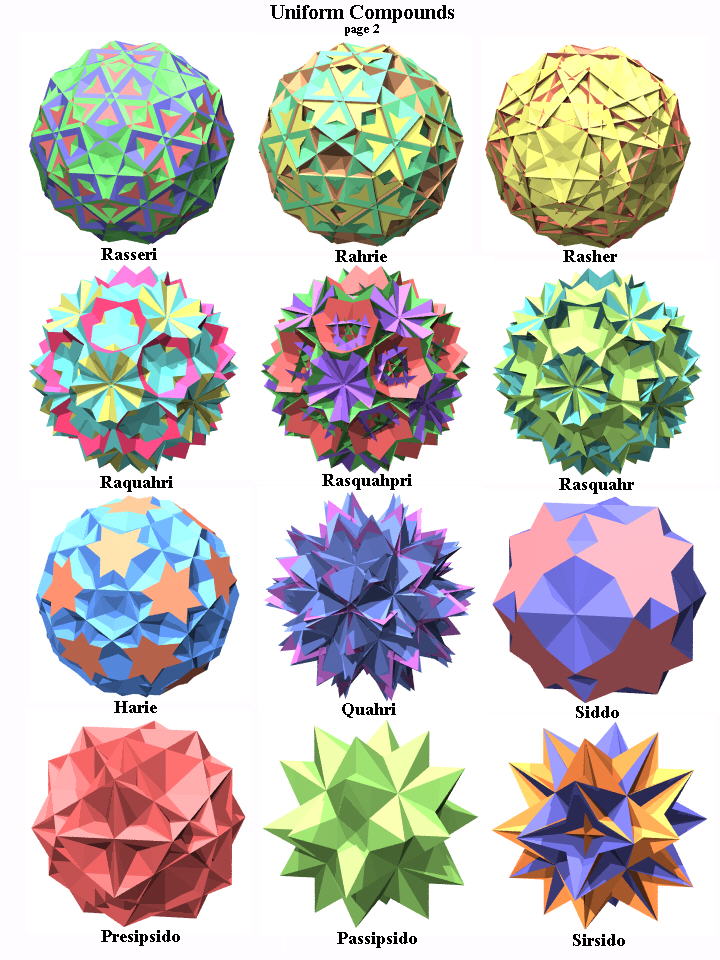
rasseri (rhombisnub rhombicosicoahedron) - S62 = compound of 5 sirco rahrie (rhombihyperhombicosicosahedron) - S64 = compound of 5 socco rasher (rhombisnub hyperhombihedron) - S63 = compound of 5 sroh raquahri (rhombiquasihyperhombicosicosahedron) - S65 = compound of 5 gocco rosaqri (rhombisnub quasirhombicosicosahedron) - S67 (old: rasquahpri = rhombisnub quasihyperpseudorhombicosicosahedron) = compound of 5 querco rasquahr (rhombisnub quasihyperrhombihedron) - S66 = compound of 5 groh tar (truncated rhombihedron) - S57 (old: harie = hyperhombicosicosahedron) = compound of 5 tic quitar (quasitruncated rhombihedron) - S58 (old: quahri = quasihyperhombicosicosahedron) = compound of 5 quith siddo (snub disoctahedron) - S46 = compound of 2 ike within an encasing x3f4o presipsido (pseudoretrosnub pentagonal snub (pseudo)disoctahedron) - S48 = compound of 2 gad within an encasing x3f4o passipsido (pseudosnub pentagrammatic snub (pseudo)disoctahedron) - S50 = compound of 2 sissid within an encasing x3f4o sirsido (small retrosnub disoctahedron) - S52 = compound of 2 gike within an encasing x3f4o |

|
sne (snub icosiicosahedron) - S47 = compound of 5 ike presipsi (pseudoretrosnub pentagonal snub (pseudo)icosiicosahedron) - S49 = compound of 5 gad passipsi (pseudosnub pentagrammattic snub (pseudo)icosiicosahedron) - S51 = compound of 5 sissid sirsei (small retrosnub icosiicosahedron) - S53 = compound of 5 gike rah (rhombihexahedron) - S08 = compound of 3 cube within an encasing x3w4o common intersection being a sirco-stellation (the mere edge-truncation of the cube) risdoh (rhombisnub dishexahedron) - S07 = compound of 6 cube : rotational freedom ro ((chiral) rhombioctahedron) - S30 = compound of 4 trip dro (dirhombioctahedron) - S31 = compound of 8 trip gassic ((chiral) great snub cube) - S42 = compound of 3 squap gidsac (great disnub cube) - S43 = compound of 6 squap griso (great rhombisnub octahedron) - S38 = compound of 4 hip sno (snub octahedron) - S12 = compound of 4 oct within an encasing o3x4q |

|
daso (old: doso; disnub octahedron) - S11 = compound of 8 oct : rotational freedom, see idso, hidso, odso idso (inner disnub octahedron) special range of daso hidso (hexagrammattic disnub octahedron) special case of daso (lateral {3} become (rotated) 2{3}-compounds) odso (outer disnub octahedron) special range of daso dissit (disnub tetrahedron) - S10 = compound of 4 oct : rotational freedom, see idsit, hidsit, odsit idsit (inner disnub tetrahedron) special range of dissit hidsit (hexagrammattic disnub tetrahedron) special case of dissit (lateral {3} become (rotated) 2{3}-compounds) odsit (outer disnub tetrahedron) special range of dissit sis (small snubihedron) - S01 = compound of 6 tet : rotational freedom, see snu snu (snubihedron) - S03 special case of sis (2-fold axes become 4-fold) within an encasing x3w4o dis (disnubihedron) - S02 = compound of 12 tet : rotational freedom rassid (rhombisnub dodecahedron) - S40 = compound of 6 dip grassid (great rhombisnub dodecahedron) - S41 = compound of 6 stiddip rosi (rhombisnub icosahedron) - S39 = compound of 10 hip |

|
kred (old: red, chiro-rhombidodecahedron) - S34 = compound of 6 pip within an encasing f3x5o dird (dirhimbidodecahedron) - S35 = compound of 12 pip within an encasing f3x5o vertices coincide by pairs gikrid (great chirorhombidodecahedron) - S36 = compound of 6 stip within an encasing srid {;3,5;}[6{;5/2,2;}] giddird (great dirhombidodecahedron) - S37 = compound of 12 stip within an encasing srid vertices coincide by pairs 2{;3,5;}[12{;5/2,2;}] kri (chirorhombicosahedron) - S32 = compound of 10 trip within an encasing x3f5o dri (dirhombicosahedron) - S33 = compound of 20 trip within an encasing x3f5o vertices coincide by pairs gassid (great snub dodecahedron) - S27 = compound of 6 pap gadsid (great disnub dodecahedron) - S26 = compound of 12 pap : rotational freedom gissed (great invertisnub dodecahedron) - S29 = compound of 6 starp gidasid (great invertidisnub dodecahedron) - S28 = compound of 12 starp : rotational freedom sassid ((chiral) small snub dodecahedron) - S44 = compound of 6 stap sadsid (small disnub dodecahedron) - S45 = compound of 12 stap |

|
si (snub icosahedron) - S16 = compound of 10 oct addasi (altered disnub icosahedron) - S13 : rotational freedom, see oddasi, dasi, iddasi, giddasi oddasi (outer disnub icosahedron) = compound of 20 oct special range of addasi dasi (disnub icosahedron) - S14 = compound of 20 oct special case of addasi edge-faceting of gidrid vertices coincide by pairs iddasi (inner disnub icosahedron) = compound of 20 oct special range of addasi giddasi (great disnub icosahedron) = compound of 20 oct special range of addasi gissi (great snub icosahedron) - S15 = compound of 10 oct sapisseri ((chiral) snub pseudosnub rhombicosahedron) - S19 = compound of 20 thah edge-faceting of gidrid vertices coincide by pairs disco (disnub cuboctahedron) - S68 = compound of 2 snic dissid (disnub icosidodecahedron) - S69 = compound of 2 snid disdid (disnub dodecadodecahedron) - S73 = compound of 2 siddid giddasid (great disnub icosidodecahedron) - S70 = compound of 2 gosid idisdid (invertidisnub dodecadodecahedron) - S74 = compound of 2 isdid |

|
gidsid (great invertidisnub icosidodecahedron) - S71 = compound of 2 gisid desided (disnub icosidodecadodecahedron) - S75 = compound of 2 sided gidrissid (great diretrosnub icosidodecahedron) - S72 = compound of 2 girsid |
The similar compound of 2 enantiomorphic snubs (as given on Bowers last 2 figure plates above) for the case of gisdid there (and usually) is excluded from the listings for the same reason as Grünbaumian figures were excluded from normal polytopes, i.e. because of the complete coincidence of facial elements (all pentagrams in that case). That one would be a further edge faceting of gidrid. – Likewise the compound of 3 (orthogonal) op is here not contained. The corresponding blend is nothing but sroh. Conjungately the compound of 3 (orthogonal) stop, blending out the coincident squares, would result in groh. In the latter 2 cases 1/2 of the squares each would have aligned in complete coincidence.
None the same, what really is missing in these above pictures, are all the axial cases, based on the 2D compounds
cf.
![]()
| Axials | n/d-prisms | n/d-antiprisms d odd |
n/d-antiprisms d even |
| regular compound bases |
 S21 (esp. 2 cubes) |
 S23 (esp. 2 oct) |
 S25 |
| rotational freedom |
 S20 |
 S22 |
 S24 |
Interesting compounds with higher symmetry than that of its componenents so can even be obtained from non-uniform components. Sure, the result then will not become uniform either. As an according example might serve the compound of 5 rad. Obviously it shows up 2 different vertex types.
©, cf.

---- 2D (euclidean tilings) ----
At the end of 2020 Binnendyk came up with some compounds of flat euclidean tilings as well. He found them by generalizing common edge figures of spherical space polyhedra or according compounds to higher numbers, then becoming flat. (© : The below provided hand-drawn sketches all are his and are reproduced from that cited page.)
|
vertex configuration |
P = 4 (spherical) |
P = 5 (spherical) |
P = 6 (euclidean compound) |
|---|---|---|---|
| (3, 6, P/2, 6) |
tisso = 2-tut-compound |
siid |
2-that-compound |
| (P/2, P, P/2, P) |
compound of 3 square-dihedra (as diagonals of oct) together with inserted Grünbaumian edge multicovers |
did |
3-that-compound |
| (3, 2P/3, P, 2P/3) |
gocco |
gidditdid |
4-rothat-compound |
| (4, P/2, 4, P) |
rah = 3-cube-compound |
raded |
3-rothat-compound |
| (6, 2P, 2P/3) |
cotco |
idtid |
4-grothat-compound |
| (2P/3, 2P/3, (10-P)/(6-P)) |
quith |
quit sissid |
(denser) ∞-azip-compound |
| (4, 2P/3, 2(10-P)/(6-P)) |
quitco |
quitdid |
(sparser) ∞-azip-compound |
Further there are singular cases too, which cannot be given within such a dynamic extrapolation:
 © ©
3-trat-compound |

ghat - "great hexagonal tiling", (old: fexat - "fissal hexagonal tiling"), 3-hexat-compound |
---- 2D (hyperbolic tilings) ----
Just to mention that even within hyperbolic space there exist compounds too. For example the following compound of two x4o8o (osquat), which also could be described as {8,4}[2{4,8}].
©
---- 4D ----
Because the set of uniform polychora is not proven to be enlisted completely so far, even less is known about uniform compounds of 4-dimensional space. And even of those which are known so far only some are listed here ...
| sorted alphabetically by components | |
|---|---|
teppix = compound of 60 deca in dattady regiment, in hi army vertices coincide by 3 3{5,3,3}[60{3;3;3}] ??? = compound of 6 dedip in hi army {5,3,3}[6{;5,2;5}] sadtap = compound of 36 dedip in sidtaxhi regiment, in hi army vertices coincide by 6, edges coincide by pairs 6{5,3,3}[36{;5,2;5}] fasp = compound of 12 distadedip ("fissal swirlprism") xehix = compound of 60 duhd ("hexacosahemihexacosachoron") in sishi regiment katdex = compound of 2 ex ("chirotetrahedral dishexacosachoron") spohi = compound of 5 ex in hi army {5,3,3}[5{3,3,5}] kepisna = compound of 6 ex in rox army {3;3,5}[6{3,3,5}] sody = compound of 10 ex in hi army vertices coincide by pairs 2{5,3,3}[10{3,3,5}] kidisna = compound of 10 ex in rahi army {3,3;5}[10{3,3,5}] pedisna = compound of 12 ex in rox army vertices coincide by pairs 2{3;3,5}[12{3,3,5}] ditusna = compound of 20 ex in rahi army vertices coincide by pairs 2{3,3;5}[20{3,3,5}] firmax = compound of 25 firp ("facetorectified medial hexacosachoron") in romex regiment frahdet = compound of 2 firt ("facetobirectified haddet") firdac = compound of 75 firt ("faceted rectified dodecahedronary cubichoron") in rahi army fispohi (old: fasophi) = compound of 5 fix ("faceted small pentishecatonicosachoron") in hi army {5,3,3}[5{3,5,5/2}] kihp = compound of 6 fix ("chiroicosahedral hyperprismatochoron") in rox army fassody = compound of 10 fix ("faceted small deckishecatonicosachoron" /"faceted snub decahecatonicosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{3,5,5/2}]2{3,3,5} dihp = compound of 12 fix ("disicosahedral hyperprismatochoron") in rox army vertices coincide by pairs firdox (old: fapchix) = compound of 25 frico ("facetorectified dodecahedronary-hexacosachoron", old: "facetopentacubic hecatonicosahexacosachoron") in rissidtixhi regiment, in rahi army gogakix = compound of 5 gaghi ("great grand chirohexacosachoron") in hi army {5,3,3}[5{5,5/2,3}] gigakidhep = compound of 6 gaghi ("great grand chirododecahedral hyperprismatochoron") in rox army gigadex = compound of 10 gaghi ("great grand dishexacosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{5,5/2,3}]2{3,3,5} gigadidhep = compound of 12 gaghi ("great grand disdodecahedral hyperprismatochoron") in rox army vertices coincide by pairs gakix = compound of 5 gahi ("grand chirohexacosachoron") in hi army {5,3,3}[5{5,3,5/2}] gakidhep = compound of 6 gahi ("grand chirododecahedral hyperprismatochoron") in rox army gadex = compound of 10 gahi ("grand dishexacosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{5,3,5/2}]2{3,3,5} gadidhep = compound of 12 gahi ("grand disdodecahedral hyperprismatochoron") in rox army vertices coincide by pairs ??? = compound of 2 gap ??? = compound of 36 gap in some semiuniform xhi army gaqragi = compound of 3 gaqrit ("great quasirhombated gico") gaqrigastic = compound of 6 gaqrit ("great quasirhombated gistic") gaskix = compound of 5 gashi ("grand stellated chirohexacosachoron") in hi army {5,3,3}[5{5/2,5,5/2}] gaskadhep = compound of 6 gashi ("grand stellated chirododecahedral hyperprismatochoron") in rox army gasdex = compound of 10 gashi ("grand stellated dishexacosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{5/2,5,5/2}]2{3,3,5} gasdadhep = compound of 12 gashi ("grand stellated disdodecahedral hyperprismatochoron") in rox army vertices coincide by pairs gasspohi = compound of 5 gax ("grand snub pentishecatonicosachoron") in hi army {5,3,3}[5{3,3,5/2}] gassody = compound of 10 gax ("grand snub deckishecatonicosachoron") in hi army vertices coincide by pairs 2{5,3,3}[10{3,3,5/2}] gakpisna = compound of 6 gax ("grand chiropental snubbachoron") in rox army gapdisna = compound of 12 gax ("grand pental disnubbachoron") in rox army vertices coincide by pairs afpox = compound of 60 gippid in affixthi regiment vertices coincide by pairs gepdi = compound of 3 girdo ("great prismatodisicositetrachoron") in giddic regiment, in spic army giskix = compound of 5 gishi ("great stellated chirohexacosachoron") in hi army {5,3,3}[5{5/2,3,5}] giskadhep = compound of 6 gishi ("great stellated chirododecahedral hyperprismatochoron") in rox army gisdex = compound of 10 gishi ("great stellated dishexacosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{5/2,3,5}]2{3,3,5} gisdadhep = compound of 12 gishi ("great stellated disdodecahedral hyperprismatochoron") in rox army vertices coincide by pairs gisdidhop = compound of 20 gishi ("great stellated disicosahedronary dishyperprismatochoron") kiparp = compound of 6 gisp ("chiropentagrammatic retroprismatochoron") in rox army diparp = compound of 12 gisp ("dipentagrammatic retroprismatochoron") in rox army vertices coincide by pairs gifspohi = compound of 5 gofix ("great faceted small pentishecatonicosachoron") in hi army {5,3,3}[5{3,5/2,5}] gikihp = compound of 6 gofix ("great chiroicosahedral hyperprismatochoron") in rox army gifsody = compound of 10 gofix ("great faceted small deckishecatonicosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{3,5/2,5}]2{3,3,5} gidihp = compound of 12 gofix ("great disicosahedral hyperprismatochoron") in rox army vertices coincide by pairs gogiskix = compound of 5 gogishi ("great grand stellated chirohexacosachoron") gogaskadhep = compound of 6 gogishi ("great grand stellated chirododecahedral hyperprismatochoron") in f3o3x5o army gogisdex = compound of 10 gogishi ("great grand stellated dishexacosachoron") gogasdadhep = compound of 12 gogishi ("great grand stellated disdodecahedral hyperprismatochoron") in f3o3x5o army gokix = compound of 5 gohi ("great chirohexacosachoron") in hi army {5,3,3}[5{5,5/2,5}] gikadhep = compound of 6 gohi ("great chirododecahedral hyperprismatochoron") in rox army godex = compound of 10 gohi ("great dishexacosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{5,5/2,5}]2{3,3,5} gedadhep = compound of 12 gohi ("great disdodecahedral hyperprismatochoron") in rox army vertices coincide by pairs gedidhop = compound of 20 gohi ("great disicosahedronary dishyperprismatochoron") garstic = compound of 2 grico ("great rhombated stellated tetracontoctachoron") gristed = compound of 2 (inverted) grip ("great rhombated stellated decachoron") in sobcated army gargi = compound of 3 grit ("great rhombated gico") gargastic = compound of 6 grit ("great rhombated gistic") ditsop = compound of 12 gudap ("ditrigonary swirlprism") in sishi regiment, in ex army haddet = compound of 2 hex in tes army common intersection being a ico {4,3,3}[2{3,3,4}]{3,4,3} cf. bidemicube details stico = compound of 3 hex in ico army common intersection being a ico {3,4,3}[3{3,3,4}]2{3,4,3} flag-transitive sistic = compound of 6 hex in stoc army = compound of 2 stico flag-transitive kixfig = compound of 8 hex ("chirocrossifissal 64-choron") in odip army (because hex is just the 8-3-stepprism within odip) chiral kotapna = compound of 12 hex ("chirotrigonal antiprismary enenahexachoron") in rico army {3;4,3}[12{3,3,4}] ??? = compound of 15 hex in ex army common intersection being a hi {3,3,5}[15{3,3,4}]2{5,3,3} cefdig = compound of 16 hex ("crossifissal dis64-choron") in odip army (because hex is just the 8-3-stepprism within odip) ??? = compound of 18 hex (happens to be uniform indeed, even so its verf is the non-uniform compound of 3 oct) (dual of 18 tes compound) botapna = compound of 24 hex ("bitrigonal antiprismary enenahexachoron") in rico army vertices coincide by pairs 2{3;4,3}[24{3,3,4}] hesatdi = compound of 50 hex (obtained as alternation of each tes in the 25 tes compound) stidox = compound of 75 hex in ex army common intersection being a hi vertices coincide by 5 5{3,3,5}[75{3,3,4}]10{5,3,3} = compound of 25 stico ??? = compound of 75 hex in hi army common intersection being an ex {5,3,3}[75{3,3,4}]2{3,3,5}(var) (occuring as a different substructure of the 675 hex-comp.) ??? = compound of 300 hex in hi army common intersection being an ex vertices coincide by 4 4{5,3,3}[300{3,3,4}]8{3,3,5} ??? = compound of 600 hex in hi army common intersection being an ex vertices coincide by 8 8{5,3,3}[600{3,3,4}]16{3,3,5} ??? = compound of 675 hex in hi army common intersection being an ex vertices coincide by 9 9{5,3,3}[675{3,3,4}]18{3,3,5} ??? = compound of 2 hi common intersection being an ex [5{5,3,3}]{3,3,5} kadhep = compound of 6 hi ("chirododecahedral hyperprismatochoron") in f3o3x5o army ??? = compound of 10 hi common intersection being an ex [10{5,3,3}]2{3,3,5} dadhep = compound of 12 hi ("disdodecahedral hyperprismatochoron") in f3o3x5o army affip = compound of 16 hiddip in afdec regiment, in cont army vertices coincide by pairs 2{3,4,3}[16{;6,2;6}] datap = compound of 100 hiddip in dattady regiment, in hi army vertices coincide by 6, edges coincide by pairs 6{5,3,3}[100{;6,2;6}] dethnah = compound of 2 hinnit dynaphi = compound of 75 hinnit ("decahecatonicosintercepted pentahecatonicosachoron") in rahi army stoc = compound of 2 (dual) ico in bicont (cont-dual) army common intersection being a cont [2{3,4,3}]{3;4;3} flag-transitive kitapna = compound of 4 ico in rico army {3;4,3}[4{3,4,3}] chi = chiral compound of 5 ico in a chiral sub-sishi regiment, in ex army (not all edges being used) common intersection being a hi {3,3,5}[5{3,4,3}]{5,3,3} kitefa = compound of 6 ico in spic army {;3,4,3;}[6{3,4,3}] bitapna = compound of 8 ico in rico army vertices coincide by 2 2{3;4,3}[8{3,4,3}] bitefa = compound of 12 ico in spic army 2{;3,4,3;}[12{3,4,3}] ??? = compound of 20 ico in a chiral sub-sishi regiment, in ex army = dox - chi dox = compound of 25 ico in sishi regiment, in ex army common intersection being an ex vertices coincide by 5 5{3,3,5}[25{3,4,3}]{3,3,5} ??? = compound of 25 ico in hi army {5,3,3}[25{3,4,3}]5{5,3,3} (using the duals of the former) ??? = compound of 25 ico in hi army {5,3,3}[25{3,4,3}](var) (occuring as a substructure of the 5 ex-comp.) ??? = compound of 25 ico common intersection being an ex [25{3,4,3}]{5,3,3}(var) (using the duals of the former) ??? = compound of 100 ico in hi army vertices coincide by 4 4{5,3,3}[100{3,4,3}]5{5,3,3} ??? = compound of 200 ico in hi army vertices coincide by 8 8{5,3,3}[200{3,4,3}]5{5,3,3} ??? = compound of 225 ico in hi army vertices coincide by 9 9{5,3,3}[225{3,4,3}] ??? = dual compound of 225 ico common intersection being an ex 9 octs each are co-realmic (building a compound on their own) [225{3,4,3}]9{3,3,5} sadixhix = compound of 25 ihi in sishi regiment, in ex army vertices coincide by 5 (different orientation of ihis than for gadixhix) gadixhix = compound of 25 ihi in sishi regiment, in ex army vertices coincide by 5 (different orientation of ihis than for sadixhix) xix = compound of 25 ini ("hexacosahexacosachoron") in rissidtixhi regiment, in rahi army |
??? = [Grünbaumian] compound of 3 odip in srit regiment vertices coincide by 2 blending out pairs of coincident squares → garpit ??? = [Grünbaumian] compound of 9 odip in spic regiment vertices coincide by 4 blending out pairs of coincident squares → sirc kra = compound of 10 ope ("chirorhombicosahedral alterprism") in tipe-variant army alterprism based on kri ostople = compound of 2 ostodip ({8}x{8/3} + {8/3}x{8}) pedeple = compound of 2 padedip ({5}x{10} + {10}x{5}) gakskos = compound of 5 paphacki ("great chirosnub chillioctacosachoron") ??? = compound of 10 paphacki in hi army in 10-sishi-compound regiment ??? = compound of 10 paphicki in hi army in 10-sishi-compound regiment sadegdip = compound of 4 pedip ("stellated-decagonal duoprism") in dedip army {10}x{10}[4{5}x{5}] tripendip = compound of 9 pedip ("tripentagonal duoprism") {15}x{15}[9{5}x{5}] ??? = compound of 16 pedip {20}x{20}[16{5}x{5}] ??? = compound of 144 pedip in ex army vertices coincide by 6 6{3,3,5}[144{5}x{5}] sted = compound of 2 (dual) pen in bideca (deca-dual) army common intersection being a deca [2{3,3,3}]{3;3;3} cf. bisimplex details flag-transitive gysfi = compound of 4 pen ("gyrosimplexifissal icosachoron") in pedip army chiral kisfis = compound of 5 pen ("chirosimplexifissal icosipentachoron") in pedip army (because pen is just the 5-2-stepprism within pedip) chiral sifdis = compound of 10 pen ("simplexifissal disicosipentachoron") in pedip army ??? = compound of 20 pen in hi army {5,3,3}[20{3,3,3}] mix = compound of 120 pen in mix regiment, in hi army common intersection being an ex {5,3,3}[120{3,3,3}]{3,3,5} flag-transitive ??? = compound of 120 pen in hi army common intersection being an ex {5,3,3}[120{3,3,3}]{3,3,5}(var) (occuring as a different substructure of the following) ??? = compound of 720 pen in hi army common intersection being an ex 6{5,3,3}[720{3,3,3}]6{3,3,5} pinnix = compound of 120 pinnip ("prismatointercepted hexacosachoron") in romex regiment pestideple = compound of 2 pistadedip ({5}x{10/3} + {10/3}x{5}) gecupady = compound of 5 prarsi ("great cupoliprismatodishecatonicosachoron") blend of 5 gecupadies would result in gad phiddix parstic = compound of 2 prico ("prismatorhombated stellated tetracontoctachoron") pirsted = compound of 2 (inverted) prip ("prismatorhombated stellated decachoron") scupady = compound of 5 prissi ("small cupoliprismatodishecatonicosachoron") blend of 5 scupadies would result in sad phiddix pregi = compound of 3 prit ("prismatorhombated gico") pirgastic = compound of 6 prit ("prismatorhombated gistic") badhidy = non-isohedral compound of quit sishi and tigaghi in sabbadipady regiment, in sidpixhi quitagi = compound of 3 quitit ("quasitruncated gico") quit gistic = compound of 6 quitit ("quasitruncated gistic") quetdac = compound of 75 quitit ("quasitruncated dodecahedronary cubichoron") in thi-variant army risted = compound of 2 (inverted) rap ("rectified stellated decachoron") in bamid army romex = compound of 120 rap ("rectified medial hexacosachoron") in romex regiment, in rahi army misaxphi = compound of 25 rappisdi ("medial snub hexacosapentishecatonicosachoron") gisaxphi = compound of 25 rasdi ("great snub hexacosapentishecatonicosachoron") rastic = compound of 2 rico ("rectified stellated tetracontoctachoron") rectified stoc roktefa = compound of 6 rico ("rectified chirotegmic hecatontetraconttetrachoron") rectified kitefa robtefa = compound of 12 rico ("rectified bitegmic hecatontetraconttetrachoron") rectified bitefa redox (old: pichix) = compound of 25 rico ("rectified dodecahedronary-hexacosachoron", old: "pentacubic hecatonicosahexacosachoron") in rissidtixhi regiment, in rahi army 2{3,3;5}[25{3;4,3}] brahdet = compound of 2 rit ("birectified haddet") ragi = compound of 3 rit ("rectified gico") in rico army {3;4,3}[3{3,3;4}] ragistic = compound of 6 rit ("rectified gistic") redac = compound of 75 rit ("rectified dac") in rahi army rekatdex = compound of 2 rox ("rectified chirotetrahedral dishexacosachoron") raspohi = compound of 5 rox ("rectified small pentishecatonicosachoron") ??? = compound of 10 rox (however has coincident elements, its blend is: sadsadox) disti = compound of 2 sadi ("disnub tetrakisicositetrachoron") (where ikes in turn compound into siddoes, and 24 tets compound into soes) ??? = compound of 2 sadi (where ikes are like the facets of cont) sisaxphi = compound of 25 sadi ("small snub hexacosapentishecatonicosachoron") in sidpixhi army spagi = compound of 3 sidpith ("small prismated gico") spagastic = compound of 6 sidpith ("small prismated gistic") sepdi = compound of 3 sirdo ("small prismatodisicositetrachoron") in spic regiment and army siskix = compound of 5 sishi ("small stellated chirohexacosachoron") in hi army {5,3,3}[5{5/2,5,3}] skadhep = compound of 6 sishi ("stellated chirododecahedral hyperprismatochoron") in rox army sisdex = compound of 10 sishi ("small stellated dishexacosachoron") in hi army common intersection being a ex vertices coincide by pairs 2{5,3,3}[10{5/2,5,3}]2{3,3,5} sidadhep = compound of 12 sishi ("stellated disdodecahedral hyperprismatochoron") in rox army vertices coincide by pairs kepap = compound of 6 sisp ("chiropentagonal antiprismatochoron") in rox army dipap = compound of 12 sisp ("dipentagonal antiprismatochoron") in rox army vertices coincide by pairs sistople = compound of 2 sistodip in gittith regiment, in tat army ({4}x{8/3} + {8/3}x{4}) sople = compound of 2 sodip in sidpith regiment and army ({4}x{8} + {8}x{4}) ??? = compound of 5 spic ("small prismated chirohecatonicosachoron") dopix = compound of 60 spid in sishi regiment, in ex army vertices coincide by 10, edges coincide by 3 10{3,3,5}[60{;3,3,3;}] ??? = compound of 12 spidrox in o3f3x5o army ??? = compound of 24 spidrox in gidpixhi army serstic = compound of 2 srico ("small rhombated stoc") sristed = compound of 2 (inverted) srip ("small rhombated stellated decachoron") ??? = compound of 3 srit in spic regiment vertices coincide by 2 2{;3,4,3;}[3{3;3,4;}] gadtap = compound of 36 stadidip in gadtaxady regiment, in hi army vertices coincide by 6, edges coincide by pairs 6{5,3,3}[36{10/3}x{10/3}] stardeple = compound of 2 stardedip ({5/2}x{10} + {10}x{5/2}) ??? = compound of 144 stardedip in sidpixhi army starpeple = compound of 2 starpedip ({5}x{5/2} + {5/2}x{5}) starpepla = compound of 2 starpedip ({5}x{5/2} + inv{5/2}x inv{5}) ??? = compound of 4 starpedip in gap army spidy = compound of 24 starpedip in sishi regiment, in ex army vertices coincide by 5 5{3,3,5}[24{5}x{5/2}] ??? = compound of 144 stardip in ex army vertices coincide by 6 6{3,3,5}[144{5/2}x{5/2}] starstideple = compound of 2 stastidedip ({5/2}x{10/3} + {10/3}x{5/2}) ??? = [Grünbaumian] compound of 3 stodip in wavitoth regiment vertices coincide by 2 blending out pairs of coincident squares → gaqript ??? = [Grünbaumian] compound of 9 stodip in giddic regiment vertices coincide by 4 blending out pairs of coincident squares → girc bitagi = compound of 3 tah ("bitruncated gico") butgastic = compound of 6 tah ("bitruncated gistic") ??? = compound of 75 tah in hixady army tagi = compound of 3 tat ("truncated gico") tagistic = compound of 6 tat ("truncated gistic") tedac = compound of 75 tat ("truncated dodecahedronary cubichoron") in sidpixhi-variant army ??? = compound of 2 tes common intersection being a hex [2{4,3,3}]{3,3,4} gico = compound of 3 tes in ico regiment common intersection being an ico vertices coincide by pairs 2{3,4,3}[3{4,3,3}]{3,4,3} flag-transitive socdip = compound of 4 tes in odip army {8}x{8}[4{4,3,3}] gistic = compound of 6 tes in stoc army = compound of 2 gico flag-transitive trisqdip = compound of 9 tes in twaddip army {12}x{12}[9{4,3,3}] kitcana = compound of 12 tes ("chirotrigonal crystallic enenahexachoron") in rico army vertices coincide by pairs 2{3;4,3}[12{4,3,3}] cac = compound of 15 tes ("chirocubichoron") in a chiral sub-sishi regiment, in ex army (not all edges being used) 2{3,3,5}[15{4,3,3}]{5,3,3} tesqdip = compound of 16 tes ("tetrasquare duoprism") {16}x{16}[16{4,3,3}] ??? = compound of 18 tes in cont army (dual of 18 hex compound) bitcana = compound of 24 tes ("bitrigonal crystallic enenahexachoron") in rico army vertices coincide by 4 4{3;4,3}[24{4,3,3}] ??? = compound of 25 tes {20}x{20}[25{4,3,3}] ??? = compound of 36 tes in spic army ??? = compound of 36 tes {24}x{24}[36{4,3,3}] ??? = compound of 48 tes in gidpith army 2{;3;3;4;}[48{4,3,3}] ??? = compound of 49 tes {28}x{28}[49{4,3,3}] ??? = compound of 64 tes {32}x{32}[64{4,3,3}] dac = compound of 75 tes ("dodecahedronary cubichoron") in sishi regiment, in ex army vertices coincide by 10 2{3,3,5}[75{4,3,3}] ??? = compound of 75 hex in hi army common intersection being a ex 2{5,3,3}[75{4,3,3}]{3,3,5}(var) (occuring as dual of the var. 75 hex-comp.) ??? = compound of 81 tes {36}x{36}[81{4,3,3}] ??? = compound of 100 tes {40}x{40}[100{4,3,3}] ??? = compound of 300 tes in hi army common intersection being a ex vertices coincide by 8 8{5,3,3}[300{4,3,3}]4{3,3,5} ??? = compound of 600 tes in hi army common intersection being a ex vertices coincide by 16 16{5,3,3}[600{4,3,3}]8{3,3,5} ??? = compound of 675 tes in hi army common intersection being a ex vertices coincide by 18 18{5,3,3}[675{4,3,3}]9{3,3,5} tikatdex = compound of 2 tex ("truncated chirotetrahedral dishexacosachoron") tispohi = compound of 5 tex ("truncated small pentishecatonicosachoron") tissody = compound of 10 tex ("truncated small deckishecatonicosachoron") thiple = compound of 2 thiddip ({3}x{6} + {6}x{3}) dathah = compound of 2 tho ("distesseractihemihexadecachoron") in tes army (different orientation of thoes than for chehah) chehah = compound of 2 tho ("chirohexadecahemihexadecachoron") in tes army (different orientation of thoes than for dathah) tastic = compound of 2 tico truncated stoc tiktefa = compound of 6 tico ("truncated chirotegmic hecatontetraconttetrachoron") truncated kitefa tibtefa = compound of 12 tico ("truncated bitegmic hecatontetraconttetrachoron") truncated bitefa tidox = compound of 25 tico in tissidtixhi regiment vertices coincide by pairs tisted = compound of 2 tip truncated sted tomex = compound of 120 tip truncated mix shigdip = compound of 4 triddip ("stellated-hexagonal duoprism") in hiddip army {6}x{6}[4{3}x{3}] fendip = compound of 9 triddip ("fissary-enneagonal duoprism") in edip army {9}x{9}[9{3}x{3}] tetridip = compound of 16 triddip ("tetratrigon duoprism") {12}x{12}[16{3}x{3}] pentridip = compound of 25 triddip ("pentatrigon duoprism") {15}x{15}[25{3}x{3}] ??? = compound of 36 triddip {18}x{18}[36{3}x{3}] ... |
Here as well compounds with completely coincident facets shall be excluded. For according examples we eg. could mention the compound of 10 roxes, where 1/5 of the octs would coincide completely, so that the according blend results in nothing but sadsadox. – By conjugacy in the compound of 10 raggixes according octs again would coincide completely, thence blending into gadsadox.
---- 5D ----
Only few is known here so far ...
| sorted alphabetically by components | |
|---|---|
todin = compound of 2 (alternate) hin in pent army common intersection being a nit o3o3o3o4x [2 o3o3o3o4s] o3o3x3o4o cf. bidemicube details sikap = chiral compound of 3 hin ("stellated icositetrachoric chiroantiprism") stade = compound of 2 (dual) hix in bidot army common intersection being a dot [2{;3,3,3,3}]{3,3;3,3} cf. bisimplex details only 5D flag-transitive taxt = compound of 6 hix ("triaxis trigonism") vertex figure of tree vertices coincide by 2 ??? = compound of 30 hix in sarx army |
bic = compound of 10 squoct in rat regiment vertices coincide by 6 6{3;3,3,4}[10{;4,2;3,4}] tistade = compound of 2 (mutually inv.) tix ("truncated stellated dodecateron") ??? = compound of 40 tratrip in card army ... |
Compounds with completely coincident facets shall be excluded again. For according examples we eg. could mention the compound of 6 hins, where 1/5 of the hexes would coincide completely, so that the according blend results in nothing but hosiap.
---- 6D ----
Only few is known here so far ...
| sorted alphabetically by components | |
|---|---|
soctip = compound of 8 ax ("stellated-octagonal triprism") {8}x{8}x{8}[8{4,3,3,3,3}] tree = compound of 15 ax ("trishexacontapeton") in brag regiment vertices coincide by 6 edges coincide by 2 in fact its 180 pent facets form 60 gicope trisqtip = compound of 27 ax ("trisquare triprism") {12}x{12}x{12}[27{4,3,3,3,3}] tesqtip = compound of 64 ax ("tetrasquare triprism") {16}x{16}x{16}[64{4,3,3,3,3}] ??? = compound of 125 ax {20}x{20}x{20}[125{4,3,3,3,3}] ket = compound of 36 dotip ("heptacontiditetracositriacontidipeton") in mo regiment vertices coincide by 20 20 o3o3o3o3o *c3x [36 o3o3x3o3o x] gedak = compound of 2 (alternate) hax in ax army common intersection being a brox o3o3o3o3o4x [2 o3o3o3o3o4s] o3o3o3x3o4o cf. bidemicube details ??? = compound of 80 hejak beppim = compound of 9 hixip ("octadecaprismaticpentacontatetrapeton") in jak regiment vertices coincide by 4 moph = compound of 27 hixip ("pentacontatetraprismatichecatonhexacontidipeton") in jak regiment vertices coincide by 12 pok = compound of 36 hixip ("prismatoheptacontidipeton") in jak regiment vertices coincide by 16 |
stef = compound of 2 (dual) hop in o3o3m3m3o3o army common intersection being a fe [2{;3,3,3,3,3}]{3,3;3;3,3} cf. bisimplex details only 6D flag-transitive ta = compound of 5 hop {3,3;3,3,3}[5{;3,3,3,3,3}] ??? = compound of 240 hop in spil army madek = compound of 2 (inverted) jak ??? = compound of 80 jak in trim regiment eh = compound of 2 tetdip wa = compound of 20 tetdip in hax regiment vertices coincide by 10 ??? = compound of 80 tojak shigtip = compound of 8 trittip ("stellated-hexagonal triprism") {6}x{6}x{6}[8{3}x{3}x{3}] fentip = compound of 27 trittip ("fissary-enneagonal triprism") {9}x{9}x{9}[27{3}x{3}x{3}] tetritip = compound of 64 trittip ("tetratrigon triprism") {12}x{12}x{12}[64{3}x{3}x{3}] ye = compound of 80 trittip ("heptacosiicosapeton") in mo regiment vertices coincide by 30 ... |
---- 7D ----
Only few is known here so far ...
| sorted alphabetically by components | |
|---|---|
sadad = compound of 7 geep in naq army faq = compound of 36 he ("fissal pentacosihebdomecontahexaexon") in laq regiment vertices coincide by 20 ??? = compound of 135 hept in lin army ??? = compound of 2 hesa in hept army common intersection being a bersa o3o3o3o3o3o4x [2 o3o3o3o3o3o4s] o3o3o3o3x3o4o cf. bidemicube details ??? = compound of 8 naq in same army as rassiz ??? = compound of 30 naq in barz army (however has coincident elements, its blend is: hoshostaz) she = compound of 2 oca ("stellated hexadecaexon") (dual position) in o3o3o3m3o3o3o army common intersection being a he [2{;3,3,3,3,3,3}]{3,3,3;3,3,3} cf. bisimplex details flag-transitive sig = compound of 8 oca ("simplexial hexacontatetraexon") in hesa army |
saz = compound of 16 oca ("simplexial hecatonicosioctaexon") in hept army common intersection being a zee {4,3,3,3,3,3}[16{3,3,3,3,3,3}]{3,3,3,3,3,4} that one works for any Mersenne number dimension (D = 2k-1) fisq = compound of 72 oca ("fissal simplexic pentacosiheptacontahexaexon") (full symmetric) in lin army flag-transitive rush = compound of 2 roc ("rectified stellated hexadecaexon") (inverted orientations) in naq army rassiz = compound of 16 roc also: compound of 8 rush ("rectified simplexial hecatonicosioctaexon") deq = compound of 72 roc (full symmetric) in naq army it's the rectified fisq zeb = compound of 672 trahix in naq army ... |
---- 8D ----
Only few is known here so far ...
| sorted alphabetically by components | |
|---|---|
sib = compound of 2 ene ("stellated octadecazetton") (dual position) in o3o3o3m3m3o3o3o army common intersection being a be [2{;3,3,3,3,3,3,3}]{3,3,3;3;3,3,3} cf. bisimplex details flag-transitive |
say = compound of 1920 ene ("small myriaheptachiliadiacosioctacontazetton") in bif army (all 17280 facets remain oca) self-dual flag-transitive ??? = compound of 2 hocto in octo army common intersection being a bro o3o3o3o3o3o3o4x [2 o3o3o3o3o3o3o4s] o3o3o3o3o3x3o4o cf. bidemicube details ??? = compound of 15 rek in fy army vertices coincide by 7 ... |
Simplices in general do miss the inversion symmetry. Accordingly the compound of a mutually dual simplex pair xo3oo...oo3ox exists for any dimension. It obviously is flag-transitive (and thus regular in that sense) in any dimension. Also the intersection kernel not only can be provided generally in its combinatrical shape, but well in its correct metric size as well. In fact, eg. within odd dimensions, the (d-1)/2 dimensional elements of both components will intersect perpendicularily within a thereby each spanned affine hyperplane, thus resulting in the kernel's new vertices. (Sadly this idea however does not apply to even dimensions too, because that term then no longer is integral, while differnt dimensional elements would not have the same respective inradii.) However, by construction, the facet-wise inradius of the components and of the kernel surely will have to match in general. In dimensional sequence we get:
0D: intersection kernel of o and o is o when scaled down by 1 1D: intersection kernel of x and x is u when scaled down by 2 2D: intersection kernel of x3o and o3x is x3x when scaled down by 3 3D: intersection kernel of so, i.e. x3o3o and o3o3x is o3u3o when scaled down by 4 4D: intersection kernel of sted, i.e. x3o3o3o and o3o3o3x is o3x3x3o when scaled down by 5 5D: intersection kernel of stade, i.e. x3o3o3o3o and o3o3o3o3x is o3o3u3o3o when scaled down by 6 6D: intersection kernel of stef, i.e. x3o3o3o3o3o and o3o3o3o3o3x is o3o3x3x3o3o when scaled down by 7 7D: intersection kernel of she, i.e. x3o3o3o3o3o3o and o3o3o3o3o3o3x is o3o3o3u3o3o3o when scaled down by 8 8D: intersection kernel of sib, i.e. x3o3o3o3o3o3o3o and o3o3o3o3o3o3o3x is o3o3o3x3x3o3o3o when scaled down by 9 9D: intersection kernel of ..., i.e. x3o3o3o3o3o3o3o3o and o3o3o3o3o3o3o3o3x is o3o3o3o3u3o3o3o3o when scaled down by 10
I.e. the odd-dimensional intersection kernels here are just the mid-rectified simplices, while the even-dimensional ones are exactly the mid-truncated simplices, generally being scaled down by a radial factor of d+1 each.
Hypercubes generally can be vertex-alternated (snubbed) in order to produce the general (then q-scaled) demi(hyper)cube. If being holosnubbed instead, then both vertex-inscribed (q-scaled) demicubes would occur, i.e. the latter ones then align as a the general compound xo3oo3ox *b3oo...oo3oo. That very construction shows that it generally is vertex-regular (because it has the regular hypercube as its hull). When it comes to consider the intersection kernels of that series of compounds we first observe that the facets of these compounds are (still q-scaled) simplices (underneath the hypercube's vertices) as well as (likewise q-scaled) demicubes (within the facet planes of the hypercube). One observes further that those demicubes in turn form a subdimensional compound of 2 each. The very construction as a holosnub (of the hypercube) shows moreover that the 2 (full-dimensional) demicubes should intersect edge-midwise. Thence the facets of the searched for intersection kernel should be (1/q-scaled) rectified simplices and (also 1/q-scaled) rectified demicubes – both scalings wrt. the original edge size of the hypercube for sure, or, when given wrt. those of the (full-sized) compound instead, these scalings would become 1/2. This is obvious because of the midwise inscribed figure of all their face triangles is just a 1/2-scaled (dual) triangle. We further note additionally that the rectified demicube is nothing but the birectified hypercube. And, just as the demicubes occured above both full-dimensional as well as for facets, we too observe that the facets of the birectified hypercube would be just those mentioned rectified simplices and birectified hypercubes in turn. Thence we get finally:
3D: intersection kernel of so, i.e. x3o3o and o3o3x is x3o4o when scaled down by 2 4D: intersection kernel of haddet, i.e. x3o3o *b3o and o3o3x *b3o is o3x3o4o when scaled down by 2 5D: intersection kernel of todin, i.e. x3o3o *b3o3o and o3o3x *b3o3o is o3o3x3o4o when scaled down by 2 6D: intersection kernel of gedak, i.e. x3o3o *b3o3o3o and o3o3x *b3o3o3o is o3o3o3x3o4o when scaled down by 2 7D: intersection kernel of ..., i.e. x3o3o *b3o3o3o3o and o3o3x *b3o3o3o3o is o3o3o3o3x3o4o when scaled down by 2 8D: intersection kernel of ..., i.e. x3o3o *b3o3o3o3o3o and o3o3x *b3o3o3o3o3o is o3o3o3o3o3x3o4o when scaled down by 2 9D: intersection kernel of ..., i.e. x3o3o *b3o3o3o3o3o3o and o3o3x *b3o3o3o3o3o3o is o3o3o3o3o3o3x3o4o when scaled down by 2
I.e. those intersection kernels here generally are just the birectified hypercubes, each being scaled down by a radial factor of 2.
© 2004-2025 | top of page |