Acronym	srid
TOCID symbol	rID
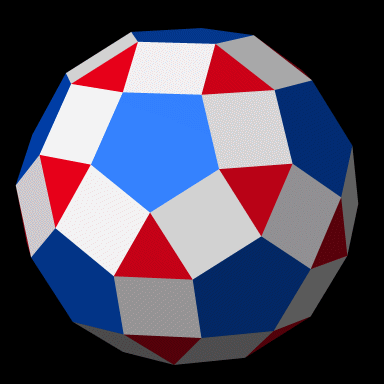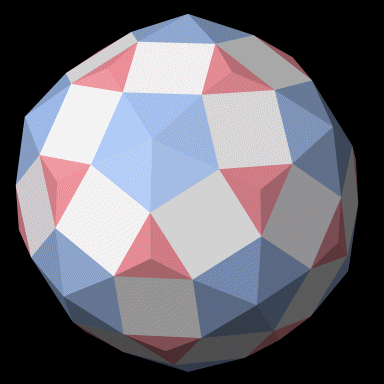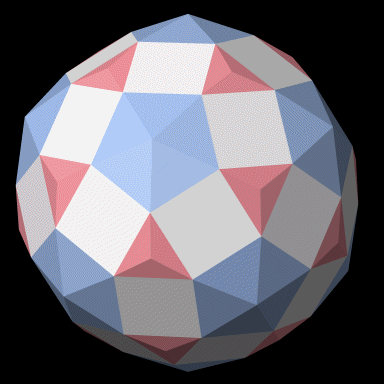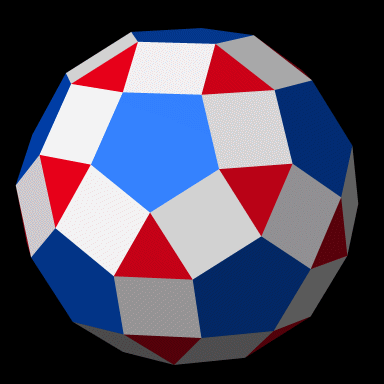
Name	small rhombicosidodecahedron
	© ©
Circumradius	sqrt[sqrt(5)+11/4] = 2.232951
Vertex figure	[3,4,5,4] = xf&#q
Vertex layers	Layer Symmetry Subsymmetries   o3o5o o3o . o . o . o5o 1 x3o5x x3o . {3} first x . x {4} first . o5x {5} first 2 x3f . o . F . x5x 3a o3U . F . f . x5f 3b F3x . 4a f3F . V . o . F5o 4b f . U 5 U3x . U . F . o5F 6a x3U . x . W . f5x 6b W . x 7 F3f . F . V . x5x 8a U3o . x . W . x5o opposite {5} 8b x3F . W . x 9 f3x . U . F   10a o3x . opposite {3} V . o 10b f . U 11   F . f 12 o . F 13 x . x opposite {4} (F=ff, U=fu, V=x+ff, W=x+fu)
General of army	(is itself convex)
Colonel of regiment	(is itself locally convex – other uniform polyhedral members: saddid   sird – other edge facetings)
Confer	Grünbaumian relatives: 2srid   related Johnson solids: pecu   dirid   gyrid   pabidrid   mabidrid   pagydrid   magydrid   gybadrid   bagydrid   tagygrid   tedrid   variations: a3b5c   q3o5x   f3o5x   v3o5f
External links

As abstract polytope srid is isomorphic to qrid, thereby replacing prograde pentagons by retrograde pentagrams.

Incidence matrix according to Dynkin symbol

x3o5x

. . . | 60 |  2  2 |  1  2  1
------+----+-------+---------
x . . |  2 | 60  * |  1  1  0
. . x |  2 |  * 60 |  0  1  1
------+----+-------+---------
x3o . |  3 |  3  0 | 20  *  *
x . x |  4 |  2  2 |  * 30  *
. o5x |  5 |  0  5 |  *  * 12

x5/4o3/2x 

.   .   . | 60 |  2  2 |  1  2  1
----------+----+-------+---------
x   .   . |  2 | 60  * |  1  1  0
.   .   x |  2 |  * 60 |  0  1  1
----------+----+-------+---------
x5/4o   . |  5 |  5  0 | 12  *  *
x   .   x |  4 |  2  2 |  * 30  *
.   o3/2x |  3 |  0  3 |  *  * 20