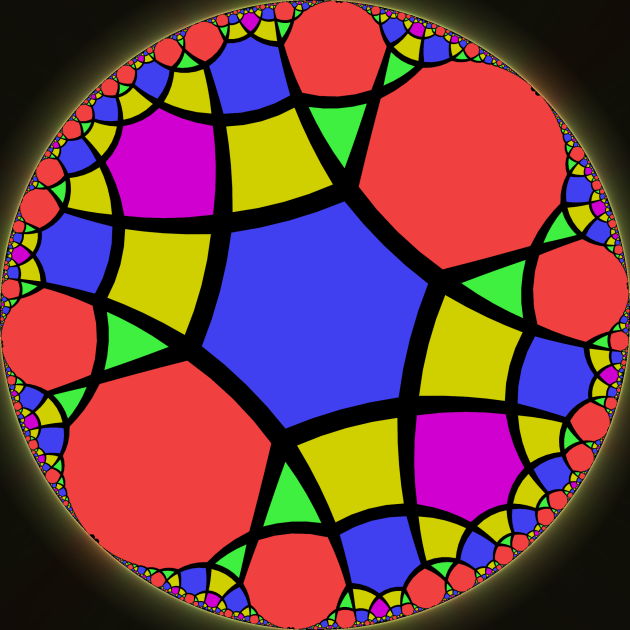
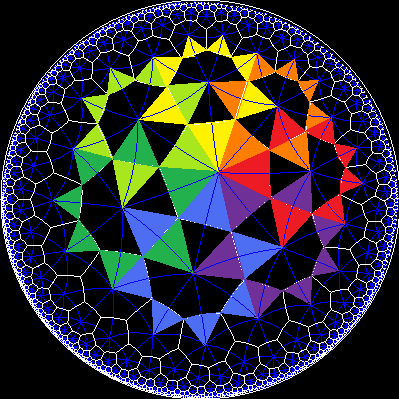
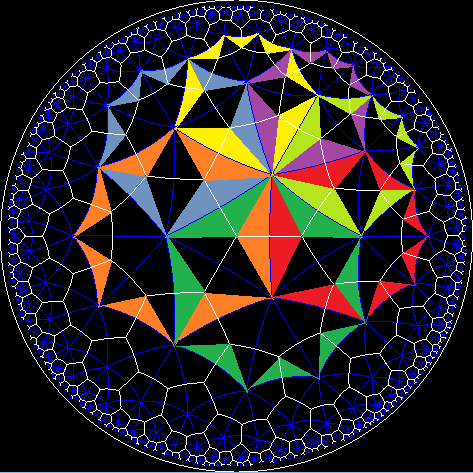
Hyperbolic Tesselations

• Simplicial Fundamental Domains
  • 2D Tesselations
    • Compact Tilings
    • Non-Compact Tilings
  • 3D Tesselations
    • Compact Honeycombs
    • Non-Compact Honeycombs
  • 4D Tesselations
    • Compact Tetracombs
    • Non-Compact Tetracombs
  • 5D Tesselations (Pentacombs)
  • 6D Tesselations (Hexacombs)
  • 7D Tesselations (Heptacombs)
  • 8D Tesselations (Octacombs)
  • 9D Tesselations (Enneacombs)
  • ...
• General Fundamental Domains
  • 2D More general Tilings
    • A Theorem on completely even-sided tilings and some therefrom derivable ones
    • A generalization of Dynkin diagrams
    • A fully general quest for tilings of type [a,a,a,b] results in up to 4 globally differnet tilings each
    • A similar research for tilings of type [a,a,a,a,b] even results in up to 14 globally different ones
    • ...
  • 3D More general Honeycombs
  • ...

Tesselations based on simplicial domains

Both, spherical space tesselations (aka polytopes) and Euclidean space tesselations, base their reflection symmetry groups on simplicial fundamental domains. In 2D spherical geometry those are known as Schwarz triangles, in 3D they are called Goursat tetrahedra. In Euclidean space this restriction was relaxed in so far as parallel mirrors would be allowed, i.e. ones which don't intersect anymore. The submultiplicative number of the dihedral angle (i.e. the link mark) in such cases was set to ∞. This results in an offshore vertex of the former simplicial fundamental domain, drifted far away to infinity. But that's all what can happen. Now taking over this view onto hyperbolic space, already gives lots of stuff to deal with. – Even though, it should be noted, that this still is not the end of the story, there are other possibilities too.

Besides from the shape and the size of the fundamental domain, for instance its extend to infinity ("cusps"), also the extend of the tiles can be considered. For Euclidean tilings, honeycombs, etc. there are 2 classes, one using only finite tiles (polytopes of spherical geometry) but building up complexes which nonetheless fill all of Euclidean space, or alternatively those other ones, which use additionally infinitely extended tiles, i.e. euclidean tilings, honeycombs, etc. from one dimension less as building blocks within the next dimension. In hyperbolic space, there even is one case more:

1. compact:   hyperbolic tesselations using finite (spherical polytopial) tiles only
2. paracompact:   hyperbolic tesselations which additionally (to class 1) include lower dimensional euclidean tesselations as infinite tiles
3. hypercompact:   hyperbolic tesselations which additionally (to either class 1 or 2) include lower dimensional hyperbolic constituends

However, strictly speaking, those 3 terms in fact more relate to the property of the fundamental domain of kaleidoscopical construction, than to the thereby obtained facets (tiles) themselves. This esp. will become relevant when dealing with non-convex tiles. That distintion then relates to bounded domains, to infinitely size and still finite volume domains, and to infinite volumed domains respectively. But, when dealing with convex tiles only, the above, much easier deducible description indeed coincides with that.

Finally, right from their definition, Dynkin symbols, both for the mere symmetry groups, and for the described polytopes or tesselations, are essentially based on that simplicial restriction and thus not versatile outside that very scope. (But attempts for more general Coxeter domains can be made too.)

A further class are laminates. These are built from infinite regions of a tesselation, which are made from compact tiles only. Those regions further are bounded by some infinite (pseudo) facetings, which serve as reflection, glide-reflection, etc. As such laminates belong to class 1. – Note that these facets might become real ones within class 3: There such laminar regions could occur between bollotiles (tiles of hyperbolic structure themselves). Clearly we should restrict here to cases, where these bollotiles do have exactly the same curvature as the whole tesselation. Because then those behave like hemi-facets of spherical space: One could consider the external, infinitely multiple self-blends of those tesselations, which would just blend out these bollotiles. That is, these serve as mirrors, each reflecting the laminar region. The such derived laminate accordingly is called the lamina-truncate of the un-blended structure. Both these notions were introduced by W.Krieger.

©

Further fun would occur with laminates in the non-uniform CRF realm. For instance when two different laminates have the same curvature as well as the same margin tiling, because then instead of simply reflecting the laminate itself these could be used alternatingly instead, cf. the right picture with the mixture of (4,6,4,6) and (3,∞,3,∞).

Just as for spherical geometry, the hyperbolic one has also a non-vanishing uniform curvature. Accordingly a circumradius here too is well-defined. Only that this quantity would provide purely imaginary values for hyperbolics. In fact, the formulas for radius derivation, based on a given Wythoffian Dynkin diagram (being implemented within the spreadsheet, which is provided at the download page), would work here exactly the same.

----
 2D  Tilings (up)
----

In this dimension any Dynkin symbol of type oPoQo would be hyperbolic, whenever 1/P + 1/Q < 1/2 (or equivalently (P-2)(Q-2) > 4). (In fact ">" within the first formula would qualify spherical, and "=" would qualify euclidean.) For convex cases, i.e. integral line mark numbers, the single euclidean solution for a loop Dynkin symbol is o3o3o3*a; no spherical does exist. Anything beyond thus qualifies as a symmetry group of hyperbolic space. As long as finite line mark numbers are used only, for this dimension we remain within the above mentioned first class. – But even the general hyperbolic case for oPoQoR*a can be formalized by 1/P + 1/Q + 1/R < 1 for any rational P,Q,R (each >1), thereby extending the above formula to cases with R<>2 as well.

With respect to the node markings we will have exactly the same cases as given explicitly in that listing for the general Schwarz triangle oPoQoR*a (providing cases and their general incidence matrices; although there, in addition for each of those general incidence matrix cases, so far only links to spherical and euclidean space representants are provided).

Compact Tilings

(A nice applet for visualization of 2D hyperbolic tilings (as well as euclidean ones) is tyler. In case make sure to check "hyperbolic". In fact it was designed to work beyond triangular domains as well.)

x3o7o - hetrat
o3x7o - thet
o3o7x - heat
x3x7o - thetrat
x3o7x - srothet
o3x7x - theat
x3x7x - grothet

x3o8o - otrat
o3x8o - toct
o3o8x - ocat
x3x8o - totrat
x3o8x - srotoct
o3x8x - tocat
x3x8x - grotoct

x3o10o - detrat
o3x10o - tidect
o3o10x - decat
x3x10o - tidtrat
x3o10x - srotdect
o3x10x - tidecat
x3x10x - grotdect

x4o5o - pesquat
o4x5o - tepet
o4o5x - peat
x4x5o - topesquat
x4o5x - srotepet
o4x5x - topeat
x4x5x - grotepet

x4o6o - hisquat
o4x6o - tehat
o4o6x - shexat
x4x6o - thisquat
x4o6x - srotehat
o4x6x - toshexat
x4x6x - grotehat

x4o8o - osquat
o4x8o - teoct
o4o8x - socat
x4x8o - ocat
x4o8x - sroteoct
o4x8x - tosocat
x4x8x - groteoct

s3s7s - snathet

o3o8s - ditetsquat
        (old: dittitecat)
x3o8s - sittitetrat
o3x8s - toct
x3x8s - totrat

s3s8o - stititet
s3s8x - srotoct

s3s8s - snatoct

s3s10s - snatdect
...

s4o5o - pepat
s4x5o - tepet
s4o5x - topepat
s4x5x - topeat

o4s5s
x4s5s - srotepet

s4s5s - stepet

s4o6o - hihexat
s4o6x - thihexat
o4s6o
x4s6x - srotehat
o4o6s - ditetetrat
x4o6s
...

s4s6o
s4s6x - srotehat
s4o6s
s4o6s'
o4s6s
x4s6s - srotehat
...

s4s6s

s4o8o - ococat
o4s8o
o4o8s - osquat
s4s8o - ditetsquat
        (old: dittitecat)
s4o8s
o4s8s
s4s8s
...

x5o5o - pepat
o5x5o - peat
x5x5o - topepat
x5o5x - tepet
x5x5x - topeat

x5o6o - hipat
o5x6o - phat
o5o6x - phexat
x5x6o
x5o6x
o5x6x
x5x6x

x5o10o - depat
o5x10o - pidect
o5o10x - pedecat
x5x10o - decat
x5o10x - sropdect
o5x10x - topdecat
x5x10x - gropdect

x6o6o - hihexat
o6x6o - shexat
x6x6o - thihexat
x6o6x - tehat
x6x6x - toshexat

x8o8o - ococat
o8x8o - socat
x8x8o - tococat
x8o8x - teoct
x8x8x - tosocat

s5s5s - spepat

o5o10s - depat
s5s10o
s5s10s
...

s6o6o
o6s6o
x6s6o
s6s6o
s6o6s
s6s6s
...

s8o8o
o8s8o - osquat
s8s8o
s8o8s
s8s8s
...

x3o3o4*a - ditetsquat
           (old: dittitecat)
o3x3o4*a - otrat
x3x3o4*a - sittitetrat
x3o3x4*a - toct
x3x3x4*a - totrat

x3o4o4*a - ditetetrat
o3o4x4*a - hisquat
x3x4o4*a - tehat
x3o4x4*a - sittiteteat
x3x4x4*a - thisquat

x3o4o5*a - ditetpeat
o3x4o5*a
o3o4x5*a
x3x4o5*a
x3o4x5*a
o3x4x5*a
x3x4x5*a

x3o5o5*a - diptapt
o3o5x5*a - hipat
x3x5o5*a - phat
x3o5x5*a
x3x5x5*a

x4o4o4*a - osquat
x4x4o4*a - teoct
x4x4x4*a - ocat

s3s3s4*a - stititet

o3o4s4*a - hihexat
x3o4s4*a
s3s4o4*a
s3s4s4*a
...

s3s4s5*a

s4o4o4*a - ococat
s4s4o4*a
s4s4s4*a - ditetsquat
           (old: dittitecat)
...

In contrast to the situation of euclidean space tilings for both the spherical and hyperbolical tilings the size of the tiles is fixed by the absolute geometry of the filled manifold, i.e. its curvature, and the to be used vertex figure. For instance, let P₀ be a vertex of xPoQo, let P₁ be the center of an adjacent edge, and P₂ the center of an adjacent face (in fact a xPo), then the distances φ = P₀P₁, χ = P₀P₂, and ψ = P₁P₂ depend on the absolute geometry of oPoQo via

cosh(φ) = cos(π/P) / sin(π/Q)
cosh(χ) = cot(π/P) · cot(π/Q)
cosh(ψ) = cos(π/Q) / sin(π/P)

Therefore xPoQo itself can be described as a tiling with edge length 2φ, having Q P-gons at each vertex, and the P-gons will have a circumradius of χ and an inradius of ψ.

The only regular star-tesselations have the symmetries o-P-o-P/2-o, here P being an odd integer greater than 5. All those star-tesselations would have density 3. (The case P = 5 already describes the spherical space tesselation or polyhedron sissid respectively gad.) In fact, x-P/2-o-P-o are derived as stellations of xPo3o. Dually, the edge-skeletons of x-P-o-P/2-o and of x3oPo are the same.

Non-regular other compact star-tesselations however do exist, cf. eg. siditetpeat. (In fact, that examplified one occurs as a faceting of x5o4o, where the purple pentagrams are just inscribed into the pentagons and the green squares are simply its vertex figures.) Or cf. the other shown example o8x8/3o3*a (which likewise is inscribable, then into x8o3o).

			©
x7/2o7o - sheat	x7o7/2o - gheat		o5/2x4o4*a - siditetpeat	o8x8/3o3*a - ditoot

Paracompact Tilings

©

As there is just a single 1D euclidean space tiling, aze, the only wythoffian tilings of hyperbolic plane, which use euclidean tiles in addition to polygons, are based on the reflection groups oPoQoR*a, where still 1/P + 1/Q + 1/R < 1, but at least one of those link marks being infinite. Here aze then will be understood to describe an horocyclic tile (a.k.a. apeirogon).

x3o∞o - aztrat
o3x∞o - tazt
o3o∞x - azat
x3x∞o - taztrat
x3o∞x - srotazt
o3x∞x - tazat
x3x∞x - grotazt

x4o∞o - asquat
o4x∞o - tezt
o4o∞x - squazat
x4x∞o - tasquat
x4o∞x - srotezt
o4x∞x - tosquazat
x4x∞x - grotezt

x5o∞o - azpat
o5x∞o - pazt
o5o∞x - pazat
x5x∞o - tazpat
o5x∞x - topazat
...

x6o∞o - azhexat
o6x∞o - hazt
o6o∞x - hazat
x6x∞o - tazhexat
o6x∞x - thazat
...

x∞o∞o - azazat
o∞x∞o - squazat
x∞x∞o - azat
x∞o∞x - tezt
x∞x∞x - tosquazat

x3o3o∞*a
o3x3o∞*a - aztrat
x3x3o∞*a
x3o3x∞*a - tazt
x3x3x∞*a - taztrat

...
x3o5x∞*a - dazatrapeat
...

...
x4o4x∞*a - tezt
x4x4x∞*a - tasquat
...

...
x6o6x∞*a - hazt
...

x3o∞o∞*a
o3o∞x∞*a
x3x∞o∞*a - hazt
x3o∞x∞*a
x3x∞x∞*a

...

x∞o∞o∞*a - azazat
x∞x∞o∞*a - squazat
x∞x∞x∞*a - azat

o3o∞s
s3s∞o
s3s∞s - snatazt

o4s∞s - sazazat
...

s∞s∞s - sazazat

s3s3s∞*a

...

s3s∞s∞*a

...

s∞s∞s∞*a

(For tilings with more general fundamental domains cf. Coxeter domains.)

----
 3D  Honeycombs (up)
----

Compact Honeycombs

Here the restriction to finite tiles is much more effective, at least if being considered with respect to non-product honeycombs. For convex cases (integral line mark numbers) we only have the following 9 irreducible symmetry groups, resp. the therefrom derived listed Wythoffian hyperbolic honeycombs.

x3o5o3o - ikhon
o3x5o3o - rih
x3x5o3o - tih
x3o5x3o - srih
x3o5o3x - spiddih
o3x5x3o - dih
x3x5x3o - grih
x3x5o3x - prih
x3x5x3x - gipiddih

x4o3o5o - pechon
o4x3o5o - ripech
o4o3x5o - riddoh
o4o3o5x - doehon
x4x3o5o - tipech
x4o3x5o - sripech
x4o3o5x - sidpicdoh
o4x3x5o - ciddoh
o4x3o5x - sriddoh
o4o3x5x - tiddoh
x4x3x5o - gripech
x4x3o5x - priddoh
x4o3x5x - pripech
o4x3x5x - griddoh
x4x3x5x - gidpicdoh

x5o3o5o - pedhon
o5x3o5o - ripped
x5x3o5o - tipped
x5o3x5o - sripped
x5o3o5x - spidded
o5x3x5o - diddoh
x5x3x5o - gripped
x5x3o5x - pripped
x5x3x5x - gipidded

x3o3o *b5o - apech
o3x3o *b5o - riddoh
o3o3o *b5x - doehon
x3x3o *b5o - tapech
x3o3x *b5o - ripech
x3o3o *b5x - birapech
o3x3o *b5x - tiddoh
x3x3x *b5o - ciddoh
x3x3o *b5x - bitapech
x3x3x *b5x - griddoh

s3s5s3s - snih *) 
...

s4o3o5o - apech
s4o3x5o - tapech
s4o3o5x - birapech
s4o3x5x - bitapech
...
o4x3o5β
...

s5s3s5s *)
β5o3x5o
...

...

x3o3o3o4*a - gadtatdic
o3x3o3o4*a - gacocaddit
x3x3o3o4*a - cytitch
x3o3x3o4*a - ritch
x3o3o3x4*a - cyticth
o3x3x3o4*a - cytoth
x3x3x3o4*a - titdoh
x3x3o3x4*a - titch
x3x3x3x4*a - otitch

x3o4o3o4*a - cohon
x3x4o3o4*a - cytoch
x3o4x3o4*a - racoh
x3o4o3x4*a - cytacoh
x3x4x3o4*a - tucoh
x3x4x3x4*a - otacoh

x3o3o3o5*a
o3x3o3o5*a
x3x3o3o5*a
x3o3x3o5*a
x3o3o3x5*a
o3x3x3o5*a
x3x3x3o5*a
x3x3o3x5*a
x3x3x3x5*a

x3o4o3o5*a
o3x4o3o5*a
x3x4o3o5*a
x3o4x3o5*a
x3o4o3x5*a
o3x4x3o5*a
x3x4x3o5*a
x3x4o3x5*a
x3x4x3x5*a

x3o5o3o5*a
x3x5o3o5*a
x3o5x3o5*a
x3o5o3x5*a
x3x5x3o5*a
x3x5x3x5*a

...

...

...

o3β4β3o5*a *)
...

...

*) These figures occur only as alternations. An all unit edged representation does not exist.

Trying to extend the class with linear Dynkin diagrams into non-convex realms, i.e. asking for compact regular star-honeycombs, would come out to be hopeless either. In fact, the actual choice of any Kepler-Poinsot polyhedron (as well for cell as for vertex figure) produces spherical curvatures only. – But this would not bother the other (non-linear) types of Dynkin diagram structures (nor non-compact linears)!

x5/2o5o3o5*a
o5/2o5x3o5*a
x5/2x5o3o5*a
x5/2o5x3o5*a
x5/2o5o3x5*a
o5/2o5x3x5*a
x5/2x5x3o5*a - [Grünbaumian]
x5/2x5o3x5*a - [Grünbaumian]
x5/2o5x3x5*a
x5/2x5x3x5*a - [Grünbaumian]

x5o3o3o5/2*b - ditdih
o5x3o3o5/2*b
o5o3x3o5/2*b
o5o3o3x5/2*b
x5x3o3o5/2*b
x5o3x3o5/2*b
x5o3o3x5/2*b
o5x3x3o5/2*b
o5x3o3x5/2*b - [Grünbaumian]
o5o3x3x5/2*b
x5x3x3o5/2*b
x5x3o3x5/2*b - [Grünbaumian]
x5o3x3x5/2*b
o5x3x3x5/2*b - [Grünbaumian]
x5x3x3x5/2*b - [Grünbaumian]

Also to class 1 would belong additionally all the honeycomb products of any 2D hyperbolic tiling with (an appropriate hyperbolic space version of) aze. This is due to the fact that in this product neither of the full-dimensional elements themselves (considered as bodies) remain true elements of the product (although those could be seen as being pseudo elements thereof).

More generally the laminates belong here. For quite long the only known uniform lamina-truncates (cf. definition) are lamina-trunc( x4x3o8o ) and lamina-trunc( o8o4x *b3x ). Within 2023 Mecejide came up with 2 additional ones, then also showing up their relations to Coxeter domains.

Already in 1997 W. Krieger found an infinite series of uniform, pyritohedral vertex figured honeycombs belonging here as well (each featuring 8 cubes and 6 p-gonal prisms per vertex). Other singular cases would be spd{3,5,3} (with 2 does, 6 paps, and 6 ikes per vertex) and pd{3,5,3} (with 4 does and 12 paps per vertex), as detailed under subsymmetric diminishings.

In early 2021 a compact non-Wythoffian though uniform hyperbolic was found by a guy calling himself "Grand Antiprism", the octsnich, having 2 octs and 8 snics per vertex.

In reply W. Krieger found x3o:s3s4s, a compact non-Wythoffian with 32 tets and 6 octs per vertex, the vertex figure of which happens to be snic. She then even found that its rectification o3x:s3s4s and its truncation x3x:s3s4s, then each providing snics for cells, would be likewise valide. In 2022 the author extrapolated these moreover into the general ones x3o:s3sPs, o3x:s3sPs, and x3x:s3sPs, then surely mostly hypercompact each.

Paracompact Honeycombs

Irreducible 3D hyperbolic reflectional symmetry groups within class 2, with finite integral link marks only, would group into the following classes, which would include euclidean tilings in addition to spherical space tiles. Those noncompact hyperbolic groups can be considered over-extended forms, like the affine groups, adding a second node in sequence to the first added node, with letter names marked up by a '++' superscript.

o3o6o3o

o3o4o4o
o4o4o4o

o3o3o6o
o4o3o6o
o5o3o6o
o6o3o6o

o3o3o *b6o

o4o4o *b3o
o4o4o *b4o

o3o3o3o3*b
o4o3o3o3*b
o5o3o3o3*b
o6o3o3o3*b

x3o6o3o - trah
o3x6o3o - ritrah
x3x6o3o - hexah
x3o6x3o - sritrah
x3o6o3x - spidditrah
o3x6x3o - ditrah
x3x6x3o - gritrah
x3x6o3x - pritrah
x3x6x3x - gipidditrah

x3o4o4o - octh
o3x4o4o - rocth
o3o4x4o - risquah
o3o4o4x - squah
x3x4o4o - tocth
x3o4x4o - srocth
x3o4o4x - sidposquah
o3x4x4o - osquah
o3x4o4x - srisquah
o3o4x4x - tisquah
x3x4x4o - grocth
x3x4o4x - prisquah
x3o4x4x - procth
o3x4x4x - grisquah
x3x4x4x - gidposquah

x4o4o4o - sisquah
o4x4o4o - squah
x4x4o4o - tissish
x4o4x4o - risquah
x4o4o4x - spiddish
o4x4x4o - dish
x4x4x4o - tisquah
x4x4o4x - prissish
x4x4x4x - gipiddish

x3o3o6o - thon
o3x3o6o - rath
o3o3x6o - rihexah
o3o3o6x - hexah
...

x4o3o6o - hachon
o4x3o6o - rihach
o4o3x6o - rishexah
o4o3o6x - shexah
x4o3o6x - sidpichexah
o4x3x6o - chexah
...

x5o3o6o - hedhon
o5o3o6x - phexah
...

x6o3o6o - hihexah
o6x3o6o - rihihexah
x6o3o6x - spiddihexah
o6x3x6o - hexah
...

x3o3o *b6o - ahach
o3x3o *b6o - tachach
x3o3o *b6x - birachach
x3x3o *b6x - bitachach
...

x4o4o *b3o
o4x4o *b3o
o4o4o *b3x
x4x4o *b3o
x4o4x *b3o - risquah
x4o4o *b3x
o4x4o *b3x - tocth
x4x4x *b3o
x4x4o *b3x
x4o4x *b3x
x4x4x *b3x

x4o4o *b4o - sisquah
o4x4o *b4o - squah
x4x4o *b4o - tissish
x4o4x *b4o - squah
x4x4x *b4o
x4o4x *b4x - risquah
x4x4x *b4x

x3o3o3o3*b - thon
o3x3o3o3*b - rath
o3o3x3o3*b - ahexah
x3x3o3o3*b
x3o3x3o3*b - birahexah
o3x3x3o3*b - tahexah
o3o3x3x3*b
x3x3x3o3*b - bitahexah
x3o3x3x3*b
o3x3x3x3*b
x3x3x3x3*b

x4o3o3o3*b - hachon
o4x3o3o3*b
o4o3x3o3*b - ashexah
x4x3o3o3*b
x4o3x3o3*b - birashexah
o4x3x3o3*b - tashexah
o4o3x3x3*b
x4x3x3o3*b - bitashexah
x4o3x3x3*b
o4x3x3x3*b
x4x3x3x3*b

x5o3o3o3*b
o5x3o3o3*b
o5o3x3o3*b - aphexah
x5x3o3o3*b
x5o3x3o3*b - biraphexah
o5x3x3o3*b - taphexah
o5o3x3x3*b
x5x3x3o3*b - bitaphexah
x5o3x3x3*b
o5x3x3x3*b
x5x3x3x3*b

x6o3o3o3*b - hihexah
o6x3o3o3*b - rihihexah
o6o3x3o3*b - trah
x6x3o3o3*b
x6o3x3o3*b
o6x3x3o3*b - ritrah
o6o3x3x3*b
x6x3x3o3*b
x6o3x3x3*b
o6x3x3x3*b - hexah
x6x3x3x3*b

s3s6o3o - ahexah
s3s6o3x - pristrah **)
s3s6s3s - snatrah *) 
...

o3o4o4s
o3o4s4o
x3o4s4o
s3s4o4o **)
x3x4o4s
s3s4o4x **)
s3s4o4s' *)
...

s4o4o4o - sisquah
o4s4o4o
s4o4s4o
s4o4o4s
...

o3o3o6s - ahexah
...

s4o3o6o - ahach
o4o3o6s - ashexah
s4o3o6x - birachach
x4o3o6s - birashexah
s4o3o6s' - quishexah
...

o5o3o6s - aphexah
...

o6s3s6o - ahexah
...

x3o3o *b6s - quishexah
...

s4o4s *b3o
x4s4o *b3s *)
...

s4o4o *b4o - sisquah
o4s4o *b4o
s4o4s *b4o
...

s4o3o3o3*b
s4o3x3o3*b - quishexah
...

o6s3s3s3*b - ahexah
...

o3o3o3o6*a
o3o4o3o6*a
o3o5o3o6*a
o3o6o3o6*a

o3o3o4o4*a
o3o4o4o4*a
o4o4o4o4*a

o3o3o3o3*a3*c

o3o3o3o3*a3*c *b3*d

...

...

...

x3o6o3o6*a
x3x6o3o6*a - shexah
...

o3o3o4x4*a
...

x3o4o4o4*a
...
o3o4x4o4*a
...

x4o4o4o4*a - sisquah
x4x4o4o4*a
x4o4x4o4*a - squah
x4x4x4o4*a
x4x4x4x4*a

o3x3o3o3*a3*c - ahach
x3x3o3o3*a3*c - quishexah
...

x3o3o3o3*a3*c *b3*d - trah
x3x3o3o3*a3*c *b3*d - rihihexah
x3x3x3o3*a3*c *b3*d - ritrah
x3x3x3x3*a3*c *b3*d - hexah

s3s6o3o6*a - ashexah
...

o3o3o4s4*a
...

o3o4s4o4*a
...

s4o4o4o4*a - sisquah
s4o4s4o4*a
...

...

s3s3s3s3*a3*c *b3*d - ahexah
...

*) These figures occur only as alternations. An all unit edged representation does not exist.
**) Although being rescalable to equal edge lengths, those figures are only scaliform.

E.g. the linear diagrams oPoQoRo, in order to be at most paracompact, in general would require to bow under both, (P-2)(Q-2) ≤ 4 and (Q-2)(R-2) ≤ 4. Further, those numbers again can be used to derive the according geometry: Any xPoQoRo consists of xPoQo-cells only, those having edges of length 2φ, an circumradius of χ, and an inradius of ψ, where

cosh(φ) = cos(π/P) sin(π/R) / sin(π/hQ,R)
cosh(ψ) = sin(π/P) cos(π/R) / sin(π/hP,Q)
cosh(χ) = cos(π/P) cos(π/Q) cos(π/R) / sin(π/hP,Q) sin(π/hQ,R)
with:
cos2(π/hP,Q) = cos2(π/P) + cos2(π/Q)

(The last equation clearly evaluates into h_P,2 = P,  h_2,Q = Q,  h_3,3 = 4,  h_3,4 = h_4,3 = 6,  h_3,5 = h_5,3 = 10,  h_3,6 = h_4,4 = h_6,3 = ∞. Geometrically this number is related to the Petrie polygon of each of the corresponding regular polyhedra or tilings, i.e. their largest regular shadow polygon.)

Hypercompact Honeycombs

As any neither compact nor paracompact hyperbolic honeycomb would be hypercompact, those clearly have infinite count. So there cannot be a complete listing, not even tentatively. Only a few, randomly selected examples might follow here.

x3o3o7o - hetoh
o3x3o7o - rahet
o3o3x7o - raheath
o3o3o7x - heath

o3o4o8x
o3o4o8s
o3o5o10x
o3o5o10s °)

o4x4oPo (for P>4)
o4s4oPo (for P>4) °)

x4x4o5o

x4oPo4x (for P>4)
s4oPo4s (for P>4) °)

x4x3o8o
x4x3x8o

o6o5x∞x

x8o4o *b3o
o8o4x *b3x

x3o5x *b4o

x5o5o *b5/2o (non-convex)

o3o3o4x4*b
o3x3x4o4*b
o3x3x4x4*b
o3o3o4s4*b °)

x4o3o4x4*b
s4o3o4s4*b **)

o3o4x4o4*b

o3o5x5o5*b

o6o5x∞x3*b

x3o5x5o3*a

o4x4o4x4*aP*c (for P>2)
o4s4o4s4*aP*c (for P>2) °)

x xPoQo (for any hyperbolic xPoQo)
x oPxQo (for any hyperbolic oPxQo)
x xPxQo (for any hyperbolic xPxQo)
x xPoQx (for any hyperbolic xPoQx)
x xPxQx (for any hyperbolic xPxQx)

x x3o8o - otratip
x x3x8o - totratip

x o4o5x - peatip
x o4o6x - shexatip

°) These figures not only allow for an all unit edged representation but then even become uniform.
**) Although being rescalable to equal edge lengths, those figures are only scaliform.

Although x4o3o4x4*b is itself not quasiregular it still allows for rectification as well as truncation, as all of its faces are alike squares. This then results in the still hypercompact CRF honeycombs rect( x4o3o4x4*b ) and trunc( x4o3o4x4*b ) respectively, which then incorporate Johnson solids for (some of its) cells.

(For honeycombs with more general fundamental domains cf. Coxeter domains.)

And starry hypercompact honeycombs do exist as well. Just to mention an in 2023 provided example found by B. Klein: (idtid+tidect). In fact, this still uniform honeycomb comes out to be an edge-faceting of the likewise starry wythoffian compact honeycomb o3x4o4o5/2*b. But even regular starry hypercompact honeycombs do exist, eg. x5/2o5o4o.

----
 4D  Tetracombs (up)
----

Compact Tetracombs

Dwelling within class 1 only, is equally restrictive here. Potential irreducible symmetries are:

x3o3o3o5o - pente
o3x3o3o5o - rapente
o3o3x3o5o
o3o3o3x5o - rahitte
o3o3o3o5x - hitte
...

x4o3o3o5o - pitest
o4x3o3o5o
o4o3x3o5o
o4o3o3x5o
o4o3o3o5x - shitte
...

x5o3o3o5o - phitte
o5x3o3o5o - raphitte
o5o3x3o5o
...
x5o3o3o5x

x3o3o5o5/2o
o3x3o5o5/2o
o3o3x5o5/2o
o3o3o5x5/2o
o3o3o5o5/2x
...

x3o5o5/2o5o
o3x5o5/2o5o
o3o5x5/2o5o
o3o5o5/2x5o
o3o5o5/2o5x
...

x3o3o *b3o5o
o3x3o *b3o5o
o3o3o *b3x5o
o3o3o *b3o5x
...

x3o3o3o3o4*a
o3x3o3o3o4*a
o3o3x3o3o4*a
...

...

...

...

...

...

Only the convex symmetries are exhausted within the table. This already is enough to show that here there are exactly 5 convex regulars and 4 regular star-tetracombs within class 1.

Beyond 4D, there will be no irreducible symmetry within class 1 anymore.

Within this dimension as well lamina-truncate uniforms are known, contit and odipt, which btw. are the only known non-regular dual pair, both of which are uniform.

x3o3o5o5/2o
o3x3o5o5/2o
o3o3x5o5/2o
o3o3o5x5/2o
o3o3o5o5/2x
x3x3o5o5/2o
x3o3x5o5/2o
x3o3o5x5/2o
x3o3o5o5/2x
o3x3x5o5/2o
o3x3o5x5/2o
o3x3o5o5/2x
o3o3x5x5/2o
o3o3x5o5/2x
o3o3o5x5/2x - [Grünbaumian]
x3x3x5o5/2o
x3x3o5x5/2o
x3x3o5o5/2x
x3o3x5x5/2o
x3o3x5o5/2x
x3o3o5x5/2x - [Grünbaumian]
o3x3x5x5/2o
o3x3x5o5/2x
o3x3o5x5/2x - [Grünbaumian]
o3o3x5x5/2x - [Grünbaumian]
x3x3x5x5/2o
x3x3x5o5/2x
x3x3o5x5/2x - [Grünbaumian]
x3o3x5x5/2x - [Grünbaumian]
o3x3x5x5/2x - [Grünbaumian]
x3x3x5x5/2x - [Grünbaumian]

x3o5o5/2o5o
o3x5o5/2o5o
o3o5x5/2o5o
o3o5o5/2x5o
o3o5o5/2o5x
x3x5o5/2o5o
x3o5x5/2o5o
x3o5o5/2x5o
x3o5o5/2o5x
o3x5x5/2o5o
o3x5o5/2x5o
o3x5o5/2o5x - [Grünbaumian]
o3o5x5/2x5o - [Grünbaumian]
o3o5x5/2o5x
o3o5o5/2x5x
x3x5x5/2o5o
x3x5o5/2x5o
x3x5o5/2o5x - [Grünbaumian]
x3o5x5/2x5o - [Grünbaumian]
x3o5x5/2o5x
x3o5o5/2x5x
o3x5x5/2x5o - [Grünbaumian]
o3x5x5/2o5x
o3x5o5/2x5x
o3o5x5/2x5x - [Grünbaumian]
x3x5x5/2x5o - [Grünbaumian]
x3x5x5/2o5x
x3x5o5/2x5x
x3o5x5/2x5x - [Grünbaumian]
o3x5x5/2x5x - [Grünbaumian]
x3x5x5/2x5x - [Grünbaumian]

Paracompact Tetracombs

The potential irreducible convex cases within class 2 are provided by the following groups. Those provide 2 more regular figures.

o3o4o3o4o

o3o3o *b4o3o
o3o4o *b3o3o
o3o4o *b3o4o

o3o4o *b3o *b3o

o3o3o3o3o3*b
o4o3o3o3o3*b

o3o3o4o3o4*a

o3o3o3o3*a3o3*c

x3o4o3o4o
o3x4o3o4o
o3o4x3o4o
o3o4o3x4o
o3o4o3o4x - chont
...
x3x4o3o4o
...

...
s3s4o3o4o
o3o4o3o4s
...

...

...

...

...

...

Hypercompact Tetracombs

As any neither compact nor paracompact hyperbolic tetracomb would be hypercompact, those clearly have infinite count. So there cannot be a complete listing, not even tentatively. Only a few, randomly selected examples might follow here.

o3x4x3o8o
x3x4x3x8o

o4x4o3*a4x4o3*a

o4s4o3*a4s4o3*a *)

x3o3o3o *b3o3*c
o3x3o3o *b3o3*c
o3o3o3o *b3x3*c

*) Although being rescalable to equal edge lengths, those figures are only scaliform.

Additionally a still hypercompact lamina-truncate uniform is known here, the lamina-truncate( x3x4x3x8o ).

Although o4x4o3*a4x4o3*a is itself not quasiregular it still allows for rectification as well as truncation, as all of its faces are alike squares. Because additionally its edges had been symmetry equivalent, it becomes clear that thoss then result in still hypercompact scaliform tetracombs rect( o4x4o3*a4x4o3*a ) and trunc( o4x4o3*a4x4o3*a ) respectively, which then not only incorporate Johnson solids for (some of its) cells, but also have an in turn truely scaliform facet type.

----
 5D  Pentacombs (up)
----

Just providing irreducible convex symmetries. In class 1 (compact ones) there is none.

Paracompact Pentacombs

In class 2 we have only the groups

o3o3o3o4o3o
o3o3o4o3o3o
o3o4o3o3o4o

o3o3o *b3o4o3o
o3o3o3o4o *c3o
o4o3o3o4o *c3o

o3o3o *b3o *b3o3o
o3o3o *b3o *b3o4o

o3o3o *b3o *b3o *b3o

o3o3o3o3o3o3*b

o3o3o3o3o3o4*a
o3o3o4o3o3o4*a

x3o3o3o4o3o
o3o3o3o4o3x
...
o3o3o3o4s3s

x3o3o4o3o3o
o3x3o4o3o3o
...

o3o4o3x3o4o
x3x4o3o3o4o
...
s3s4o3o3o4o

x3o3o3o4o *c3o
...

o4o3o3o4o *c3x
o4o3x3o4o *c3o
...

x3o3o *b3o *b3o3o
o3x3o *b3o *b3o3o
o3o3o *b3o *b3o3x
...

x3o3o *b3o *b3o *b3o
o3x3o *b3o *b3o *b3o
...

Hypercompact Pentacombs

Again those clearly have infinite count. So there cannot be a complete listing, not even tentatively. Only a few, randomly selected examples might follow here.

x3x4o3o4o3o
s3s4o3o4o3o

----
 6D  Hexacombs (up)
----

Just providing irreducible convex symmetries. In class 1 (compact ones) there is none.

Paracompact Hexacombs

In class 2 we have only the groups

o3o3o3o3o4o *c3o

o3o3o3o3o *b3o *c3o

o3o3o3o3o3o3o3*b

Hypercompact Hexacombs

Again those clearly have infinite count. So there cannot be a complete listing, not even tentatively. Only a few, randomly selected examples might follow here.

x3o3o3o3o4o3o

o3o3o3o4o3o3x

x3o3o3o3o *b3o *b3o
o3x3o3o3o *b3o *b3o
o3o3o3o3x *b3o *b3o

x3o3o *b3o *b3o *b3o *b3o

----
 7D  Heptacombs (up)
----

Just providing irreducible convex symmetries. In class 1 (compact ones) there is none.

Paracompact Heptacombs

In class 2 we have only the groups

o3o3o3o3o3o *c3o3o
o3o3o3o3o3o4o *c3o

o3o3o3o3o3o *b3o *d3o

o3o3o3o3o3o3o3o3*b

x3o3o3o3o3o *c3o3o
o3o3x3o3o3o *c3o3o
o3o3o3o3o3x *c3o3o

----
 8D  Octacombs (up)
----

Just providing irreducible convex symmetries. In class 1 (compact ones) there is none.

Paracompact Octacombs

In class 2 we have only the groups

o3o3o3o3o3o3o3o *d3o
o3o3o3o3o3o3o4o *c3o

o3o3o3o3o3o3o *b3o *e3o

o3o3o3o3o3o3o3o3o3*b

x3o3o3o3o3o3o3o *d3o
o3o3o3o3o3o3o3x *d3o
o3o3o3o3o3o3o3o *d3x

Hypercompact Octacombs

Again those clearly have infinite count. So there cannot be a complete listing, not even tentatively. Only a few, randomly selected examples might follow here.

x3o3o3o3o3o3o *c3o3o
o3o3o3o3o3o3x *c3o3o

----
 9D  Enneacombs (up)
----

Just providing irreducible convex symmetries. In class 1 (compact ones) there is none.

Paracompact Enneacombs

In class 2 we have only the groups

o3o3o3o3o3o3o3o3o *c3o
o3o3o3o3o3o3o3o4o *c3o

o3o3o3o3o3o3o3o *b3o *f3o

x3o3o3o3o3o3o3o3o *c3o
o3o3o3o3o3o3o3o3x *c3o
o3o3o3o3o3o3o3o3o *c3x

Hypercompact Enneacombs

Again those clearly have infinite count. So there cannot be a complete listing, not even tentatively. Only a few, randomly selected examples might follow here.

x3o3o3o3o3o3o3o3o *d3o
o3o3o3o3o3o3o3o3x *d3o
o3o3o3o3o3o3o3o3o *d3x