|
Acronym
|
prissi
|
|
Name
|
prismatorhombisnub icositetrachoron,
prismato-semistruncated icositetrachoron,
runcic snub icositetrachoron
|
|
|
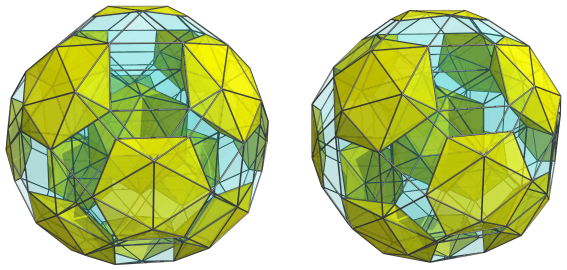 © ©
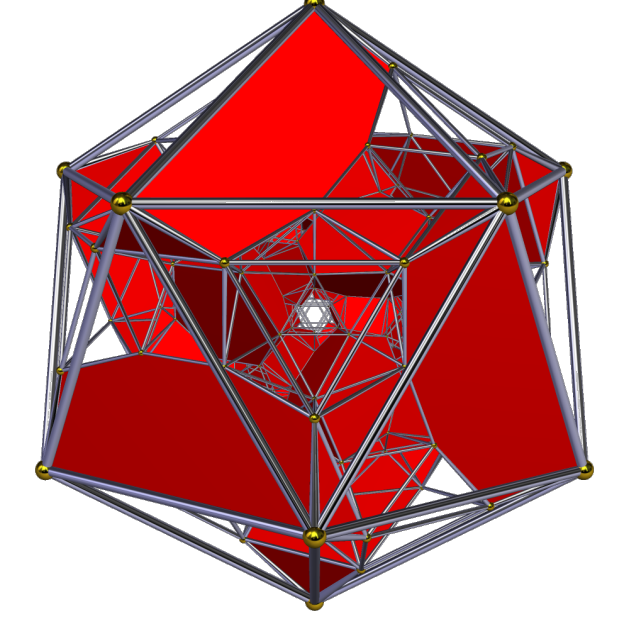 © © 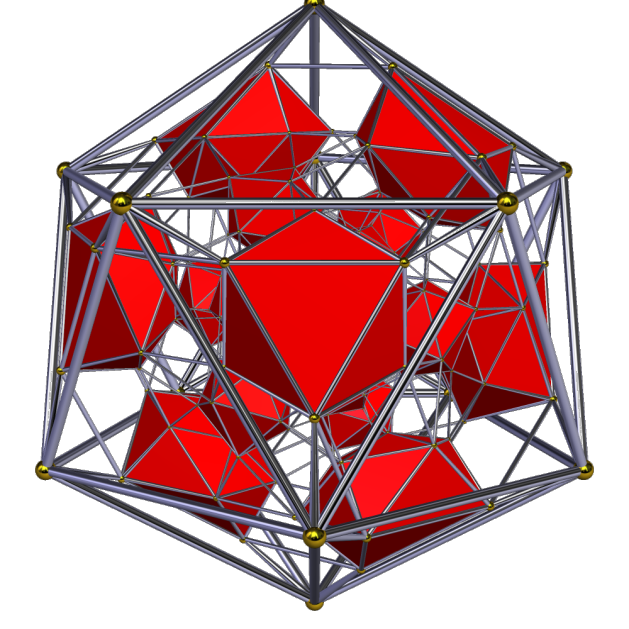 © ©
|
|
Cross sections
|
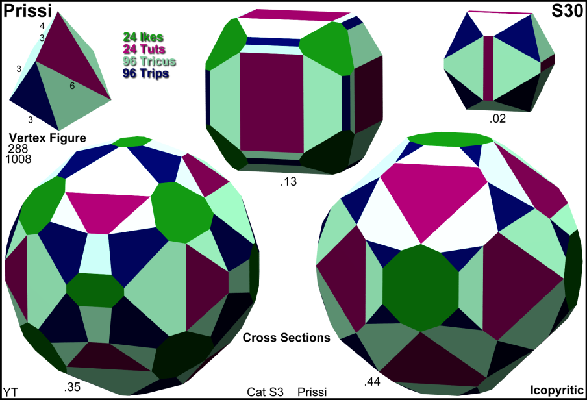 © ©
|
|
Circumradius
|
sqrt[4+sqrt(5)] = 2.497212
|
|
Vertex figure
|
 © ©
|
|
Coordinates
|
-
(0, 1/2, (1+sqrt(5))/4, (7+sqrt(5))/4) & all even permutations, all changes of sign
-
(1/2, 1, (3+sqrt(5))/4, (5+sqrt(5))/4) & all even permutations, all changes of sign
|
|
Dihedral angles
|
- at {6} between tricu and tut: arccos[-(1+3 sqrt(5))/8] = 164.477512°
- at {4} between tricu and trip: arccos[-sqrt(5/6)] = 155.905157°
- at {3} between ike and trip: 150°
- at {3} between ike and tricu: arccos[-sqrt(5/8)] = 142.238756°
- at {3} between tricu and tut: arccos[-(3 sqrt(5)-1)/8] = 135.522488°
|
|
Face vector
|
288, 1008, 960, 240
|
|
Confer
|
- more general:
-
aoc3ddd3cao *b3oca&#ze
- uniform relatives:
-
sadi
ico
- related CRFs:
-
icau prissi
- related scaliforms:
-
s3s6o3x
- general polytopal classes:
-
scaliform
|
External
links
|




|
As abstract polytope prissi is isomorphic to prarsi,
thereby replacing prograde squares by retrogrades, respectively
ike by gike.
This is a scaliform polychoron, first found (in 2005 by Klitzing) as a variation of a faceting
of the prismatorhombated icositetrachoron (prico).
The mere faceting of prico however could be described as qoQ3xxx3Qqo *b3oQq&#zh.
It further can be obtained as a Stott addition of the snub icositetrachoron (sadi) with the dually
oriented icositetrachoron (ico), resulting in shifting formerly face-connected icosahedra (ike)
one edge length appart.
Moreover it can be obtained by chopping off all but 288 vertices of padohi.
This could be seen best from the vertex figure of padohi: vo5ox&#q, of which the vertex figure of prissi is a faceting.
Prissi can also be considered as the double application of an alternated faceting according to
x3x4x3x (gippic) → x3x4s3s (prico) = x3x4o3x → s'3s'4s3s = s3s4o3x.
(Because of the intrinsic symmetry of the symbol x3x4x3x, the application order (s first or s' first) obviously is irrelevant.)
Conversely an according full-symmetrical blend of 25 such prissies (5 per vertex)
wrt. that padohi relation,
using modular 2 reductions and blending in turn the co-realmic tricues into ohos, would result in
sad phiddix, a member of the former's regiment.
Incidence matrix according to Dynkin symbol
s3s4o3x
demi( . . . . ) | 288 | 2 1 4 | 1 2 3 4 2 | 1 2 1 3
----------------+-----+-------------+-------------------+------------
demi( . . . x ) | 2 | 288 * * | 1 0 0 2 1 | 0 1 1 2
. s4o . | 2 | * 144 * | 0 0 2 0 2 | 1 0 1 2
sefa( s3s . . ) | 2 | * * 576 | 0 1 1 1 0 | 1 1 0 1
----------------+-----+-------------+-------------------+------------
demi( . . o3x ) | 3 | 3 0 0 | 96 * * * * | 0 0 1 1
s3s . . ♦ 3 | 0 0 3 | * 192 * * * | 1 1 0 0
sefa( s3s4o . ) | 3 | 0 1 2 | * * 288 * * | 1 0 0 1
sefa( s3s 2 x ) | 4 | 2 0 2 | * * * 288 * | 0 1 0 1
sefa( . s4o3x ) | 6 | 3 3 0 | * * * * 96 | 0 0 1 1
----------------+-----+-------------+-------------------+------------
s3s4o . ♦ 12 | 0 6 24 | 0 8 12 0 0 | 24 * * *
s3s 2 x ♦ 6 | 3 0 6 | 0 2 0 3 0 | * 96 * *
. s4o3x ♦ 12 | 12 6 0 | 4 0 0 0 4 | * * 24 *
sefa( s3s4o3x ) ♦ 9 | 6 3 6 | 1 0 3 3 1 | * * * 96
starting figure: x3x4o3x
fox3xxx3xfo *b3oxf&#zx → height = 0
o..3o..3o.. *b3o.. | 96 * * | 2 1 2 2 0 0 0 0 0 | 2 1 2 1 2 2 2 0 0 0 0 0 0 | 1 1 2 1 2 0 0 0
.o.3.o.3.o. *b3.o. | * 96 * | 0 0 2 0 2 1 2 0 0 | 0 0 2 2 0 0 2 1 2 1 2 0 0 | 0 2 0 1 2 1 1 0
..o3..o3..o *b3..o | * * 96 | 0 0 0 2 0 0 2 1 2 | 0 0 0 0 2 1 2 0 0 2 2 2 1 | 0 0 1 1 2 0 2 1
-----------------------+----------+-------------------------------+-----------------------------------------+---------------------
... x.. ... ... | 2 0 0 | 96 * * * * * * * * | 1 1 1 0 1 0 0 0 0 0 0 0 0 | 1 1 1 0 1 0 0 0
... ... x.. ... | 2 0 0 | * 48 * * * * * * * | 2 0 0 0 0 2 0 0 0 0 0 0 0 | 1 0 2 1 0 0 0 0
oo.3oo.3oo. *b3oo.&#x | 1 1 0 | * * 192 * * * * * * | 0 0 1 1 0 0 1 0 0 0 0 0 0 | 0 1 0 1 1 0 0 0
o.o3o.o3o.o *b3o.o&#x | 1 0 1 | * * * 192 * * * * * | 0 0 0 0 1 1 1 0 0 0 0 0 0 | 0 0 1 1 1 0 0 0
... .x. ... ... | 0 2 0 | * * * * 96 * * * * | 0 0 1 0 0 0 0 1 1 0 1 0 0 | 0 1 0 0 1 1 1 0
... ... ... .x. | 0 2 0 | * * * * * 48 * * * | 0 0 0 2 0 0 0 0 2 0 0 0 0 | 0 2 0 1 0 1 0 0
.oo3.oo3.oo *b3.oo&#x | 0 1 1 | * * * * * * 192 * * | 0 0 0 0 0 0 1 0 0 1 1 0 0 | 0 0 0 1 1 0 1 0
..x ... ... ... | 0 0 2 | * * * * * * * 48 * | 0 0 0 0 0 0 0 0 0 2 0 2 0 | 0 0 0 1 0 0 2 1
... ..x ... ... | 0 0 2 | * * * * * * * * 96 | 0 0 0 0 1 0 0 0 0 0 1 1 1 | 0 0 1 0 1 0 1 1
-----------------------+----------+-------------------------------+-----------------------------------------+---------------------
... x..3x.. ... | 6 0 0 | 3 3 0 0 0 0 0 0 0 | 32 * * * * * * * * * * * * | 1 0 1 0 0 0 0 0
... x.. ... *b3o.. | 3 0 0 | 3 0 0 0 0 0 0 0 0 | * 32 * * * * * * * * * * * | 1 1 0 0 0 0 0 0
... xx. ... ...&#x | 2 2 0 | 1 0 2 0 1 0 0 0 0 | * * 96 * * * * * * * * * * | 0 1 0 0 1 0 0 0
... ... ... ox.&#x | 1 2 0 | 0 0 2 0 0 1 0 0 0 | * * * 96 * * * * * * * * * | 0 1 0 1 0 0 0 0
... x.x ... ...&#x | 2 0 2 | 1 0 0 2 0 0 0 0 1 | * * * * 96 * * * * * * * * | 0 0 1 0 1 0 0 0
... ... x.o ...&#x | 2 0 1 | 0 1 0 2 0 0 0 0 0 | * * * * * 96 * * * * * * * | 0 0 1 1 0 0 0 0
ooo3ooo3ooo *b3ooo&#x | 1 1 1 | 0 0 1 1 0 0 1 0 0 | * * * * * * 192 * * * * * * | 0 0 0 1 1 0 0 0
.o.3.x. ... ... | 0 3 0 | 0 0 0 0 3 0 0 0 0 | * * * * * * * 32 * * * * * | 0 0 0 0 0 1 1 0
... .x. ... *b3.x. | 0 6 0 | 0 0 0 0 3 3 0 0 0 | * * * * * * * * 32 * * * * | 0 1 0 0 0 1 0 0
.ox ... ... ...&#x | 0 1 2 | 0 0 0 0 0 0 2 1 0 | * * * * * * * * * 96 * * * | 0 0 0 1 0 0 1 0
... .xx ... ...&#x | 0 2 2 | 0 0 0 0 1 0 2 0 1 | * * * * * * * * * * 96 * * | 0 0 0 0 1 0 1 0
..x3..x ... ... | 0 0 6 | 0 0 0 0 0 0 0 3 3 | * * * * * * * * * * * 32 * | 0 0 0 0 0 0 1 1
... ..x3..o ... | 0 0 3 | 0 0 0 0 0 0 0 0 3 | * * * * * * * * * * * * 32 | 0 0 1 0 0 0 0 1
-----------------------+----------+-------------------------------+-----------------------------------------+---------------------
... x..3x.. *b3o.. ♦ 12 0 0 | 12 6 0 0 0 0 0 0 0 | 4 4 0 0 0 0 0 0 0 0 0 0 0 | 8 * * * * * * *
... xx. ... *b3ox.&#x ♦ 3 6 0 | 3 0 6 0 3 3 0 0 0 | 0 1 3 3 0 0 0 0 1 0 0 0 0 | * 32 * * * * * *
... x.x3x.o ...&#x ♦ 6 0 3 | 3 3 0 6 0 0 0 0 3 | 1 0 0 0 3 3 0 0 0 0 0 0 1 | * * 32 * * * * *
fox ... xfo oxf&#zx ♦ 4 4 4 | 0 2 8 8 0 2 8 2 0 | 0 0 0 4 0 4 8 0 0 4 0 0 0 | * * * 24 * * * *
... xxx ... ...&#x ♦ 2 2 2 | 1 0 2 2 1 0 2 0 1 | 0 0 1 0 1 0 2 0 0 0 1 0 0 | * * * * 96 * * *
.o.3.x. ... *b3.x. ♦ 0 12 0 | 0 0 0 0 12 6 0 0 0 | 0 0 0 0 0 0 0 4 4 0 0 0 0 | * * * * * 8 * *
.ox3.xx ... ...&#x ♦ 0 3 6 | 0 0 0 0 3 0 6 3 3 | 0 0 0 0 0 0 0 1 0 3 3 1 0 | * * * * * * 32 *
..x3..x3..o ... ♦ 0 0 12 | 0 0 0 0 0 0 0 6 12 | 0 0 0 0 0 0 0 0 0 0 0 4 4 | * * * * * * * 8
©
©
©
©
©


