Acronym	gohi, gpD
Name	great hecatonicosachoron, greatened polydodecahedron
Cross sections	©
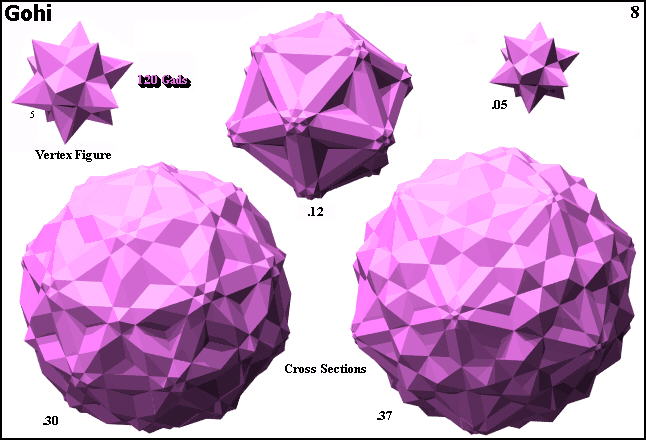Circumradius	(1+sqrt(5))/2 = 1.618034
Inradius	(3+sqrt(5))/4 = 1.309017
Density	6
Coordinates	(τ, 0, 0, 0)             & all permutations, all changes of sign (vertex inscribed f/q-hex) (τ/2, τ/2, τ/2, τ/2)   & all permutations, all changes of sign (vertex inscribed f-tes) (τ2/2, τ/2, 1/2, 0)   & even permutations, all changes of sign (vertex inscribed sadi) where τ = (1+sqrt(5))/2 (a. and b. together define a vertex inscribed f-ico)
General of army	ex
Colonel of regiment	ex
Dual	(selfdual)
Dihedral angles	at {5} between gad and gad:   144°
Face vector	120, 720, 720, 120
Confer	Grünbaumian relatives: gahi+gohi   ex+gohi+120id   2gohi   related segmentochora: sissidpy   general polytopal classes: Wythoffian polychora   regular   noble polytopes
External links

As abstract polytope gohi is isomorphic to gashi, thereby replacing gad by sissid, resp. replacing pentagonal faces and edge figures each by pentagrammal ones, resp. replacing sissid vertex figures by gad ones.

Its vertex pyramid is an f-scaled sissidpy.

Incidence matrix according to Dynkin symbol

x5o5/2o5o

. .   . . | 120 ♦  12 |  30 |  12
----------+-----+-----+-----+----
x .   . . |   2 | 720 |   5 |   5
----------+-----+-----+-----+----
x5o   . . |   5 |   5 | 720 |   2
----------+-----+-----+-----+----
x5o5/2o . ♦  12 |  30 |  12 | 120

snubbed forms: β5o5/2o5o

x5o5/2o5/4o

. .   .   . | 120 ♦  12 |  30 |  12
------------+-----+-----+-----+----
x .   .   . |   2 | 720 |   5 |   5
------------+-----+-----+-----+----
x5o   .   . |   5 |   5 | 720 |   2
------------+-----+-----+-----+----
x5o5/2o   . ♦  12 |  30 |  12 | 120

x5o5/3o5o

. .   . . | 120 ♦  12 |  30 |  12
----------+-----+-----+-----+----
x .   . . |   2 | 720 |   5 |   5
----------+-----+-----+-----+----
x5o   . . |   5 |   5 | 720 |   2
----------+-----+-----+-----+----
x5o5/3o . ♦  12 |  30 |  12 | 120

x5o5/3o5/4o

. .   .   . | 120 ♦  12 |  30 |  12
------------+-----+-----+-----+----
x .   .   . |   2 | 720 |   5 |   5
------------+-----+-----+-----+----
x5o   .   . |   5 |   5 | 720 |   2
------------+-----+-----+-----+----
x5o5/3o   . ♦  12 |  30 |  12 | 120

x5/4o5/2o5o

.   .   . . | 120 ♦  12 |  30 |  12
------------+-----+-----+-----+----
x   .   . . |   2 | 720 |   5 |   5
------------+-----+-----+-----+----
x5/4o   . . |   5 |   5 | 720 |   2
------------+-----+-----+-----+----
x5/4o5/2o . ♦  12 |  30 |  12 | 120

x5/4o5/2o5/4o

.   .   .   . | 120 ♦  12 |  30 |  12
--------------+-----+-----+-----+----
x   .   .   . |   2 | 720 |   5 |   5
--------------+-----+-----+-----+----
x5/4o   .   . |   5 |   5 | 720 |   2
--------------+-----+-----+-----+----
x5/4o5/2o   . ♦  12 |  30 |  12 | 120

x5/4o5/3o5o

.   .   . . | 120 ♦  12 |  30 |  12
------------+-----+-----+-----+----
x   .   . . |   2 | 720 |   5 |   5
------------+-----+-----+-----+----
x5/4o   . . |   5 |   5 | 720 |   2
------------+-----+-----+-----+----
x5/4o5/3o . ♦  12 |  30 |  12 | 120

x5/4o5/3o5/4o

.   .   .   . | 120 ♦  12 |  30 |  12
--------------+-----+-----+-----+----
x   .   .   . |   2 | 720 |   5 |   5
--------------+-----+-----+-----+----
x5/4o   .   . |   5 |   5 | 720 |   2
--------------+-----+-----+-----+----
x5/4o5/3o   . ♦  12 |  30 |  12 | 120