trat

Acronym trat (subsym.: snitititat)

Name triangular tiling,
Delone complex of hexagonal lattice A₂


regular	2-coloring of triangles	©	3-coloring of vertices	6-coloring of triangles		4-coloring of triangles	©

Vertex figure [3⁶]

Vertex layers
(first ones only)

Layer	Symmetry	Subsymmetries
	`o3o6o`	`o3o .`	`. o6o`
1	`x3o6o`	`x3o .` {3} first	`. o6o` vertex first
2		`o3u .`	`. x6o` vertex figure
3		`u3x .`	`. o6h`
4		`x3d .`	`. u6o`
...		...	...

(d = u+x = 3x)

General of army (is itself convex)

Colonel of regiment (is itself locally convex – other uniform tiling member: ditatha )

Dual hexat

Confer

uniform relative:: that hexat ditatha
related CRF tilings:: pextrat pacrothat uBxx3xoAo3xooA3*a&#zx uBxx3uxBx3xooA3*a&#zx
related rhomb tiling:: rhombic xAoo3xoAo3xooA3*a&#zx
ambification:: that
general polytopal classes:: partial Stott expansions regular noble polytopes

External
links

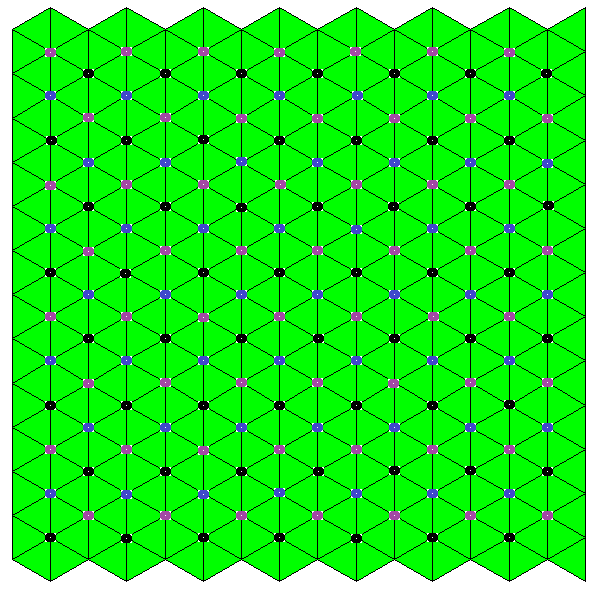
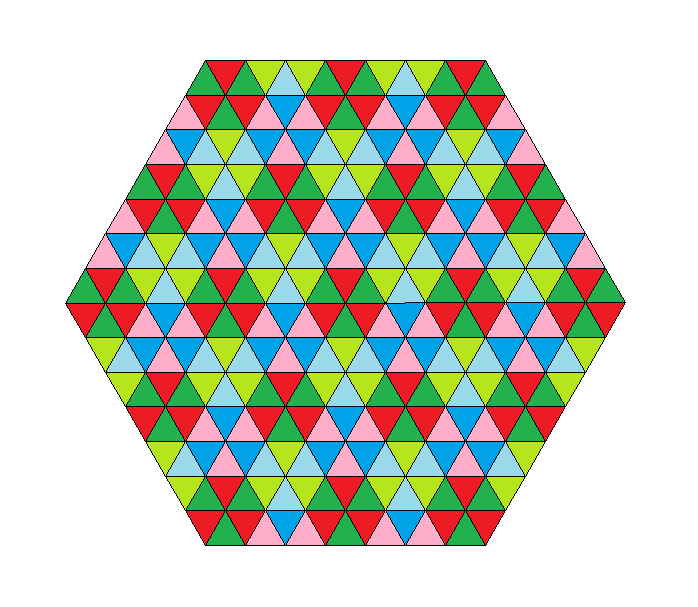
The 3-coloring of the vertices allows for partial Stott expansions running through pextrat and pacrothat finally towards rothat.

This tiling also could be considered as a vertex overlay of hexat plus 3 shifted copies of trats with edge size 3 (see below, resp. the above 6-coloring of triangles). Then this decomposition allows for a further 3-step transformation running through uBxx3xoAo3xooA3*a&#zx and uBxx3uxBx3xooA3*a&#zx towards a pure hexat (A = d = 3x, B = 4x). – So this transformation sequence is not a true partial Stott expansion one because pairs of triangles become transformed into single hexagons. But this could be recovered by combining these pairs into rhombs. The corresponding sequence member here then would be rhomb-xAoo3xoAo3xooA3*a&#zx.

The 4-coloring of the faces is still uniform, in fact it could be considered as snub tritritriangular tiling (snitititat), s3s3s3*a.

Incidence matrix according to Dynkin symbol

x3o6o   (N → ∞)

. . . | N |  6 |  6
------+---+----+---
x . . | 2 | 3N |  2
------+---+----+---
x3o . | 3 |  3 | 2N

x3o3o3*a    (N → ∞)

. . .    | N |  6 | 3 3
---------+---+----+----
x . .    | 2 | 3N | 1 1
---------+---+----+----
x3o .    | 3 |  3 | N *
x . o3*a | 3 |  3 | * N

x3o6/5o   (N → ∞)

. .   . | N |  6 |  6
--------+---+----+---
x .   . | 2 | 3N |  2
--------+---+----+---
x3o   . | 3 |  3 | 2N

x3/2o6o   (N → ∞)

.   . . | N |  6 |  6
--------+---+----+---
x   . . | 2 | 3N |  2
--------+---+----+---
x3/2o . | 3 |  3 | 2N

x3/2o6/5o   (N → ∞)

.   .   . | N |  6 |  6
----------+---+----+---
x   .   . | 2 | 3N |  2
----------+---+----+---
x3/2o   . | 3 |  3 | 2N

x3/2o3/2o3*a    (N → ∞)

.   .   .    | N |  6 | 3 3
-------------+---+----+----
x   .   .    | 2 | 3N | 1 1
-------------+---+----+----
x3/2o   .    | 3 |  3 | N *
x   .   o3*a | 3 |  3 | * N

o3/2x3/2o3*a    (N → ∞)

.   .   .    | N |  6 | 3 3
-------------+---+----+----
.   x   .    | 2 | 3N | 1 1
-------------+---+----+----
o3/2x   .    | 3 |  3 | N *
.   x3/2o .  | 3 |  3 | * N

s6o3o    (N → ∞)

demi( . . . ) | N |  6 | 3 3
--------------+---+----+----
sefa( s6o . ) | 2 | 3N | 1 1
--------------+---+----+----
      s6o .   ♦ 3 |  3 | N *
sefa( s6o3o ) | 3 |  3 | * N

starting figure: x6o3o

s3s6o    (N → ∞)

demi( . . . ) | 3N |  4  2 |  2 1  3
--------------+----+-------+--------
sefa( s3s . ) |  2 | 6N  * |  1 0  1
sefa( . s6o ) |  2 |  * 3N |  0 1  1
--------------+----+-------+--------
      s3s .   ♦  3 |  3  0 | 2N *  *
      . s6o   ♦  3 |  0  3 |  * N  *
sefa( s3s6o ) |  3 |  2  1 |  * * 3N

starting figure: x3x6o

s3s3s3*a   (N → ∞)

demi( . . .    ) | 3N |  2  2  2 | 1 1 1  3
-----------------+----+----------+---------
sefa( s3s .    ) |  2 | 3N  *  * | 1 0 0  1
sefa( s . s3*a ) |  2 |  * 3N  * | 0 1 0  1
sefa( . s3s    ) |  2 |  *  * 3N | 0 0 1  1
-----------------+----+----------+---------
      s3s .      ♦  3 |  3  0  0 | N * *  *
      s . s3*a   ♦  3 |  0  3  0 | * N *  *
      . s3s      ♦  3 |  0  0  3 | * * N  *
sefa( s3s3s3*a ) |  3 |  1  1  1 | * * * 3N

starting figure: x3x3x3*a

xdoo3xodo3xood3*a&#zx   (N → ∞, d = 3x)

o...3o...3o...3*a    | 6N * * * |  1  1  1  1  1  1 |  1  1  1  1  1  1
.o..3.o..3.o..3*a    |  * N * * |  0  0  0  6  0  0 |  3  3  0  0  0  0
..o.3..o.3..o.3*a    |  * * N * |  0  0  0  0  6  0 |  0  0  3  3  0  0
...o3...o3...o3*a    |  * * * N |  0  0  0  0  0  6 |  0  0  0  0  3  3
---------------------+----------+-------------------+------------------
x... .... ....       |  2 0 0 0 | 3N  *  *  *  *  * |  0  0  1  0  1  0
.... x... ....       |  2 0 0 0 |  * 3N  *  *  *  * |  1  0  0  0  0  1
.... .... x...       |  2 0 0 0 |  *  * 3N  *  *  * |  0  1  0  1  0  0
oo..3oo..3oo..3*a&#x |  1 1 0 0 |  *  *  * 6N  *  * |  1  1  0  0  0  0
o.o.3o.o.3o.o.3*a&#x |  1 0 1 0 |  *  *  *  * 6N  * |  0  0  1  1  0  0
o..o3o..o3o..o3*a&#x |  1 0 0 1 |  *  *  *  *  * 6N |  0  0  0  0  1  1
---------------------+----------+-------------------+------------------
.... xo.. ....   &#x |  2 1 0 0 |  0  1  0  2  0  0 | 3N  *  *  *  *  *
.... .... xo..   &#x |  2 1 0 0 |  0  0  1  2  0  0 |  * 3N  *  *  *  *
x.o. .... ....   &#x |  2 0 1 0 |  1  0  0  0  2  0 |  *  * 3N  *  *  *
.... .... x.o.   &#x |  2 0 1 0 |  0  0  1  0  2  0 |  *  *  * 3N  *  *
x..o .... ....   &#x |  2 0 0 1 |  1  0  0  0  0  2 |  *  *  *  * 3N  *
.... x..o ....   &#x |  2 0 0 1 |  0  1  0  0  0  2 |  *  *  *  *  * 3N

:xo:∞:ox:&##x   (N → ∞)   → heights = sqrt(3)/2 = 0.866025

 o. ∞ o.     | N * | 2  2 0  2 | 2 1 2 1
 .o ∞ .o     | * N | 0  2 2  2 | 1 2 1 2
-------------+-----+-----------+--------
 x.   ..     | 2 0 | N  * *  * | 1 0 1 0
 oo ∞ oo &#x | 1 1 | * 2N *  * | 1 1 0 0
 ..   .x     | 0 2 | *  * N  * | 0 1 0 1
:oo:∞:oo:&#x | 1 1 | *  * * 2N | 0 0 1 1
-------------+-----+-----------+--------
 xo   .. &#x | 2 1 | 1  2 0  0 | N * * *
 ..   ox &#x | 1 2 | 0  2 1  0 | * N * *
:xo: :..:&#x | 2 1 | 1  0 0  2 | * * N *
:..: :ox:&#x | 1 2 | 0  0 1  2 | * * * N

top of page