doe

Acronym doe

TOCID symbol D

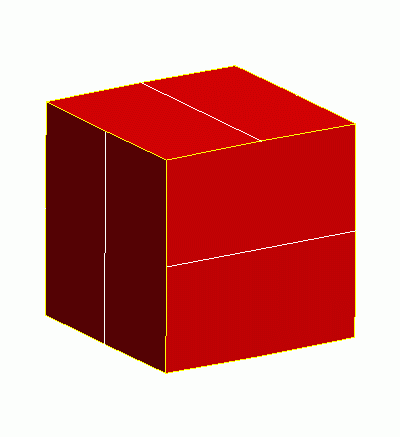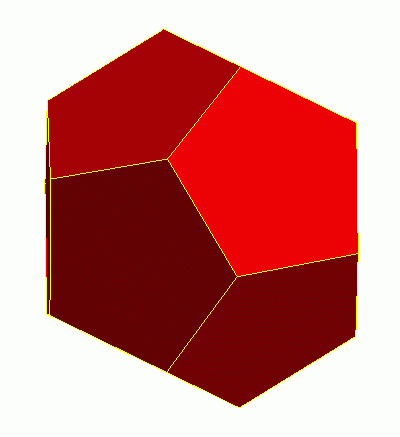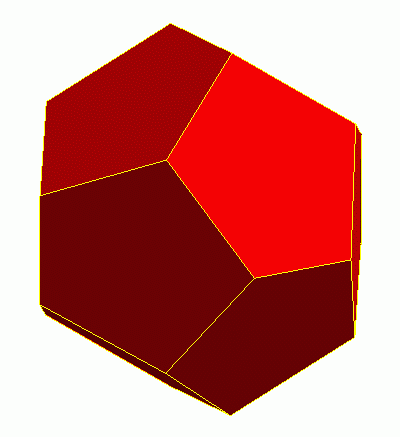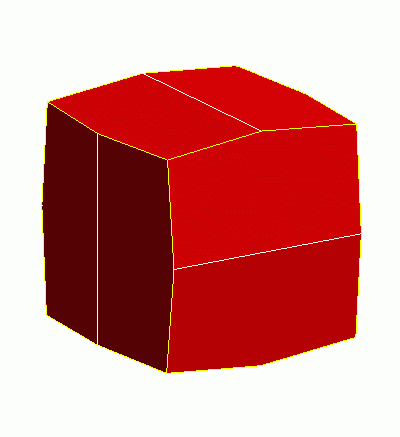
Name dodecahedron,
cosmohedron,
Goldberg polyhedron GP(1,0)

 © ©

Circumradius sqrt[(9+3 sqrt(5))/8] = 1.401259

Edge radius (3+sqrt(5))/4 = 1.309017

Inradius sqrt[(25+11 sqrt(5))/40] = 1.113516

Vertex figure [5³] = f3o

Vertex layers

Layer	Symmetry	Subsymmetries
	`o3o5o`	`o3o .`	`o . o`	`. o5o`
1	`o3o5x`	`o3o .` vertex first	`o . x` edge first	`. o5x` {5} first
2		`o3f .` vertex figure	`f . f`	`. o5f` vertex figure
3		`f3x .`	`F . o`	`. f5o`
4		`x3f .`	`x . F`	`. x5o` opposite {5}
5		`f3o .`	`F . o`
6		`o3o .` opposite vertex	`f . f`
7			`o . x` opposite edge

(F=ff)

Lace city
in approx. ASCII-art

         x         
                   
   f           f   
o                 o
                   
      F     F      
                   
o                 o
   f           f   
                   
         x

Coordinates

(τ/2, τ/2, τ/2) & all permutations, all changes of sign
(vertex inscribed f-cube)
(τ²/2, 1/2, 0) & even permutations, all changes of sign

where τ = (1+sqrt(5))/2

General of army (is itself convex)

Colonel of regiment (is itself locally convex – no other uniform polyhedral members)

Volume (15+7 sqrt(5))/4 = 7.663119

Surface 3 sqrt[25+10 sqrt(5)] = 20.645729

Dual ike

Dihedral angles

between {5} and {5}: arccos(-1/sqrt(5)) = 116.565051°

Face vector 20, 30, 12

Confer

Grünbaumian relatives:: 2doe 3doe 6doe
compounds:: 5 doe comp.
related Johnson solids:: aud pabaud
facetings:: tet-dim doe cube-dim doe ditti
stellations:: p2p5p titdi mibkid cell of gap dual
isogonal relatives:: odsnic
ambification:: id
general polytopal classes:: Wythoffian polyhedra Catalan polyhedra regular noble polytopes

External
links

©

As abstract polytope doe is isomorphic to gissid, thereby replacing pentagons by pentagrams.

The number of ways to color the dodecahedron with different colors per face is 12!/60 = 7 983 360. – This is because the color group is the permutation group of 12 elements and has size 12!, while the order of the pure rotational icosahedral group is 60. (The reflectional icosahedral group would have twice as many, i.e. 120 elements.)

The right picture shows the single celled coastal coccolithophore (phytoplankton) with the latin name Braarudosphaera bigelowii.

Incidence matrix according to Dynkin symbol

o3o5x

. . . | 20 |  3 |  3
------+----+----+---
. . x |  2 | 30 |  2
------+----+----+---
. o5x |  5 |  5 | 12

snubbed forms: o3o5β

o3/2o5x

.   . . | 20 |  3 |  3
--------+----+----+---
.   . x |  2 | 30 |  2
--------+----+----+---
.   o5x |  5 |  5 | 12

x5/4o3o

.   . . | 20 |  3 |  3
--------+----+----+---
x   . . |  2 | 30 |  2
--------+----+----+---
x5/4o . |  5 |  5 | 12

x5/4o3/2o

.   .   . | 20 |  3 |  3
----------+----+----+---
x   .   . |  2 | 30 |  2
----------+----+----+---
x5/4o   . |  5 |  5 | 12

xfoo5oofx&#xt   → outer heights = sqrt[(5+sqrt(5))/10] = 0.850651
                  inner height = sqrt[(5-sqrt(5))/10] = 0.525731
({5} || pseudo f-{5} || dual pseudo f-{5} || dual {5})

o...5o...     | 5 * * * | 2 1  0 0 0 | 1 2 0 0
.o..5.o..     | * 5 * * | 0 1  2 0 0 | 0 2 1 0
..o.5..o.     | * * 5 * | 0 0  2 1 0 | 0 1 2 0
...o5...o     | * * * 5 | 0 0  0 1 2 | 0 0 2 1
--------------+---------+------------+--------
x... ....     | 2 0 0 0 | 5 *  * * * | 1 1 0 0
oo..5oo..&#x  | 1 1 0 0 | * 5  * * * | 0 2 0 0
.oo.5.oo.&#x  | 0 1 1 0 | * * 10 * * | 0 1 1 0
..oo5..oo&#x  | 0 0 1 1 | * *  * 5 * | 0 0 2 0
.... ...x     | 0 0 0 2 | * *  * * 5 | 0 0 1 1
--------------+---------+------------+--------
x...5o...     | 5 0 0 0 | 5 0  0 0 0 | 1 * * *
xfo. ....&#xt | 2 2 1 0 | 1 2  2 0 0 | * 5 * *
.... .ofx&#xt | 0 1 2 2 | 0 0  2 2 1 | * * 5 *
...o5...x     | 0 0 0 5 | 0 0  0 0 5 | * * * 1

or
o...5o...      & | 10  * |  2  1  0 | 1  2
.o..5.o..      & |  * 10 |  0  1  2 | 0  3
-----------------+-------+----------+-----
x... ....      & |  2  0 | 10  *  * | 1  1
oo..5oo..&#x   & |  1  1 |  * 10  * | 0  2
.oo.5.oo.&#x     |  0  2 |  *  * 10 | 0  2
-----------------+-------+----------+-----
x...5o...      & |  5  0 |  5  0  0 | 2  *
xfo. ....&#xt  & |  2  3 |  1  2  2 | * 10

ofxfoo3oofxfo&#xt   → outer heights = sqrt[(3-sqrt(5))/6] = 0.356822
                      tropal heights = 1/sqrt(3) = 0.577350
                      inner height = sqrt[(3+sqrt(5))/6] = 0.934172
(pt || pseudo f-{3} || pseudo (f,x)-{6} || pseudo (x,f)-{6} || pseudo dual f-{3} || pt)

o.....3o.....     | 1 * * * * * | 3 0 0 0 0 0 0 | 3 0 0 0
.o....3.o....     | * 3 * * * * | 1 2 0 0 0 0 0 | 2 1 0 0
..o...3..o...     | * * 6 * * * | 0 1 1 1 0 0 0 | 1 1 1 0
...o..3...o..     | * * * 6 * * | 0 0 0 1 1 1 0 | 0 1 1 1
....o.3....o.     | * * * * 3 * | 0 0 0 0 0 2 1 | 0 0 1 2
.....o3.....o     | * * * * * 1 | 0 0 0 0 0 0 3 | 0 0 0 3
------------------+-------------+---------------+--------
oo....3oo....&#x  | 1 1 0 0 0 0 | 3 * * * * * * | 2 0 0 0
.oo...3.oo...&#x  | 0 1 1 0 0 0 | * 6 * * * * * | 1 1 0 0
..x... ......     | 0 0 2 0 0 0 | * * 3 * * * * | 1 0 1 0
..oo..3..oo..&#x  | 0 0 1 1 0 0 | * * * 6 * * * | 0 1 1 0
...... ...x..     | 0 0 0 2 0 0 | * * * * 3 * * | 0 1 0 1
...oo.3...oo.&#x  | 0 0 0 1 1 0 | * * * * * 6 * | 0 0 1 1
....oo3....oo&#x  | 0 0 0 0 1 1 | * * * * * * 3 | 0 0 0 2
------------------+-------------+---------------+--------
ofx... ......&#xt | 1 2 2 0 0 0 | 2 2 1 0 0 0 0 | 3 * * *
...... .ofx..&#xt | 0 1 2 2 0 0 | 0 2 0 2 1 0 0 | * 3 * *
..xfo. ......&#xt | 0 0 2 2 1 0 | 0 0 1 2 0 2 0 | * * 3 *
...... ...xfo&#xt | 0 0 0 2 2 1 | 0 0 0 0 1 2 2 | * * * 3

or
o.....3o.....      & | 2 *  * | 3  0 0 0 | 3 0
.o....3.o....      & | * 6  * | 1  2 0 0 | 2 1
..o...3..o...      & | * * 12 | 0  1 1 1 | 1 2
---------------------+--------+----------+----
oo....3oo....&#x   & | 1 1  0 | 6  * * * | 2 0
.oo...3.oo...&#x   & | 0 1  1 | * 12 * * | 1 1
..x... ......      & | 0 0  2 | *  * 6 * | 1 1
..oo..3..oo..&#x     | 0 0  2 | *  * * 6 | 0 2
---------------------+--------+----------+----
ofx... ......&#xt  & | 1 2  2 | 2  2 1 0 | 6 *
...... .ofx..&#xt  & | 0 1  4 | 0  2 1 2 | * 6

xfoFofx ofFxFfo&#xt   (F=ff) → height(1,2) = height(3,4) = height(4,5) = height(6,7) = 1/2
                                height(2,3) = height(5,6) = (sqrt(5)-1)/4 = 0.309017
(line || pseudo f-{4} || pseudo ortho ff-line || pseudo (ff,x)-{4} || pseudo ortho ff-line || pseudo f-{4} || line)

o...... o......     | 2 * * * * * * | 1 2 0 0 0 0 0 0 0 0 | 2 1 0 0 0
.o..... .o.....     | * 4 * * * * * | 0 1 1 1 0 0 0 0 0 0 | 1 1 1 0 0
..o.... ..o....     | * * 2 * * * * | 0 0 2 0 1 0 0 0 0 0 | 1 0 2 0 0
...o... ...o...     | * * * 4 * * * | 0 0 0 1 0 1 1 0 0 0 | 0 1 1 1 0
....o.. ....o..     | * * * * 2 * * | 0 0 0 0 1 0 0 2 0 0 | 0 0 2 0 1
.....o. .....o.     | * * * * * 4 * | 0 0 0 0 0 0 1 1 1 0 | 0 0 1 1 1
......o ......o     | * * * * * * 2 | 0 0 0 0 0 0 0 0 2 1 | 0 0 0 1 2
--------------------+---------------+---------------------+----------
x...... .......     | 2 0 0 0 0 0 0 | 1 * * * * * * * * * | 2 0 0 0 0
oo..... oo.....&#x  | 1 1 0 0 0 0 0 | * 4 * * * * * * * * | 1 1 0 0 0
.oo.... .oo....&#x  | 0 1 1 0 0 0 0 | * * 4 * * * * * * * | 1 0 1 0 0
.o.o... .o.o...&#x  | 0 1 0 1 0 0 0 | * * * 4 * * * * * * | 0 1 1 0 0
..o.o.. ..o.o..&#x  | 0 0 1 0 1 0 0 | * * * * 2 * * * * * | 0 0 2 0 0
....... ...x...     | 0 0 0 2 0 0 0 | * * * * * 2 * * * * | 0 1 0 1 0
...o.o. ...o.o.&#x  | 0 0 0 1 0 1 0 | * * * * * * 4 * * * | 0 0 1 1 0
....oo. ....oo.&#x  | 0 0 0 0 1 1 0 | * * * * * * * 4 * * | 0 0 1 0 1
.....oo .....oo&#x  | 0 0 0 0 0 1 1 | * * * * * * * * 4 * | 0 0 0 1 1
......x .......     | 0 0 0 0 0 0 2 | * * * * * * * * * 1 | 0 0 0 0 2
--------------------+---------------+---------------------+----------
xfo.... .......&#xt | 2 2 1 0 0 0 0 | 1 2 2 0 0 0 0 0 0 0 | 2 * * * *
....... of.x...&#xt | 1 2 0 2 0 0 0 | 0 2 0 2 0 1 0 0 0 0 | * 2 * * *
.ooooo. .ooooo.&#xr | 0 1 1 1 1 1 0 | 0 0 1 1 1 0 1 1 0 0 | * * 4 * *  cycle (BCEFD)
....... ...x.fo&#xt | 0 0 0 2 0 2 1 | 0 0 0 0 0 1 2 0 2 0 | * * * 2 *
....ofx .......&#xt | 0 0 0 0 1 2 2 | 0 0 0 0 0 0 0 2 2 1 | * * * * 2

or
o...... o......      & | 4 * * * | 1 2 0 0 0 0 | 2 1 0
.o..... .o.....      & | * 8 * * | 0 1 1 1 0 0 | 1 1 1
..o.... ..o....      & | * * 4 * | 0 0 2 0 1 0 | 1 0 2
...o... ...o...        | * * * 4 | 0 0 0 2 0 1 | 0 2 1
-----------------------+---------+-------------+------
x...... .......      & | 2 0 0 0 | 2 * * * * * | 2 0 0
oo..... oo.....&#x   & | 1 1 0 0 | * 8 * * * * | 1 1 0
.oo.... .oo....&#x   & | 0 1 1 0 | * * 8 * * * | 1 0 1
.o.o... .o.o...&#x   & | 0 1 0 1 | * * * 8 * * | 0 1 1
..o.o.. ..o.o..&#x     | 0 0 2 0 | * * * * 2 * | 0 0 2
....... ...x...        | 0 0 0 2 | * * * * * 2 | 0 2 0
-----------------------+---------+-------------+------
xfo.... .......&#xt  & | 2 2 1 0 | 1 2 2 0 0 0 | 4 * *
....... of.x...&#xt  & | 1 2 0 2 | 0 2 0 2 0 1 | * 4 *
.ooooo. .ooooo.&#xr    | 0 2 2 1 | 0 0 2 2 1 0 | * * 4  cycle (BCEFD)

oxfF xFfo Fofx&#zx   (F=ff) → existing heights = 0
(tegum sum of 3 mutually perp. (x,F)-{4} and an f-cube)

o... o... o...     | 4 * * * | 1 2 0 0 0 0 | 1 2 0
.o.. .o.. .o..     | * 4 * * | 0 0 1 2 0 0 | 2 0 1
..o. ..o. ..o.     | * * 8 * | 0 1 0 1 1 0 | 1 1 1
...o ...o ...o     | * * * 4 | 0 0 0 0 2 1 | 0 1 2
-------------------+---------+-------------+------
.... x... ....     | 2 0 0 0 | 2 * * * * * | 0 2 0
o.o. o.o. o.o.&#x  | 1 0 1 0 | * 8 * * * * | 1 1 0
.x.. .... ....     | 0 2 0 0 | * * 2 * * * | 2 0 0
.oo. .oo. .oo.&#x  | 0 1 1 0 | * * * 8 * * | 1 0 1
..oo ..oo ..oo&#x  | 0 0 1 1 | * * * * 8 * | 0 1 1
.... .... ...x     | 0 0 0 2 | * * * * * 2 | 0 0 2
-------------------+---------+-------------+------
oxf. .... ....&#xt | 1 2 2 0 | 0 2 1 2 0 0 | 4 * * tower: B-C-A
.... x.fo ....&#xt | 2 0 2 1 | 1 2 0 0 2 0 | * 4 *
.... .... .ofx&#xt | 0 1 2 2 | 0 0 0 2 2 1 | * * 4

with inscribed tet subsym. (chiral choice)

4  * * |  3 0  0 |  3 inscribed tet vertices
* 12 * |  1 1  1 |  3 neighbouring vertices (of 3 further tets)
*  * 4 |  0 0  3 |  3 remaining 5th inscribed tet vertices
-------+---------+---
1  1 0 | 12 *  * |  2
0  2 0 |  * 6  * |  2
0  1 1 |  * * 12 |  2
-------+---------+---
1  3 1 |  2 1  2 | 12

top of page