oct

Acronym oct (alt.: trap, tatet)

TOCID symbol O, T T, (3)Q

Name octahedron,
rectified tetrahedron,
tricross (β₃),
tetratetrahedron,
aerochor(id),
trigonal antiprism,
snubbed triangular dihedron,
larger Delone cell of face-centered cubic (fcc) lattice,
equatorial cross-section of (vertex first) 1/q-tes,
vertex figure of hex,
Gosset polytope 0_1,1,
lattice C₃ contact polytope (span of its small roots),
equatorial cross-section of vertex-first hex

©

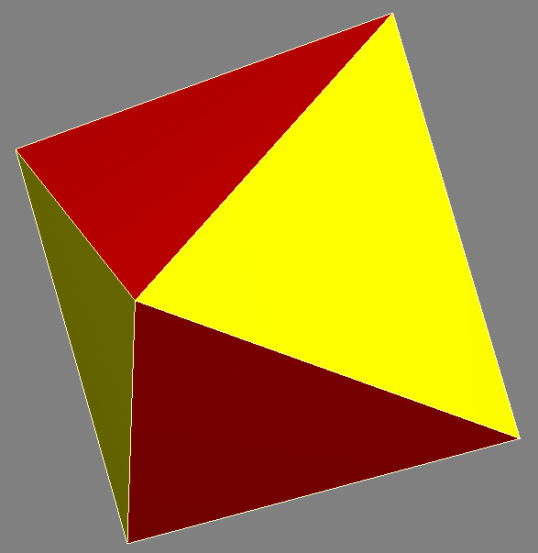VRML

 ⭳ ©

Circumradius 1/sqrt(2) = 0.707107

Edge radius 1/2

Inradius 1/sqrt(6) = 0.408248

Vertex figure [3⁴] = x4o

Snub derivation /
VRML

⭳

Vertex layers

Layer	Symmetry	Subsymmetries
	`o3o4o`	`o3o .`	`o . o`	`. o4o`
1	`x3o4o`	`x3o .` {3} first	`x . o` edge first	`. o4o` vertex first
2		`o3x .` opposite {3}	`o . q`	`. x4o` vertex figure
3			`x . o` opposite edge	`. o4o` opposite vertex
	`o3o3o`	`o3o .`	`o . o`	`. o3o`
1	`o3x3o`	`o3x .` {3} first	`o . o` vertex first	`. x3o` {3} first
2		`x3o .` opposite {3}	`x . x` vertex figure	`. o3x` opposite {3}
3			`o . o` opposite vertex

Lace city
in approx. ASCII-art

©

 x o
o x

©

  o  
o q o
  o

Lace hyper city
in approx. ASCII-art

©

Coordinates (1/sqrt(2), 0, 0) & all permutations, all changes of sign

Volume sqrt(2)/3 = 0.471405

Surface 2 sqrt(3) = 3.464102

Rel. Roundness π sqrt(3)/9 = 60.459979 %

General of army (is itself convex)

Colonel of regiment (is itself locally convex – other uniform polyhedral member: thah – other edge facetings)

Dual cube

Dihedral angles

between {3} and {3}: arccos(-1/3) = 109.471221°

Face vector 6, 12, 8

Confer

more general:: xPo3o...o3o4o
general antiprisms:: n-ap n/2-ap n/d-ap
special bipyramids:: m mNo
variations:: qo3oq&#x xo3ox&#q xo3ox&#h ho3oh&#q squit
Grünbaumian relatives:: oct+6{4} 2oct 2oct+6{4} 2oct+8{3} 2oct+12{4} 4oct
uniform relative:: thah
related Johnson solids:: squippy
related scaliform:: bobipyr
compounds:: se sno daso dissit si gissi addasi dasi oct+4gyro3py
ambification:: co
ambification pre-image:: tet
complex polytopes:: Shephard's generalized oct
general polytopal classes:: Wythoffian polyhedra Catalan polyhedra deltahedra regular noble polytopes orthoplex partial Stott expansions segmentohedra fundamental lace prisms bistratic lace towers lace simplices Coxeter-Elte-Gosset polytopes Hanner polytopes
analogs:: rectified simplex rS_n mid-rectified simplex mrS_n regular orthoplex O_n birectified hypercube brC_n

External
links

The number of ways to color the octahedron with different colors per face is 8!/24 = 1 680. – This is because the color group is the permutation group of 8 elements and has size 8!, while the order of the pure rotational octahedral group is 24. (The reflectional octahedral group would have twice as many, i.e. 48 elements.)

When considered as a trigonal antiprism s s3s (and scaled by h = sqrt(3)), then the prismatic compound with its mirrored copy will have for hull metrically exact the hip-variant q x3x. Stated the other way round, the mere alternating faceting of that variant results in this (scaled) regular shape.

The acronym oct is being used for the full symmetrical (octahedral) variant, trap refers to the trigonal antiprismatic subsymmetry, and tatet refers to the tetratetrahedron, i.e. its tetrahedral subsymmetry.

Being the dual of cube and considering that one's coordinates, it is apparent that this solid is nothing but a ball wrt. the norm |x|+|y|+|z|.

Somehow off-topic there are some neet number relations between the oct and the tet:

face count ratio: F_oct / F_tet = 8/4 = 2
face type: n_oct = n_tet = 3 (triangles each)
radius ratio: (circumradius_oct / inradius_oct)² = circumradius_tet/inradius_tet = 3
volume ratio: V_oct / V_tet = 2

Incidence matrix according to Dynkin symbol

x3o4o

. . . | 6 |  4 | 4
------+---+----+--
x . . | 2 | 12 | 2
------+---+----+--
x3o . | 3 |  3 | 8

snubbed forms: β3o4o

x3/2o4o

.   . . | 6 |  4 | 4
--------+---+----+--
x   . . | 2 | 12 | 2
--------+---+----+--
x3/2o . | 3 |  3 | 8

snubbed forms: β3/2o4o

o4/3o3x

.   . . | 6 |  4 | 4
--------+---+----+--
.   . x | 2 | 12 | 2
--------+---+----+--
.   o3x | 3 |  3 | 8

snubbed forms: o4/3o3β

o4/3o3/2x

.   .   . | 6 |  4 | 4
----------+---+----+--
.   .   x | 2 | 12 | 2
----------+---+----+--
.   o3/2x | 3 |  3 | 8

snubbed forms: o4/3o3/2β

o3x3o

. . . | 6 |  4 | 2 2
------+---+----+----
. x . | 2 | 12 | 1 1
------+---+----+----
o3x . | 3 |  3 | 4 *
. x3o | 3 |  3 | * 4

snubbed forms: o3β3o

o3/2x3o

.   . . | 6 |  4 | 2 2
--------+---+----+----
.   x . | 2 | 12 | 1 1
--------+---+----+----
o3/2x . | 3 |  3 | 4 *
.   x3o | 3 |  3 | * 4

snubbed forms: o3/2β3o

o3/2x3/2o

.   .   . | 6 |  4 | 2 2
----------+---+----+----
.   x   . | 2 | 12 | 1 1
----------+---+----+----
o3/2x   . | 3 |  3 | 4 *
.   x3/2o | 3 |  3 | * 4

snubbed forms: o3/2β3/2o

s2s3s

demi( . . .  ) | 6 | 1 1 2 | 1 3
---------------+---+-------+----
      s2s .    | 2 | 3 * * | 0 2
      s . s2*a | 2 | * 3 * | 0 2
sefa( . s3s  ) | 2 | * * 6 | 1 1
---------------+---+-------+----
      . s3s    ♦ 3 | 0 0 3 | 2 *
sefa( s2s3s  ) | 3 | 1 1 1 | * 6

or
demi( . . . )            | 6 | 2 2 | 1 3
-------------------------+---+-----+----
      s2s .  &  s . s2*a | 2 | 6 * | 0 2
sefa( . s3s )            | 2 | * 6 | 1 1
-------------------------+---+-----+----
      . s3s              ♦ 3 | 0 3 | 2 *
sefa( s2s3s )            | 3 | 2 1 | * 6

starting figure: x x3x

s2s6o

demi( . . . ) | 6 | 2 2 | 1 3
--------------+---+-----+----
      s2s .   | 2 | 6 * | 0 2
sefa( . s6o ) | 2 | * 6 | 1 1
--------------+---+-----+----
      . s6o   ♦ 3 | 0 3 | 2 *
sefa( s2s6o ) | 3 | 2 1 | * 6

starting figure: x x6o

xo3ox&#x   → height = sqrt(2/3) = 0.816497
({3} || dual {3})

o.3o.    | 3 * | 2 2 0 | 1 2 1 0
.o3.o    | * 3 | 0 2 2 | 0 1 2 1
---------+-----+-------+--------
x. ..    | 2 0 | 3 * * | 1 1 0 0
oo3oo&#x | 1 1 | * 6 * | 0 1 1 0
.. .x    | 0 2 | * * 3 | 0 0 1 1
---------+-----+-------+--------
x.3o.    | 3 0 | 3 0 0 | 1 * * *
xo ..&#x | 2 1 | 1 2 0 | * 3 * *
.. ox&#x | 1 2 | 0 2 1 | * * 3 *
.o3.x    | 0 3 | 0 0 3 | * * * 1

oxo4ooo&#xt   → both heights = 1/sqrt(2) = 0.707107
(pt || pseudo {4} || pt)

o..4o..    | 1 * * | 4 0 0 | 4 0
.o.4.o.    | * 4 * | 1 2 1 | 2 2
..o4..o    | * * 1 | 0 0 4 | 0 4
-----------+-------+-------+----
oo.4oo.&#x | 1 1 0 | 4 * * | 2 0
.x. ...    | 0 2 0 | * 4 * | 1 1
.oo4.oo&#x | 0 1 1 | * * 4 | 0 2
-----------+-------+-------+----
ox. ...&#x | 1 2 0 | 2 1 0 | 4 *
.xo ...&#x | 0 2 1 | 0 1 2 | * 4

or
o..4o..    & | 2 * | 4 0 | 4
.o.4.o.      | * 4 | 2 2 | 4
-------------+-----+-----+--
oo.4oo.&#x & | 1 1 | 8 * | 2
.x. ...      | 0 2 | * 4 | 2
-------------+-----+-----+--
ox. ...&#x & | 1 2 | 2 1 | 8

oxo oxo&#xt   → both heights = 1/sqrt(2) = 0.707107
(pt || pseudo {4} || pt)

o.. o..    | 1 * * | 4 0 0 0 | 2 2 0 0
.o. .o.    | * 4 * | 1 1 1 1 | 1 1 1 1
..o ..o    | * * 1 | 0 0 0 4 | 0 0 2 2
-----------+-------+---------+--------
oo. oo.&#x | 1 1 0 | 4 * * * | 1 1 0 0
.x. ...    | 0 2 0 | * 2 * * | 1 0 1 0
... .x.    | 0 2 0 | * * 2 * | 0 1 0 1
.oo .oo&#x | 0 1 1 | * * * 4 | 0 0 1 1
-----------+-------+---------+--------
ox. ...&#x | 1 2 0 | 2 1 0 0 | 2 * * *
... ox.&#x | 1 2 0 | 2 0 1 0 | * 2 * *
.xo ...&#x | 0 2 1 | 0 1 0 2 | * * 2 *
... .xo&#x | 0 2 1 | 0 0 1 2 | * * * 2

or
o.. o..    & | 2 * | 4 0 0 | 2 2
.o. .o.      | * 4 | 2 1 1 | 2 2
-------------+-----+-------+----
oo. oo.&#x & | 1 1 | 8 * * | 1 1
.x. ...      | 0 2 | * 2 * | 2 0
... .x.      | 0 2 | * * 2 | 0 2
-------------+-----+-------+----
ox. ...&#x & | 1 2 | 2 1 0 | 4 *
... ox.&#x & | 1 2 | 2 0 1 | * 4

xox oqo&#xt   → both heights = 1/2
(line || perp pseudo q-line || line)

o.. o..     | 2 * * | 1 2 1 0 0 | 2 2 0
.o. .o.     | * 2 * | 0 2 0 2 0 | 1 2 1
..o ..o     | * * 2 | 0 0 1 2 1 | 0 2 2
------------+-------+-----------+------
x.. ...     | 2 0 0 | 1 * * * * | 2 0 0
oo. oo.&#x  | 1 1 0 | * 4 * * * | 1 1 0
o.o o.o&#x  | 1 0 1 | * * 2 * * | 0 2 0
.oo .oo&#x  | 0 1 1 | * * * 4 * | 0 1 1
..x ...     | 0 0 2 | * * * * 1 | 0 0 2
------------+-------+-----------+------
xo. ...&#x  | 2 1 0 | 1 2 0 0 0 | 2 * *
ooo ooo&#xt | 1 1 1 | 0 1 1 1 0 | * 4 *
.ox ...&#x  | 0 1 2 | 0 0 0 2 1 | * * 2

or
o.. o..     & | 4 * | 1 2 1 | 2 2
.o. .o.       | * 2 | 0 4 0 | 2 2
--------------+-----+-------+----
x.. ...     & | 2 0 | 2 * * | 2 0
oo. oo.&#x  & | 1 1 | * 8 * | 1 1
o.o o.o&#x    | 2 0 | * * 2 | 0 2
--------------+-----+-------+----
xo. ...&#x  & | 2 1 | 1 2 0 | 4 *
ooo ooo&#xt   | 2 1 | 0 2 1 | * 4

oxox&#xr   → all cyclical heights = sqrt(3)/2 = 0.866025
             in fact this lace simplex degenerates into a rhomb with diagonals:
             height(1,3) = sqrt(2) = 1.414214
             height(2,4) = 1

o...    | 1 * * * | 2 2 0 0 0 0 0 | 1 2 1 0 0 0
.o..    | * 2 * * | 1 0 1 1 1 0 0 | 1 1 0 1 1 0
..o.    | * * 1 * | 0 0 0 2 0 2 0 | 0 0 0 1 2 1
...o    | * * * 2 | 0 1 0 0 1 1 1 | 0 1 1 0 1 1
--------+---------+---------------+------------
oo..&#x | 1 1 0 0 | 2 * * * * * * | 1 1 0 0 0 0
o..o&#x | 1 0 0 1 | * 2 * * * * * | 0 1 1 0 0 0
.x..    | 0 2 0 0 | * * 1 * * * * | 1 0 0 1 0 0
.oo.&#x | 0 1 1 0 | * * * 2 * * * | 0 0 0 1 1 0
.o.o&#x | 0 1 0 1 | * * * * 2 * * | 0 1 0 0 1 0
..oo&#x | 0 0 1 1 | * * * * * 2 * | 0 0 0 0 1 1
...x    | 0 0 0 2 | * * * * * * 1 | 0 0 1 0 0 1
--------+---------+---------------+------------
ox..&#x | 1 2 0 0 | 2 0 1 0 0 0 0 | 1 * * * * *
oo.o&#x | 1 1 0 1 | 1 1 0 0 1 0 0 | * 2 * * * *
o..x&#x | 1 0 0 2 | 0 2 0 0 0 0 1 | * * 1 * * *
.xo.&#x | 0 2 1 0 | 0 0 1 2 0 0 0 | * * * 1 * *
.ooo&#x | 0 1 1 1 | 0 0 0 1 1 1 0 | * * * * 2 *
..ox&#x | 0 0 1 2 | 0 0 0 0 0 2 1 | * * * * * 1

qo ox4oo&#zx   → height = 0
(tegum sum of q-line and perp {4})
(tegum product of q-line with {4})

o. o.4o.    | 2 * | 4 0 | 4
.o .o4.o    | * 4 | 2 2 | 4
------------+-----+-----+--
oo oo4oo&#x | 1 1 | 8 * | 2
.. .x ..    | 0 2 | * 4 | 2
------------+-----+-----+--
.. ox ..&#x | 1 2 | 2 1 | 8

qo ox ox&#zx   → height = 0
(tegum sum of q-line and perp {4})
(tegum product of q-line with {4})

o. o. o.    | 2 * | 4 0 0 | 2 2
.o .o .o    | * 4 | 2 1 1 | 2 2
------------+-----+-------+----
oo oo oo&#x | 1 1 | 8 * * | 1 1
.. .x ..    | 0 2 | * 2 * | 2 0
.. .. .x    | 0 2 | * * 2 | 0 2
------------+-----+-------+----
.. ox ..&#x | 1 2 | 2 1 0 | 4 *
.. .. ox&#x | 1 2 | 2 0 1 | * 4

qoo oqo ooq&#zx   → all heights = 0
(tegum sum of 3 perp q-lines)
(tegum product of 3 q-lines)

o.. o.. o..    | 2 * * | 2 2 0 | 4
.o. .o. .o.    | * 2 * | 2 0 2 | 4
..o ..o ..o    | * * 2 | 0 2 2 | 4
---------------+-------+-------+--
oo. oo. oo.&#x | 1 1 0 | 4 * * | 2
o.o o.o o.o&#x | 1 0 1 | * 4 * | 2
.oo .oo .oo&#x | 0 1 1 | * * 4 | 2
---------------+-------+-------+--
ooo ooo ooo&#x | 1 1 1 | 1 1 1 | 8

oooooo&#xr   → all consecutive pairwise heights = all alternating pairwise heights = 1
               Note: these lengths show that this cycle is not flat, rather it is wobbling up and down!

o.....     & | 6 | 2 2 | 3 1
-------------+---+-----+----
oo....&#x  & | 2 | 6 * | 2 0
o.o...&#x  & | 2 | * 6 | 1 1
-------------+---+-----+----
ooo...&#x  & | 3 | 2 1 | 6 *
o.o.o.&#x  & | 3 | 0 3 | * 2

oxxo&#xt   → height(1,2) = height(3,4) = 1/sqrt(12) = 0.288675
             height(2,3) = 1/sqrt(3) = 0.577350
             Note: these lengths show that this tower is not flat, rather it has additional leporello folds!

o...     & | 2 * | 2 2 0 0 | 1 1 2
.o..     & | * 4 | 1 1 1 1 | 1 1 2
-----------+-----+---------+------
oo..&#x  & | 1 1 | 4 * * * | 1 0 1
o.o.&#x  & | 1 1 | * 4 * * | 0 1 1
.x..     & | 0 2 | * * 2 * | 1 1 0
.oo.&#x    | 0 2 | * * * 2 | 0 0 2
-----------+-----+---------+------
ox..&#x  & | 1 2 | 2 0 1 0 | 2 * *
o.x.&#x  & | 1 2 | 0 2 1 0 | * 2 *
ooo.&#x  & | 1 2 | 1 1 0 1 | * * 4

top of page