Acronym	...
Name	(infinite series of) hyperbolic uniform honeycombs with pyritohedral vertex figure
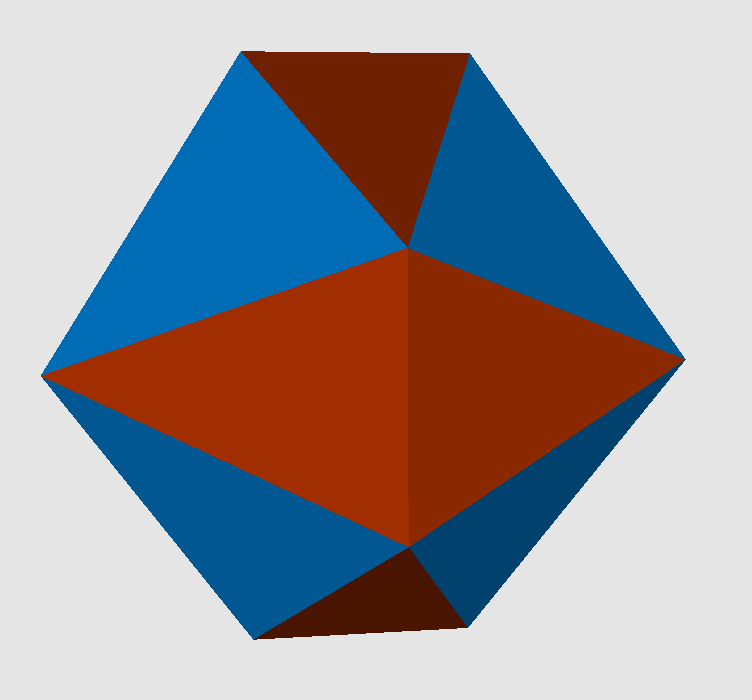
Vertex figure
Especially	chon (P=2)   pechon (P=4)

Columns of P-gonal prisms are aligned at each vertex within 3 mutually perpendicular directions, giving rise for 8 additional these connecting cubes. Accordingly the vertex figure will be snit, i.e. cao2aoc2oca&#zd with a = x(P) and d = x(4) = q (length c there generally is pseudo). As even for P = 3 this amounts for c = (1+sqrt(13))/2 = 2.302776 and thus a circumradius of that vertex figure of sqrt[(9+sqrt(13))/2] = 2.510533, which clealy is larger than 1, all members of this series would belong to hyperbolic geometry only.

In fact, it rather would be the degenerate edge case of P = 2 (with slightly other incidence matrix due to that degeneracy), where this pyritohedral verf becomes a q-scaled oct, i.e. the according honeycomb would become the only then euclidean space regular chon. For the case of P = 4 all cells would become identical cubes and the honeycomb becomes regular once again, in fact it will be pechon.

This series of pyritohedral hyperbolic honeycombs was found in 1997 by W. Krieger.

Incidence matrix according to Dynkin symbol

xPoØxPoØxPoØ*a   (N → ∞)

. . . . . .      | pN ♦  12 |  6  24 | 12  8
-----------------+----+-----+--------+------
x . . . . .    & |  2 | 6pN |  1   4 |  3  2
-----------------+----+-----+--------+------
xPo . . . .    & |  p |   p | 6N   * |  2  0
x . x . . .    & |  4 |   4 |  * 6pN |  1  1
-----------------+----+-----+--------+------
xPo . . x .    & | 2p |  3p |  2   p | 6N  *  P-gonal prism
x . x . x .      |  8 |  12 |  0   6 |  * pN  cube