Acronym	gheat
Name	great heptagonal tiling, hyperbolic order 7/2 heptagon tiling
	©
Circumradius	sqrt[(1-cos2(π/7))/(3-4 cos2(π/7))] = 0.873057 i
Vertex figure	[77]/2
Dual	x7/2o7o
Confer	related tesselations: x3o7o
External links

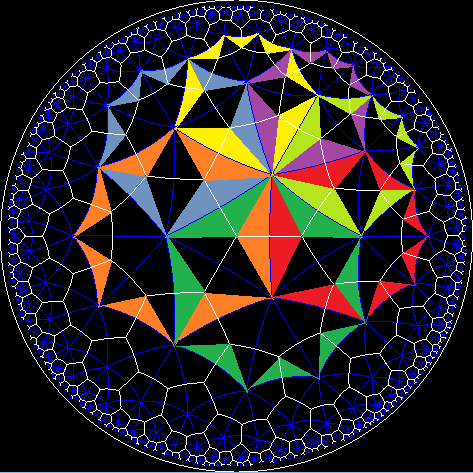
This hyperbolic star tiling can be obtained from x3o7o, when 7 triangles each are joined into one heptagon each. Every part of the tiling will be covered thrice: every triangle of that related tiling would be used in 3 heptagons each.

The right picture, by courtesy of Nan Ma, dynamically shrinks the heptagons, so that those can be spotted easier. – The left one on the other hand uses colorings instead. Even though, the[[:space:]]starry[[:space:]]central regions of those heptagons do belong to them also.

Like x3o7o this tiling allows for the according mod-wrap too, then being nothing but the "great quart = gaqua" faceting. It thence has the same amount of vertices, corresponding to choosing N=12 below.

Incidence matrix according to Dynkin symbol

o7/2o7x   (N → ∞)

.   . . | 2N |  7 |  7
--------+----+----+---
.   . x |  2 | 7N |  2
--------+----+----+---
.   o7x |  7 |  7 | 2N