©
| Site Map | Polytopes | Dynkin Diagrams | Vertex Figures, etc. | Incidence Matrices | Index |
Convex polyhedra using only equilateral triangles for faces are called deltahedra. (Those are known such because of the shape of that upper-case Greek letter.) This kind of restriction could be considered a bit more generally, as well as for higher dimensions. The most general set-up there would be that of homotopal polytopes, being defined as having only a single shape for facets. (Note that isotopal then would add that those are required to be symmetry equivalent as well – which here is not required. However obviously any isotopal polytope would also be homotopal.)
The list given here-below is not meant to be an exhaustive listing. In fact it restricts to homotopal CRFs. – Note: this setup does not require the polytopes generally to be isogonal in addition, i.e. having just a single type of vertices (vertex surroundings / vertex figures), i.e. neither by equivalence nor be symmetry. Polytopes which are both, isogonal and isotopal, commonly are known under the attribute noble (and thus generally would be listed there).
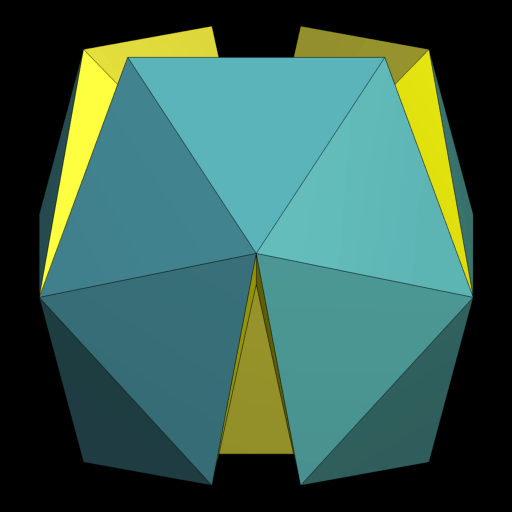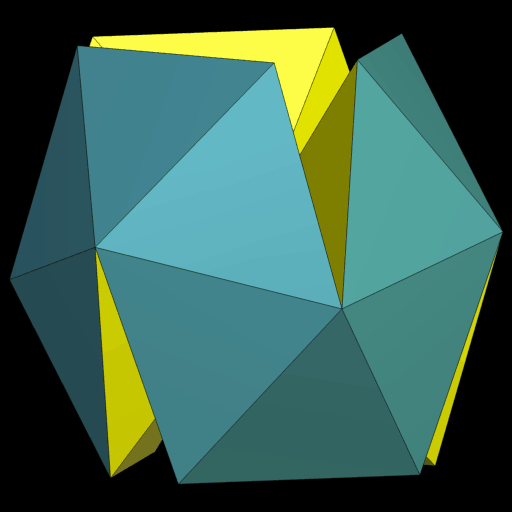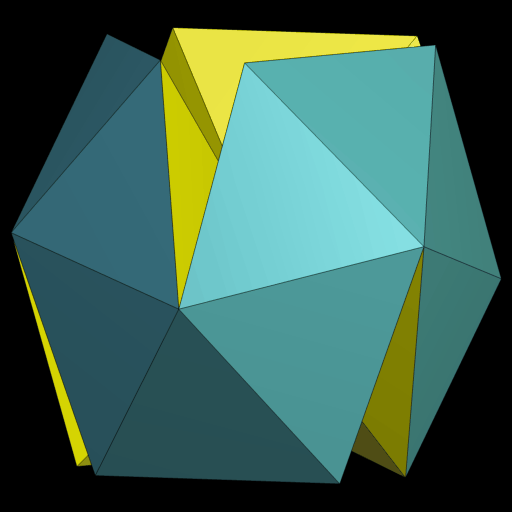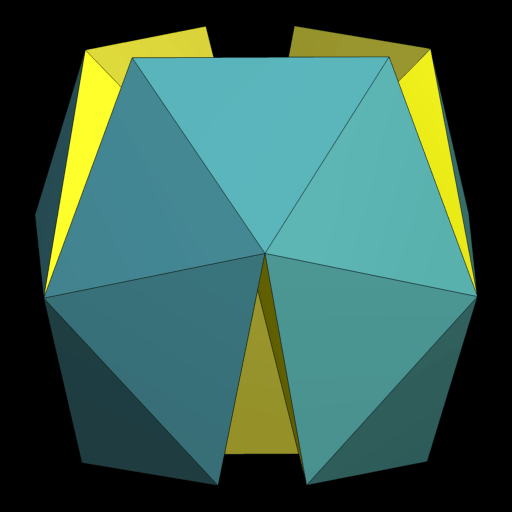
As an aside it should be mentioned however, that already some results for non-convex deltahedra have been obtained. E.g. Rausenberger in 1915 and Cundy in 1952 were initiating a research which Olshevsky in 2006 brought to its avered end: the biform acoptic deltahedra (where acoptic refers to non-convex but still non-intersecting).
The right animated non-convex polyhedra then would be a triform acoptic deltahedron with 36 faces and a triform acoptic deltahedron with 120 faces respectively.
For all simplicial polytopes in general the set of Dehn-Summerville equations can be stated.
---- 3D ----
---- 4D ----
---- 5D ----
---- 6D ----
© 2004-2025 | top of page |