|
Acronym
|
girco
|
|
TOCID symbol
|
tCO
|
|
Name
|
great rhombicuboctahedron (but not querco),
truncated cuboctahedron,
omnitruncated octahedron,
omnitruncated cube
|
|
VRML
|
⭳ ©
|
|
Circumradius
|
sqrt[13+6 sqrt(2)]/2 = 2.317611
|
Inradius
wrt. {4}
|
(3+sqrt(2))/2 = 2.207107
|
Inradius
wrt. {6}
|
sqrt[9+6 sqrt(2)]/2 = 2.090770
|
Inradius
wrt. {8}
|
sqrt(2)+1/2 = 1.914214
|
|
Vertex figure
|
[4,6,8]
|
|
Vertex layers
|
| x3x4x | x3x .
{6} first | x . x
{4} first | . x4x
{8} first |
| x3w . | u . w | . u4x |
| u3w . | x . X | . x4w |
| U3x . | U . w | . x4w |
| x3U . | w . X | . u4x |
| W . x |
| w3u . | w . X | . x4x
opposite {8} |
| W . x |
| w3x . | U . w | |
x3x .
opposite {6} | x . X |
| | u . w |
x . x
opposite{4} |
(X=x+q+q, W=u+w, U=x+w)
|
Lace city
in approx. ASCII-art
|
x w w x
x X X x
w X X w
w X X w
x X X x
x w w x
|
|
Coordinates
|
((1+2 sqrt(2))/2, (1+sqrt(2))/2, 1/2) & all permutations, all changes of sign
|
|
Volume
|
2[11+7 sqrt(2)] = 41.798990
|
|
Surface
|
12[2+sqrt(2)+sqrt(3)] = 61.755172
|
|
General of army
|
(is itself convex)
|
|
Colonel of regiment
|
(is itself locally convex
– no other uniform polyhedral members)
|
|
Dihedral angles
|
- between {4} and {6}: arccos[-sqrt(2/3)] = 144.735610°
- between {4} and {8}: 135°
- between {6} and {8}: arccos[-1/sqrt(3)] = 125.264390°
|
|
Dual
|
m3m4m
|
|
Face vector
|
48, 72, 26
|
|
Confer
|
- decompositions:
-
sirco || girco
- variations:
-
a3b4c
x3x4q
q3x4x
w3x4x
- general polytopal classes:
-
Wythoffian polyhedra
partial Stott expansions
- analogs:
-
omnitruncated hypercube otCn
|
External
links
|






|
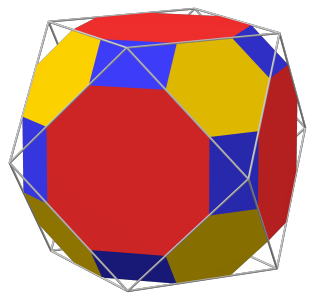
The naming great rhombicuboctahedron derives from the fact that it has faces in the face planes of a rhombidodecahedron, of an cube,
and of a octahedron. As there are 2 such archimedean figures the additional qualifier great versus small is being applied.
Note that the respective scalings of those 3 constituents has to be adopted accordingly each.
When looking more into classes of isogonal variants, then this polyhedron also could be addressed as a truncated cuboctahedron.
However true truncation would not produce squares there. In fact, as shown in the near-right picture, it rather would produce x3t4q instead, where the relative size of t depends on the truncational depth in an inverse ratio.
Note that girco can be thought of as the external blend of
1 sirco + 8 tricues + 6 squacues + 12 cubes,
cf. the far-right Steward toroid K4 \ 8Q3(E4).
This decomposition is also described as the degenerate segmentochoron
xx3ox4xx&#xt.
As abstract polytope girco is isomorphic to quitco, thereby replacing octagons by octagrams.
Incidence matrix according to Dynkin symbol
x3x4x
. . . | 48 | 1 1 1 | 1 1 1
------+----+----------+-------
x . . | 2 | 24 * * | 1 1 0
. x . | 2 | * 24 * | 1 0 1
. . x | 2 | * * 24 | 0 1 1
------+----+----------+-------
x3x . | 6 | 3 3 0 | 8 * *
x . x | 4 | 2 0 2 | * 12 *
. x4x | 8 | 0 4 4 | * * 6
snubbed forms: β3x4x, x3β4x, x3x4s, s3s4x (or as mere faceting xwX wXx Xxw&#zh), β3x4β, x3β4β, s3s4s, β3β4β, s3s4s'
xxwwxx4xuxxux&#xt → height(1,2) = height(2,3) = height(4,5) = height(5,6) = 1/sqrt(2) = 0.707107
height(3,4) = 1
({8} || pseudo (x,u)-{8} || pseudo (w,x)-{8} || pseudo (w,x)-{8} || pseudo (x,u)-{8} || {8})
o.....4o..... | 8 * * * * * | 1 1 1 0 0 0 0 0 0 0 0 0 0 | 1 1 1 0 0 0 0 0
.o....4.o.... | * 8 * * * * | 0 0 1 1 1 0 0 0 0 0 0 0 0 | 0 1 1 1 0 0 0 0
..o...4..o... | * * 8 * * * | 0 0 0 0 1 1 1 0 0 0 0 0 0 | 0 0 1 1 1 0 0 0
...o..4...o.. | * * * 8 * * | 0 0 0 0 0 0 1 1 1 0 0 0 0 | 0 0 0 1 1 1 0 0
....o.4....o. | * * * * 8 * | 0 0 0 0 0 0 0 0 1 1 1 0 0 | 0 0 0 1 0 1 1 0
.....o4.....o | * * * * * 8 | 0 0 0 0 0 0 0 0 0 0 1 1 1 | 0 0 0 0 0 1 1 1
------------------+-------------+---------------------------+----------------
x..... ...... | 2 0 0 0 0 0 | 4 * * * * * * * * * * * * | 1 1 0 0 0 0 0 0
...... x..... | 2 0 0 0 0 0 | * 4 * * * * * * * * * * * | 1 0 1 0 0 0 0 0
oo....4oo....&#x | 1 1 0 0 0 0 | * * 8 * * * * * * * * * * | 0 1 1 0 0 0 0 0
.x.... ...... | 0 2 0 0 0 0 | * * * 4 * * * * * * * * * | 0 1 0 1 0 0 0 0
.oo...4.oo...&#x | 0 1 1 0 0 0 | * * * * 8 * * * * * * * * | 0 0 1 1 0 0 0 0
...... ..x... | 0 0 2 0 0 0 | * * * * * 4 * * * * * * * | 0 0 1 0 1 0 0 0
..oo..4..oo..&#x | 0 0 1 1 0 0 | * * * * * * 8 * * * * * * | 0 0 0 1 1 0 0 0
...... ...x.. | 0 0 0 2 0 0 | * * * * * * * 4 * * * * * | 0 0 0 0 1 1 0 0
...oo.4...oo.&#x | 0 0 0 1 1 0 | * * * * * * * * 8 * * * * | 0 0 0 1 0 1 0 0
....x. ...... | 0 0 0 0 2 0 | * * * * * * * * * 4 * * * | 0 0 0 1 0 0 1 0
....oo4....oo&#x | 0 0 0 0 1 1 | * * * * * * * * * * 8 * * | 0 0 0 0 0 1 1 0
.....x ...... | 0 0 0 0 0 2 | * * * * * * * * * * * 4 * | 0 0 0 0 0 0 1 1
...... .....x | 0 0 0 0 0 2 | * * * * * * * * * * * * 4 | 0 0 0 0 0 1 0 1
------------------+-------------+---------------------------+----------------
x.....4x..... | 8 0 0 0 0 0 | 4 4 0 0 0 0 0 0 0 0 0 0 0 | 1 * * * * * * *
xx.... ......&#x | 2 2 0 0 0 0 | 1 0 2 1 0 0 0 0 0 0 0 0 0 | * 4 * * * * * *
...... xux...&#xt | 2 2 2 0 0 0 | 0 1 2 0 2 1 0 0 0 0 0 0 0 | * * 4 * * * * *
.xwwx. ......&#xt | 0 2 2 2 2 0 | 0 0 0 1 2 0 2 0 2 1 0 0 0 | * * * 4 * * * *
...... ..xx..&#x | 0 0 2 2 0 0 | 0 0 0 0 0 1 2 1 0 0 0 0 0 | * * * * 4 * * *
...... ...xux&#xt | 0 0 0 2 2 2 | 0 0 0 0 0 0 0 1 2 0 2 0 1 | * * * * * 4 * *
....xx ......&#x | 0 0 0 0 2 2 | 0 0 0 0 0 0 0 0 0 1 2 1 0 | * * * * * * 4 *
.....x4.....x | 0 0 0 0 0 8 | 0 0 0 0 0 0 0 0 0 0 0 4 4 | * * * * * * * 1
or
o.....4o..... & | 16 * * | 1 1 1 0 0 0 0 | 1 1 1 0 0
.o....4.o.... & | * 16 * | 0 0 1 1 1 0 0 | 0 1 1 1 0
..o...4..o... & | * * 16 | 0 0 0 0 1 1 1 | 0 0 1 1 1
---------------------+----------+-----------------+----------
x..... ...... & | 2 0 0 | 8 * * * * * * | 1 1 0 0 0
...... x..... & | 2 0 0 | * 8 * * * * * | 1 0 1 0 0
oo....4oo....&#x & | 1 1 0 | * * 16 * * * * | 0 1 1 0 0
.x.... ...... & | 0 2 0 | * * * 8 * * * | 0 1 0 1 0
.oo...4.oo...&#x & | 0 1 1 | * * * * 16 * * | 0 0 1 1 0
...... ..x... & | 0 0 2 | * * * * * 8 * | 0 0 1 0 1
..oo..4..oo..&#x | 0 0 2 | * * * * * * 8 | 0 0 0 1 1
---------------------+----------+-----------------+----------
x.....4x..... & | 8 0 0 | 4 4 0 0 0 0 0 | 2 * * * *
xx.... ......&#x & | 2 2 0 | 1 0 2 1 0 0 0 | * 8 * * *
...... xux...&#xt & | 2 2 2 | 0 1 2 0 2 1 0 | * * 8 * *
.xwwx. ......&#xt | 0 4 4 | 0 0 0 2 4 0 2 | * * * 4 *
...... ..xx..&#x | 0 0 4 | 0 0 0 0 0 2 2 | * * * * 4
wx3xx3xw&#zx → height = 0
(tegum sum of 2 mutually inverse (w,x,x)-toes)
o.3o.3o. | 24 * | 1 1 1 0 0 | 1 1 1 0
.o3.o3.o | * 24 | 0 0 1 1 1 | 0 1 1 1
-------------+-------+----------------+---------
.. x. .. | 2 0 | 12 * * * * | 1 0 1 0
.. .. x. | 2 0 | * 12 * * * | 1 1 0 0
oo3oo3oo&#x | 1 1 | * * 24 * * | 0 1 1 0
.x .. .. | 0 2 | * * * 12 * | 0 1 0 1
.. .x .. | 0 2 | * * * * 12 | 0 0 1 1
-------------+-------+----------------+---------
.. x.3x. | 6 0 | 3 3 0 0 0 | 4 * * *
wx .. xw&#zx | 4 4 | 0 2 4 2 0 | * 6 * *
.. xx ..&#x | 2 2 | 1 0 2 0 1 | * * 12 *
.x3.x .. | 0 6 | 0 0 0 3 3 | * * * 4
or
o.3o.3o. & | 48 | 1 1 1 | 1 1 1
---------------+----+----------+-------
.. x. .. & | 2 | 24 * * | 1 0 1
.. .. x. & | 2 | * 24 * | 1 1 0
oo3oo3oo&#x | 2 | * * 24 | 0 1 1
---------------+----+----------+-------
.. x.3x. & | 6 | 3 3 0 | 8 * *
wx .. xw&#zx | 8 | 0 4 4 | * 6 *
.. xx ..&#x | 4 | 2 0 2 | * * 12
xwX wxx4xux&#zxt → height = 0, X=x+q+q = 3.828427
o.. o..4o.. | 16 * * | 1 1 1 0 0 0 0 | 1 1 1 0 0
.o. .o.4.o. | * 16 * | 0 0 1 1 1 0 0 | 0 1 1 1 0
..o ..o4..o | * * 16 | 0 0 0 0 1 1 1 | 0 0 1 1 1
----------------+----------+-----------------+----------
x.. ... ... | 2 0 0 | 8 * * * * * * | 1 1 0 0 0
... ... x.. | 2 0 0 | * 8 * * * * * | 1 0 1 0 0
oo. oo.4oo.&#x | 1 1 0 | * * 16 * * * * | 0 1 1 0 0
... .x. ... | 0 2 0 | * * * 8 * * * | 0 1 0 1 0
.oo .oo4.oo&#x | 0 1 1 | * * * * 16 * * | 0 0 1 1 0
... ..x ... | 0 0 2 | * * * * * 8 * | 0 0 0 1 1
... ... ..x | 0 0 2 | * * * * * * 8 | 0 0 1 0 1
----------------+----------+-----------------+----------
x.. ... x.. | 4 0 0 | 2 2 0 0 0 0 0 | 4 * * * *
xw. wx. ...&#zx | 4 4 0 | 2 0 4 2 0 0 0 | * 4 * * *
... ... xux&#xt | 2 2 2 | 0 1 2 0 2 0 1 | * * 8 * *
... .xx ...&#x | 0 2 2 | 0 0 0 1 2 1 0 | * * * 8 *
... ..x4..x | 0 0 8 | 0 0 0 0 0 4 4 | * * * * 2