|
Acronym
|
poxic (alt.: phic srico, alt.: owau sidpith)
|
|
Name
|
partially octa-expanded icositetrachoron,
partially hexadeca-contracted small rhombated icositetrachoron,
octa-augmented small diprismated tesseractithexadecachoron
|
|
|
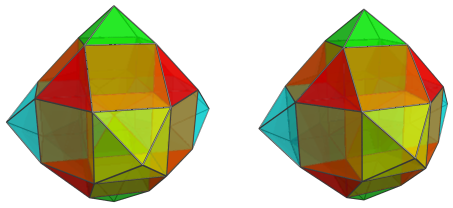 © ©
|
|
Circumradius
|
...
|
Lace city
in approx. ASCII-art
|
o4o
x4o x4o
x4o x4x x4x x4o
o4o o4w o4o
x4o x4x x4x x4o
x4o x4o
o4o
|
|
Coordinates
|
-
(1+sqrt(2), 1, 1, 1)/2 & all permutations, all changes of sign
(vertex inscribed sidpith)
-
(2+sqrt(2), 0, 0, 0)/2 & all permutations, all changes of sign
(vertex inscribed w-hex)
|
|
Dihedral angles
|
|
|
Face vector
|
72, 256, 256, 72
|
|
Confer
|
- uniform relative:
-
ico
srico
sidpith
- related segmentochora:
-
cubpy
cubasirco
- related CRFs:
-
oox3ooo4oxx&#xt
pocsric
oxwQ wxoo3xxxx4oxxo&#zx
dapabdi poxic
- general polytopal classes:
-
partial Stott expansions
|
External
links
|

|
This CRF polychoron can be obtained from ico by
partial Stott expanding only 8 of its vertices
(in hexadecachoral positioning). – Note that its octs thereby become elongated into
esquidpies, by themselves thus outlining a somehow thickened edge skeleton of hex.
And, in this comparison to hex, the other cells too can be understood either as its former cells (tets)
resp. as its likewise thickened former triangular faces (trips).
Conversely it can be obtained by a similar partial Stott contraction from
pocsric (which in turn is derived by such a contraction from srico).
Alternatively it can be obtained by augmenting the 8 full symmetrical cubes of
sidpith by cubpy. Then the thus introduced squippies
would recombine with the remaining (only prism symmetric) cubes into esquidpies.
Incidence matrix according to Dynkin symbol
wx3oo3oo4ox&#zx → height = 0
(tegum sum of w-hex and sidpith)
o.3o.3o.4o. | 8 * ♦ 8 0 0 | 12 0 0 | 6 0 0
.o3.o3.o4.o | * 64 | 1 3 3 | 3 3 6 | 3 1 3
----------------+------+----------+----------+---------
oo3oo3oo4oo&#x | 1 1 | 64 * * | 3 0 0 | 3 0 0
.x .. .. .. | 0 2 | * 96 * | 0 2 2 | 1 1 2
.. .. .. .x | 0 2 | * * 96 | 1 0 2 | 2 0 1
----------------+------+----------+----------+---------
.. .. .. ox&#x | 1 2 | 2 0 1 | 96 * * | 2 0 0
.x3.o .. .. | 0 3 | 0 3 0 | * 64 * | 0 1 1
.x .. .. .x | 0 4 | 0 2 2 | * * 96 | 1 0 1
----------------+------+----------+----------+---------
wx .. oo4ox&#zx ♦ 2 8 | 8 4 8 | 8 0 4 | 24 * *
.x3.o3.o .. ♦ 0 4 | 0 6 0 | 0 4 0 | * 16 *
.x3.o .. .x ♦ 0 6 | 0 6 3 | 0 2 3 | * * 32
wxx3ooo3oqo *b3ooq&#zx → height = 0
(tegum sum of w-hex and 2 mutually gyrated (x,q)-rits)
o..3o..3o.. *b3o.. | 8 * * ♦ 4 4 0 0 0 | 12 0 0 0 | 6 0 0 0
.o.3.o.3.o. *b3.o. | * 32 * | 1 0 3 3 0 | 3 3 6 0 | 3 1 3 0
..o3..o3..o *b3..o | * * 32 | 0 1 0 3 3 | 3 0 6 3 | 3 0 3 1
-----------------------+---------+----------------+-------------+----------
oo.3oo.3oo. *b3oo.&#x | 1 1 0 | 32 * * * * | 3 0 0 0 | 3 0 0 0
o.o3o.o3o.o *b3o.o&#x | 1 0 1 | * 32 * * * | 3 0 0 0 | 3 0 0 0
.x. ... ... ... | 0 2 0 | * * 48 * * | 0 2 2 0 | 1 1 2 0
.oo3.oo3.oo *b3.oo&#x | 0 1 1 | * * * 96 * | 1 0 2 0 | 2 0 1 0
..x ... ... ... | 0 0 2 | * * * * 48 | 0 0 2 2 | 1 0 2 1
-----------------------+---------+----------------+-------------+----------
ooo3ooo3ooo *b3ooo&#x | 1 1 1 | 1 1 0 1 0 | 96 * * * | 2 0 0 0
.x.3.o. ... ... | 0 3 0 | 0 0 3 0 0 | * 32 * * | 0 1 1 0
.xx ... ... ...&#x | 0 2 2 | 0 0 1 2 1 | * * 96 * | 1 0 1 0
..x3..o ... ... | 0 0 3 | 0 0 0 0 3 | * * * 32 | 0 0 1 1
-----------------------+---------+----------------+-------------+----------
wxx ... oqo ooq&#zx ♦ 2 4 4 | 4 4 2 8 2 | 8 0 4 0 | 24 * * *
.x.3.o. ... *b3.o. ♦ 0 4 0 | 0 0 6 0 0 | 0 4 0 0 | * 8 * *
.xx3.oo ... ...&#x ♦ 0 3 3 | 0 0 3 3 3 | 0 1 3 1 | * * 32 *
..x3..o3..o ... ♦ 0 0 4 | 0 0 0 0 6 | 0 0 0 4 | * * * 8
oxwU wxoo3oooo4oxxo&#zx → height = 0 (U=w+x=q+u=q+2x)
(tegum sum of equatorial w-oct, sircope, (w,x)-tes, and ortho U-line)
o... o...3o...4o... | 6 * * * ♦ 8 0 0 0 0 0 0 | 4 8 0 0 0 0 0 0 | 4 2 0 0 0
.o.. .o..3.o..4.o.. | * 48 * * | 1 1 2 2 1 0 0 | 1 2 2 1 2 2 2 0 | 2 1 1 1 2
..o. ..o.3..o.4..o. | * * 16 * | 0 0 0 0 3 3 1 | 0 0 0 0 0 3 6 3 | 0 3 0 1 3
...o ...o3...o4...o | * * * 2 ♦ 0 0 0 0 0 0 8 | 0 0 0 0 0 0 0 12 | 0 6 0 0 0
------------------------+-----------+----------------------+-------------------------+--------------
oo.. oo..3oo..4oo..&#x | 1 1 0 0 | 48 * * * * * * | 1 2 0 0 0 0 0 0 | 2 1 0 0 0
.x.. .... .... .... | 0 2 0 0 | * 24 * * * * * | 1 0 2 0 0 0 0 0 | 2 0 1 0 0
.... .x.. .... .... | 0 2 0 0 | * * 48 * * * * | 0 0 1 1 1 1 0 0 | 1 0 1 1 1
.... .... .... .x.. | 0 2 0 0 | * * * 48 * * * | 0 1 0 0 1 0 1 0 | 1 1 0 0 1
.oo. .oo.3.oo.4.oo.&#x | 0 1 1 0 | * * * * 48 * * | 0 0 0 0 0 2 2 0 | 0 1 0 1 2
.... .... .... ..x. | 0 0 2 0 | * * * * * 24 * | 0 0 0 0 0 0 2 1 | 0 2 0 0 1
..oo ..oo3..oo4..oo&#x | 0 0 1 1 | * * * * * * 16 | 0 0 0 0 0 0 0 3 | 0 3 0 0 0
------------------------+-----------+----------------------+-------------------------+--------------
ox.. .... .... ....&#x | 1 2 0 0 | 2 1 0 0 0 0 0 | 24 * * * * * * * | 2 0 0 0 0
.... .... .... ox..&#x | 1 2 0 0 | 2 0 0 1 0 0 0 | * 48 * * * * * * | 1 1 0 0 0
.x.. .x.. .... .... | 0 4 0 0 | 0 2 2 0 0 0 0 | * * 24 * * * * * | 1 0 1 0 0
.... .x..3.o.. .... | 0 3 0 0 | 0 0 3 0 0 0 0 | * * * 16 * * * * | 0 0 1 1 0
.... .x.. .... .x.. | 0 4 0 0 | 0 0 2 2 0 0 0 | * * * * 24 * * * | 1 0 0 0 1
.... .xo. .... ....&#x | 0 2 1 0 | 0 0 1 0 2 0 0 | * * * * * 48 * * | 0 0 0 1 1
.... .... .... .xx.&#x | 0 2 2 0 | 0 0 0 1 2 1 0 | * * * * * * 48 * | 0 1 0 0 1
.... .... .... ..xo&#x | 0 0 2 1 | 0 0 0 0 0 1 2 | * * * * * * * 24 | 0 2 0 0 0
------------------------+-----------+----------------------+-------------------------+--------------
ox.. wx.. .... ox..&#zx ♦ 2 8 0 0 | 8 4 4 4 0 0 0 | 4 4 2 0 2 0 0 0 | 12 * * * *
.... .... oooo4oxxo&#xt ♦ 1 4 4 1 | 4 0 0 4 4 4 4 | 0 4 0 0 0 0 4 4 | * 12 * * *
.x.. .x..3.o.. .... ♦ 0 6 0 0 | 0 3 6 0 0 0 0 | 0 0 3 2 0 0 0 0 | * * 8 * *
.... .xo.3.oo. ....&#x ♦ 0 3 1 0 | 0 0 3 0 3 0 0 | 0 0 0 1 0 3 0 0 | * * * 16 *
.... .xo. .... .xx.&#x ♦ 0 4 2 0 | 0 0 2 2 4 1 0 | 0 0 0 0 1 2 2 0 | * * * * 24
 ©
©