I had the idea to design a fantasy script from combinations of a small repertoire of features: namely, subsets of this set of twelve segments. Using a fixed number of segments gives some built-in error-detection. There are 924 subsets of six segments; discarding those that form disconnected graphs leaves 306, more than enough for a syllabary. (Syllabaries have been invented more often than other types of scripts, but they’re underrepresented in fantasy.)
These figures can be considered as points on an oblique hyperplane bisecting a 12-dimensional hypercube; this hyperplane has 11 dimensions. I hoped to find a neat projection of that arrangement into 2 or 3 dimensions, but
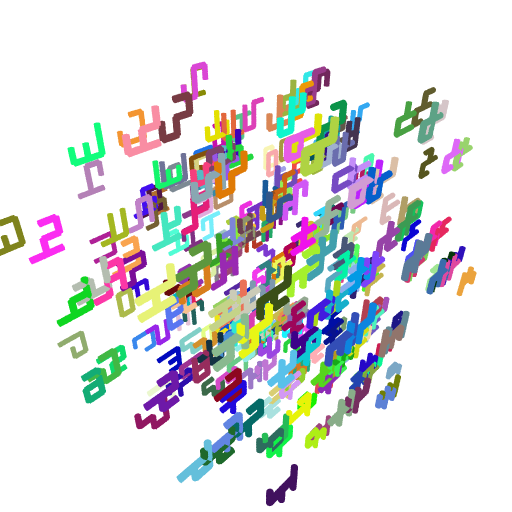
none has turned up yet.
I provisionally call these characters Takana: like Japanese kana they are intended to represent syllables (though I haven’t bothered to make assignments yet); and the superset figure resembles the kanji pronounced ta (meaning ‘field’).
Having written the above, I now think I see how to look for a good projection: list the 46665 points on the projective hypersphere that represent directions of lines joining two of the 306 nodes, find the Voronoi centers of those points, and choose three such centers roughly at right angles to each other.
Later: That’s still not right. What I want is a (n-3)-dimensional hyperplane in projective space that maximizes its minimum angle from any of the 46665 lines; collapsing the dimensions of that hyperplane should reduce the figure to 3 dimensions without collisions. But how to find that hyperplane, I have no idea. In higher dimensions my intuition stinks.
2008: Takana go goth
I verified the number 306 for fun.
I’m impressed. Is that APL? I counted the connected graphs by hand.
It’s in the J programming language, which is a successor of apl designed by the late Ken Iverson, the inventor of APL. J is supposed to have all features of APL and even more; it has better higher-order functions, and it’s pure ascii.
I shoulda included a list of the figures.
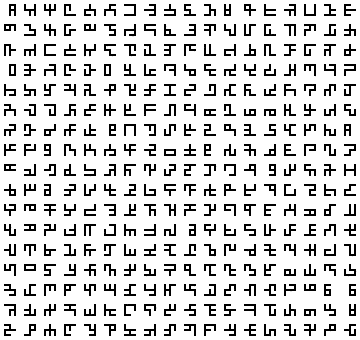
They’re in random order.
One of these pictures attracted a search for how to sing by yourself.
Tacana is the name of a language spoken in Bolivia.
Very nice, but how many are unique including rotation?
If rotation includes reflection (rotation axis in the plane), 45. If not: of those, 32 are chiral if I did not miscount, so 77.