⭳

the corresponding VRML preview in this canvas
= srid
= saddid
= sird
= pecu

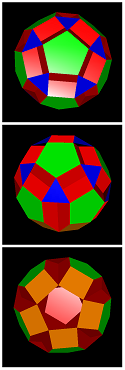
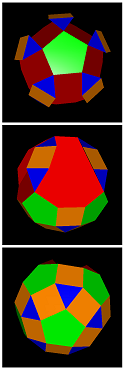
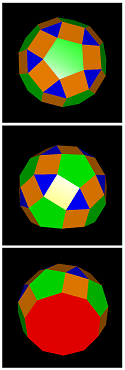
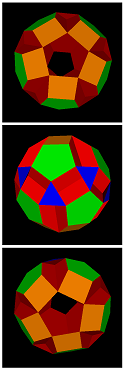
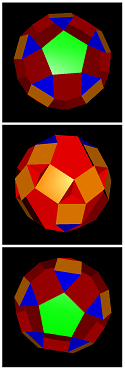

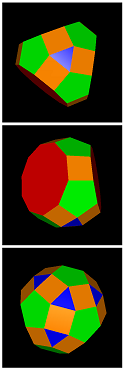
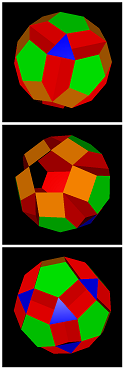
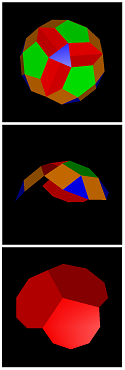
= dirid
= pabidrid
= tedrid
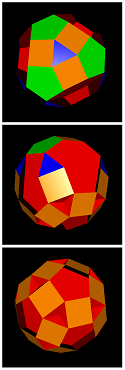
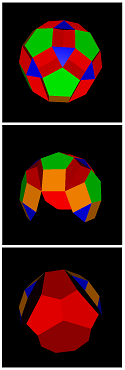
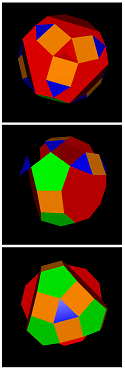
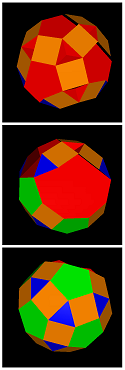
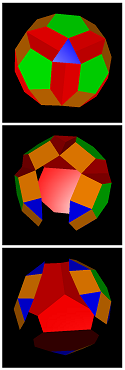
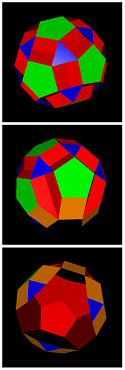
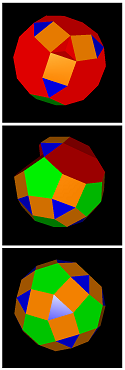
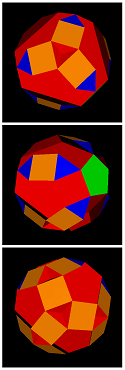

| Site Map | Polytopes | Dynkin Diagrams | Vertex Figures, etc. | Incidence Matrices | Index |
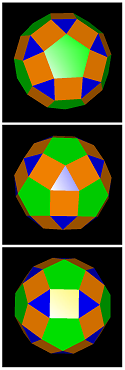
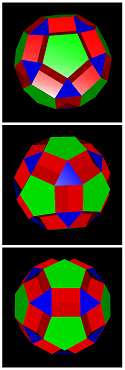
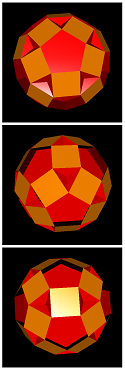
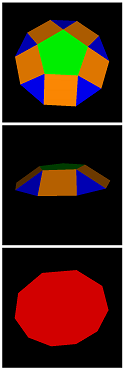
Possible facets here are the 20 triangles, 30 squares, and 12 pentagons of the comodore itself together with the 12 internal decagons. The general naming code here is accordingly srid-#{3}-#{4}-#{5}-#{10}. This gives rise for exactly 30 edge-facetings with n-gonal axial rotation symmetries, provided n > 2. In fact, 3 have full icosahedral symmetry, 10 have 5-fold pyramidal symmetry, 3 have 5-fold antiprismatic symmetry, 11 have 3-fold pyramidal symmetry, and 3 have 3-fold antiprismatic symmetry.
A well-known edge-faceting with just digonal symmetry (i.e. mirror symmetry) is the Johnson solid J81 (mabidrid).
|

|

|

|

|

|

|

|

| ||
|
clicking the triple-pictures of the table provides the corresponding VRML preview in this canvas |
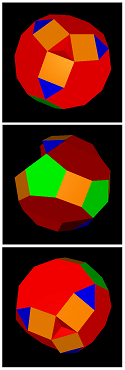
srid-20-30-12-0 = srid |
srid-20-0-12-12 = saddid |
srid-0-30-0-12 = sird |
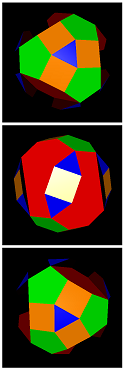
srid-5-5-1-1 = pecu | srid-5-15-5-5 | srid-5-15-5-7 | srid-5-25-1-11 | srid-10-10-6-6-a | ||
| icosahedral | 5-fold pyramidal ... | |||||||||

|

|

|

|

|

|

|

|

|

|

|
| srid-10-10-6-6-b | srid-10-20-6-6 | srid-15-5-11-11 | srid-15-15-7-5 |
srid-15-25-11-1 = dirid | srid-10-10-10-10 | srid-10-20-2-10 |
srid-10-20-10-2 = pabidrid |
srid-5-15-9-3 = tedrid | srid-5-15-9-9 | srid-7-9-3-3 |
| ... 5-fold pyramidal | 5-fold antiprismatic | 3-fold pyramidal ... | ||||||||

|

|

|

|

|

|

|

|

|

|

|
| srid-7-21-3-9 | srid-10-12-6-6-a | srid-10-12-6-6-b | srid-10-18-6-6-a | srid-10-18-6-6-b | srid-13-9-9-9 | srid-13-21-9-3 | srid-15-15-3-9 | srid-6-12-6-6 | srid-6-18-6-6 | srid-14-12-6-6 |
| ... 3-fold pyramidal | 3-fold antiprismatic | |||||||||
© 2004-2025 | top of page |