| Acronym | idsrahi |
| Name | icositetra-diminished small rhombated hecatonicosachoron |
| Circumradius | sqrt[23+10 sqrt(5)] = 6.735034 |
| Face vector | 2160, 5040, 3600, 720 |
| Confer |
|

In the same way, as sadi was derived as an icositetrachoral diminishing of ex, idsrahi can be derived as an icositetrachoral diminishing of srahi. The fundamental domain of sadi (i.e. titdi, the dual of teddi), which there clearly encompasses just a single vertex, here would encompass a complete srid. Here the pentagons of this srid will lie right in the face planes of the dodecahedral kernel of that domain. Therefore idsrahi clearly is no longer uniform (as weren't lots of the Johnson solids neither).
Sadi was diminished at 24 vertices. Idsrahi accordingly will be diminished at 24 srids. In fact 24 srid-first caps will be chopped off. Any individual of those clearly is a srid || tid. This results in several other srid, which get diminished into tedrid here.
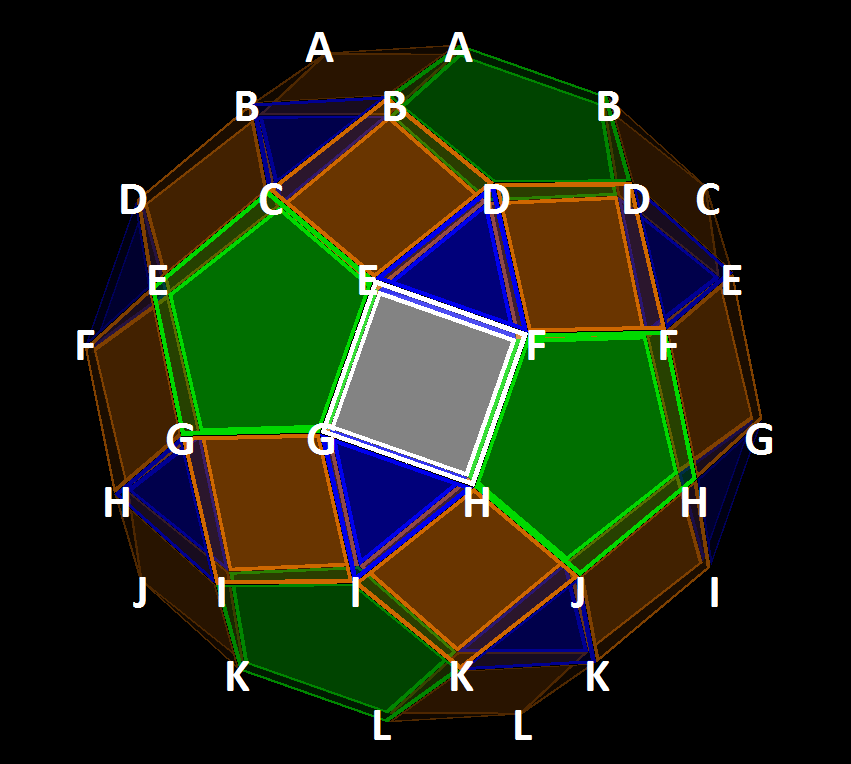
Corresponding to the 3-fold symmetry of that domain we call the vertices of srid as A, ..., L along its 3-fold axis. The other elements of idsrahi then can be classified accordingly, as given below. The srid vertices C, E, and G then would be chopped off, as those get eaten by the tids, which are to be placed around the tips of the domain. This is how the srids become tedrids. All the remaining vertex types then are placed right at the boundary of the used fundamental domain. By external symmetry (connection of those domains) we further get the identifications A=J, B=H, D=F.
A=J | 288 * * * * * | 2 2 0 0 0 0 0 0 0 2 0 0 0 | 1 2 2 1 0 0 0 0 2 0 1 0 0 0 0 | 2 2 0 0 1 0 0 B=H | * 576 * * * * | 0 1 1 1 0 0 1 0 0 0 0 0 0 | 0 1 0 1 2 1 0 0 1 0 0 0 0 0 0 | 2 1 0 0 0 0 1 D=F | * * 576 * * * | 0 0 0 1 1 2 0 0 0 0 0 0 0 | 0 0 0 1 2 0 1 2 0 0 0 0 0 0 0 | 2 0 1 0 0 0 1 I | * * * 288 * * | 0 0 0 0 0 0 2 1 1 0 0 0 0 | 0 0 0 0 2 1 0 0 2 1 0 0 0 0 0 | 2 1 0 0 0 0 1 K | * * * * 288 * | 0 0 0 0 0 0 0 0 1 2 2 1 0 | 0 0 1 0 0 0 0 0 2 1 2 1 2 0 0 | 2 1 0 1 1 0 0 L | * * * * * 144 | 0 0 0 0 0 0 0 0 0 0 0 2 4 | 0 0 0 0 0 0 0 0 0 1 0 0 4 2 2 | 2 0 0 2 0 1 0 ------------+-------------------------+-----------------------------------------------------+---------------------------------------------------------+---------------------- AA | 2 0 0 0 0 0 | 288 * * * * * * * * * * * * | 1 1 1 0 0 0 0 0 0 0 0 0 0 0 0 | 1 1 0 0 1 0 0 AB=HJ | 1 1 0 0 0 0 | * 576 * * * * * * * * * * * | 0 1 0 1 0 0 0 0 1 0 0 0 0 0 0 | 2 1 0 0 0 0 0 BB | 0 2 0 0 0 0 | * * 288 * * * * * * * * * * | 0 1 0 0 1 1 0 0 0 0 0 0 0 0 0 | 1 1 0 0 0 0 1 BD=FH | 0 1 1 0 0 0 | * * * 576 * * * * * * * * * | 0 0 0 1 2 0 0 0 0 0 0 0 0 0 0 | 2 0 0 0 0 0 1 DD=FF | 0 0 2 0 0 0 | * * * * 288 * * * * * * * * | 0 0 0 1 0 0 0 2 0 0 0 0 0 0 0 | 2 0 1 0 0 0 0 DF | 0 0 2 0 0 0 | * * * * * 576 * * * * * * * | 0 0 0 0 1 0 1 1 0 0 0 0 0 0 0 | 1 0 1 0 0 0 1 HI | 0 1 0 1 0 0 | * * * * * * 576 * * * * * * | 0 0 0 0 1 1 0 0 1 0 0 0 0 0 0 | 1 1 0 0 0 0 1 II | 0 0 0 2 0 0 | * * * * * * * 144 * * * * * | 0 0 0 0 2 0 0 0 0 1 0 0 0 0 0 | 2 0 0 0 0 0 1 IK | 0 0 0 1 1 0 | * * * * * * * * 288 * * * * | 0 0 0 0 0 0 0 0 2 1 0 0 0 0 0 | 2 1 0 0 0 0 0 JK | 1 0 0 0 1 0 | * * * * * * * * * 576 * * * | 0 0 1 0 0 0 0 0 1 0 1 0 0 0 0 | 1 1 0 0 1 0 0 KK | 0 0 0 0 2 0 | * * * * * * * * * * 288 * * | 0 0 0 0 0 0 0 0 0 0 1 1 1 0 0 | 1 0 0 1 1 0 0 KL | 0 0 0 0 1 1 | * * * * * * * * * * * 288 * | 0 0 0 0 0 0 0 0 0 1 0 0 2 0 0 | 2 0 0 1 0 0 0 LL | 0 0 0 0 0 2 | * * * * * * * * * * * * 288 | 0 0 0 0 0 0 0 0 0 0 0 0 1 1 1 | 1 0 0 1 0 1 0 ------------+-------------------------+-----------------------------------------------------+---------------------------------------------------------+---------------------- AAA | 3 0 0 0 0 0 | 3 0 0 0 0 0 0 0 0 0 0 0 0 | 96 * * * * * * * * * * * * * * | 1 0 0 0 1 0 0 AABB | 2 2 0 0 0 0 | 1 2 1 0 0 0 0 0 0 0 0 0 0 | * 288 * * * * * * * * * * * * * | 1 1 0 0 0 0 0 AAK | 2 0 0 0 1 0 | 1 0 0 0 0 0 0 0 0 2 0 0 0 | * * 288 * * * * * * * * * * * * | 0 1 0 0 1 0 0 ABDDB=FFHJH | 1 2 2 0 0 0 | 0 2 0 2 1 0 0 0 0 0 0 0 0 | * * * 288 * * * * * * * * * * * | 2 0 0 0 0 0 0 BBDFHIIHFD | 0 4 4 2 0 0 | 0 0 1 4 0 2 2 1 0 0 0 0 0 | * * * * 288 * * * * * * * * * * | 1 0 0 0 0 0 1 BBI | 0 2 0 1 0 0 | 0 0 1 0 0 0 2 0 0 0 0 0 0 | * * * * * 288 * * * * * * * * * | 0 1 0 0 0 0 1 DDD | 0 0 3 0 0 0 | 0 0 0 0 0 3 0 0 0 0 0 0 0 | * * * * * * 192 * * * * * * * * | 0 0 1 0 0 0 1 DDFF | 0 0 4 0 0 0 | 0 0 0 0 2 2 0 0 0 0 0 0 0 | * * * * * * * 288 * * * * * * * | 1 0 1 0 0 0 0 HIKJ | 1 1 0 1 1 0 | 0 1 0 0 0 0 1 0 1 1 0 0 0 | * * * * * * * * 576 * * * * * * | 1 1 0 0 0 0 0 IIKLK | 0 0 0 2 2 1 | 0 0 0 0 0 0 0 1 2 0 0 2 0 | * * * * * * * * * 144 * * * * * | 2 0 0 0 0 0 0 JKK | 1 0 0 0 2 0 | 0 0 0 0 0 0 0 0 0 2 1 0 0 | * * * * * * * * * * 288 * * * * | 1 0 0 0 1 0 0 KKK | 0 0 0 0 3 0 | 0 0 0 0 0 0 0 0 0 0 3 0 0 | * * * * * * * * * * * 96 * * * | 0 0 0 1 1 0 0 KKLL | 0 0 0 0 2 2 | 0 0 0 0 0 0 0 0 0 0 1 2 1 | * * * * * * * * * * * * 288 * * | 1 0 0 1 0 0 0 LLL | 0 0 0 0 0 3 | 0 0 0 0 0 0 0 0 0 0 0 0 3 | * * * * * * * * * * * * * 96 * | 1 0 0 0 0 1 0 LLL' | 0 0 0 0 0 3 | 0 0 0 0 0 0 0 0 0 0 0 0 3 | * * * * * * * * * * * * * * 96 | 0 0 0 1 0 1 0 ------------+-------------------------+-----------------------------------------------------+---------------------------------------------------------+---------------------- tedrid | 6 12 12 6 6 3 | 3 12 3 12 6 6 6 3 6 6 3 6 3 | 1 3 0 6 3 0 0 3 6 3 3 0 3 1 0 | 96 * * * * * * AABBIK-trip | 2 2 0 1 1 0 | 1 2 1 0 0 0 2 0 1 2 0 0 0 | 0 1 1 0 0 1 0 0 2 0 0 0 0 0 0 | * 288 * * * * * DDDDDD-trip | 0 0 6 0 0 0 | 0 0 0 0 3 6 0 0 0 0 0 0 0 | 0 0 0 0 0 0 2 3 0 0 0 0 0 0 0 | * * 96 * * * * KKKLLL-trip | 0 0 0 0 3 3 | 0 0 0 0 0 0 0 0 0 0 3 3 3 | 0 0 0 0 0 0 0 0 0 0 0 1 3 0 1 | * * * 96 * * * AAAKKK-oct | 3 0 0 0 3 0 | 3 0 0 0 0 0 0 0 0 6 3 0 0 | 1 0 3 0 0 0 0 0 0 0 3 1 0 0 0 | * * * * 96 * * LLLLLL-oct | 0 0 0 0 0 6 | 0 0 0 0 0 0 0 0 0 0 0 0 12 | 0 0 0 0 0 0 0 0 0 0 0 0 0 4 4 | * * * * * 24 * tid | 0 24 24 12 0 0 | 0 0 12 24 0 24 24 6 0 0 0 0 0 | 0 0 0 0 12 12 8 0 0 0 0 0 0 0 0 | * * * * * * 24
© 2004-2025 | top of page |