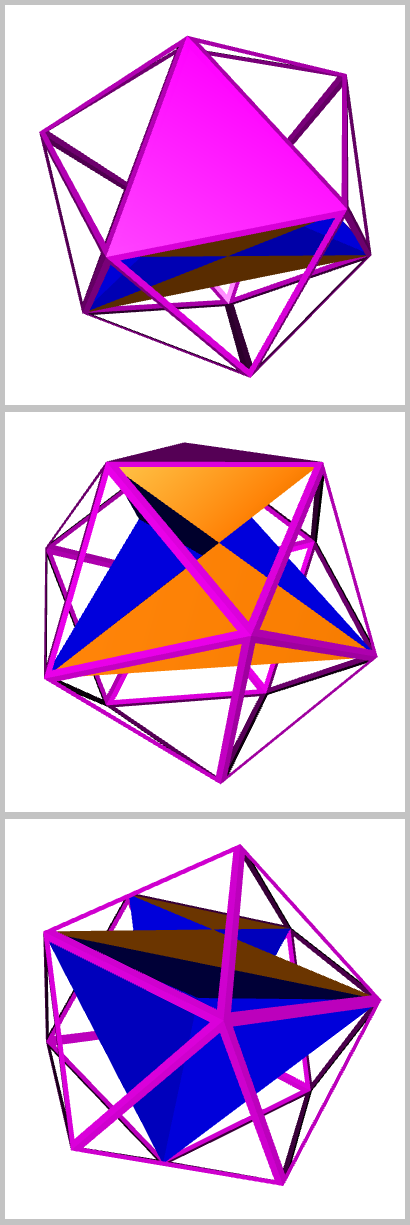
shematic display of both bases and one lacing starp
 ,
, 
at gissid's vertices at sissid's vertices
⭳ ⭳
- uniform relative:
- gax
- related scaliform:
- taller gissid || sissid
- general polytopal classes:
- segmentochora
| Acronym | gissidasissid |
| Name | shallower great stellated dodecahedron atop small stellated dodecahedron |
shematic display of both bases and one lacing starp | |
| Circumradius | (sqrt(5)-1)/2 = 0.618034 |
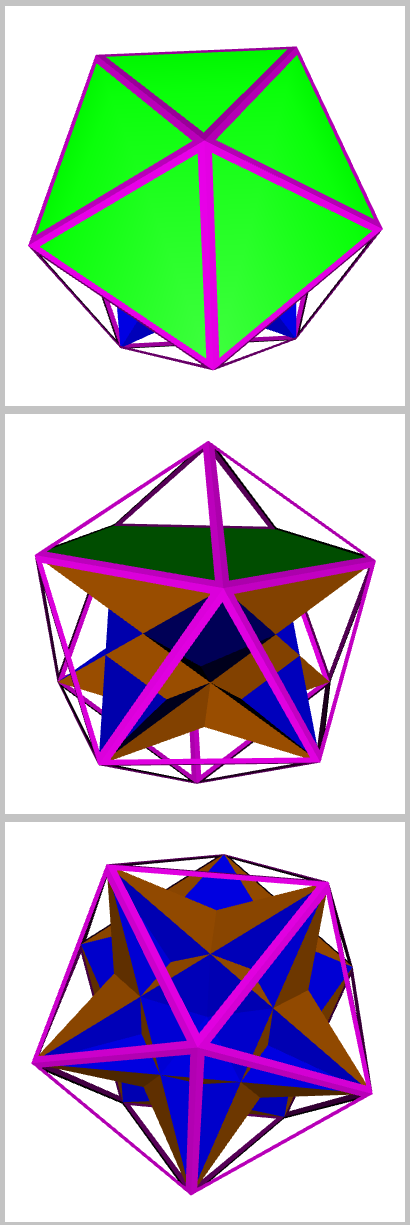
| Vertex figures |
 , , 
at gissid's vertices at sissid's vertices |
| VRML |
|
| Face vector | 32, 120, 142, 44 |
| Confer |
|
As abstract polytope gissidasissid is isomorph to gadadoe, therby replacing sissid by gad, gissid by doe, and starp by pap.
Its vertex figures above are displayed to be faceting of a v-ike. Therfrom it becomes evident that this polychoron itself occurs as an edge-faceting within gax.
It was found by the author in early 2023 shortly after Mecejide's find of gikaike.
Both the shallower and taller gissid || sissid have the same incidence matrix. In fact the bases are aligned in the same mutual orientation, just within different heights.
Within the shallower one the starps connect anti-aligned pentagrams on the same side each, while in the taller one the staps connect aligned pentagrams on the opposite side each. Likewise, in the shallower one tets connect orthogonal edges on the opposite side each, while in the taller one the tets connect orthogonal edges on the same side each.
Similarily in the shallower one the axially trigonal vertex figure at the gissid base consists of 2 aligned triangles (of different sizes) and the lacing tetragons are crossed trapezoids, while in the taller one the axially trigonal vertex figure at the gissid base consists of 2 anti-aligned triangles (of different sizes) and the lacing tetragons are convex trapezoids. Alike in the shallower one the axially pentagonal vertex figure at the sissid base is a segmentohedron spanned by an anti-aligned pentagon-pentagram pair (of same circumradius) and the lacing tetragons are crossed trapezoids, while in the taller one the axially pentagonal vertex figure at the sissid base is a segmentohedron spanned by an aligned pentagon-pentagram pair (of same circumradius) and the lacing tetragons are convex trapezoids.
gissid || sissid → height = 1/2
20 * | 3 3 0 | 3 6 3 0 | 1 3 3 0
* 12 | 0 5 5 | 0 5 10 5 | 0 5 5 1
------+----------+-------------+----------
2 0 | 30 * * | 2 2 0 0 | 1 2 1 0
1 1 | * 60 * | 0 2 2 0 | 0 2 2 0
0 2 | * * 30 | 0 0 2 2 | 0 2 1 1
------+----------+-------------+----------
5 0 | 5 0 0 | 12 * * * | 1 1 0 0 {5/2}
2 1 | 1 2 0 | * 60 * * | 0 1 1 0
1 2 | 0 2 1 | * * 60 * | 0 1 1 0
0 5 | 0 0 5 | * * * 12 | 0 1 0 1 {5/2}
------+----------+-------------+----------
20 0 | 30 0 0 | 12 0 0 0 | 1 * * * gissid
5 5 | 5 10 5 | 1 5 5 1 | * 12 * * starp
2 2 | 1 4 1 | 0 2 2 0 | * * 30 * tet
0 12 | 0 0 30 | 0 0 0 12 | * * * 1 sissid
© 2004-2025 | top of page |