©
| Acronym | ..., xFoFx3xxxxx5xofox&#xt (F=ff=x+f) |
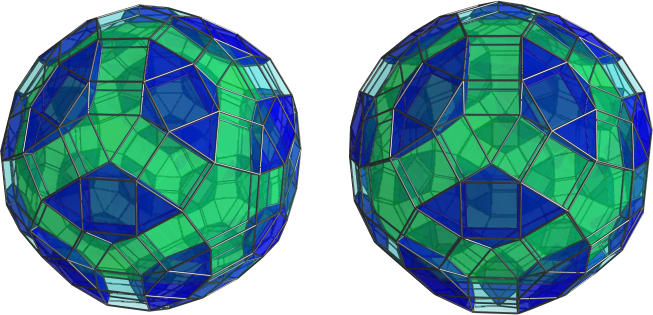
| Name | bilunabirotundic great rhombicosidodecahedron prism |
| |
| Face vector | 420, 1080, 828, 168 |
It should by pointed out here, that in this figure the axial icosahedral symmetry cannot be replaced by e.g. an octahedral one, as it could for the ursachora. This is due to he existence of the equatorial type of vertices here. These in fact are rigid, and so the attached bilbiroes have a fixed slope with respect to that partial complex of incident cells. But then, if trying to attach such a shard directly or parallely next to it, it obviously has to result in that here already used axial icosahedral symmetry.
Incidence matrix according to Dynkin symbol
xFoFx3xxxxx5xofox&#xt → height(1,2) = height(4,5) = (sqrt(5)-1)/4 = 0.309017 (F=ff=x+f) height(2,3) = height(3,4) = 1/2 (grid || pseudo (F,x)-ti || pseudo (x,f)-tid || pseudo (F,x)-ti || grid) o....3o....5o.... & | 240 * * | 1 1 1 1 1 0 0 0 | 1 1 1 1 1 1 1 1 0 0 0 | 1 1 1 1 1 0 .o...3.o...5.o... & | * 120 * | 0 0 0 2 0 2 1 0 | 0 0 0 2 1 0 0 2 1 2 0 | 0 1 0 1 2 1 ..o..3..o..5..o.. | * * 60 | 0 0 0 0 4 0 0 2 | 0 0 0 0 0 2 4 2 0 0 1 | 0 0 2 1 2 0 ------------------------+------------+-------------------------------+---------------------------------------+----------------- x.... ..... ..... & | 2 0 0 | 120 * * * * * * * | 1 1 0 0 0 1 0 0 0 0 0 | 1 0 1 1 0 0 ..... x.... ..... & | 2 0 0 | * 120 * * * * * * | 1 0 1 1 0 0 1 0 0 0 0 | 1 1 1 0 1 0 ..... ..... x.... & | 2 0 0 | * * 120 * * * * * | 0 1 1 0 1 0 0 0 0 0 0 | 1 1 0 1 0 0 oo...3oo...5oo...&#x & | 1 1 0 | * * * 240 * * * * | 0 0 0 1 1 0 0 1 0 0 0 | 0 1 0 1 1 0 o.o..3o.o..5o.o..&#x & | 1 0 1 | * * * * 240 * * * | 0 0 0 0 0 1 1 1 0 0 0 | 0 0 1 1 1 0 ..... .x... ..... & | 0 2 0 | * * * * * 120 * * | 0 0 0 1 0 0 0 0 1 1 0 | 0 1 0 0 1 1 .o.o.3.o.o.5.o.o.&#x | 0 2 0 | * * * * * * 60 * | 0 0 0 0 0 0 0 2 0 2 0 | 0 0 0 1 2 1 ..... ..x.. ..... | 0 0 2 | * * * * * * * 60 | 0 0 0 0 0 0 2 0 0 0 1 | 0 0 2 0 1 0 ------------------------+------------+-------------------------------+---------------------------------------+----------------- x....3x.... ..... & | 6 0 0 | 3 3 0 0 0 0 0 0 | 40 * * * * * * * * * * | 1 0 1 0 0 0 x.... ..... x.... & | 4 0 0 | 2 0 2 0 0 0 0 0 | * 60 * * * * * * * * * | 1 0 0 1 0 0 ..... x....5x.... & | 10 0 0 | 0 5 5 0 0 0 0 0 | * * 24 * * * * * * * * | 1 1 0 0 0 0 ..... xx... .....&#x & | 2 2 0 | 0 1 0 2 0 1 0 0 | * * * 120 * * * * * * * | 0 1 0 0 1 0 ..... ..... xo...&#x & | 2 1 0 | 0 0 1 2 0 0 0 0 | * * * * 120 * * * * * * | 0 1 0 1 0 0 x.o.. ..... .....&#x & | 2 0 1 | 1 0 0 0 2 0 0 0 | * * * * * 120 * * * * * | 0 0 1 1 0 0 ..... x.x.. .....&#x & | 2 0 2 | 0 1 0 0 2 0 0 1 | * * * * * * 120 * * * * | 0 0 1 0 1 0 ooooo3ooooo5ooooo&#xt | 2 2 1 | 0 0 0 2 2 0 1 0 | * * * * * * * 120 * * * | 0 0 0 1 1 0 ..... .x...5.o... & | 0 5 0 | 0 0 0 0 0 5 0 0 | * * * * * * * * 24 * * | 0 1 0 0 0 1 ..... .x.x. .....&#x | 0 4 0 | 0 0 0 0 0 2 2 0 | * * * * * * * * * 60 * | 0 0 0 0 1 1 ..o..3..x.. ..... | 0 0 3 | 0 0 0 0 0 0 0 3 | * * * * * * * * * * 20 | 0 0 2 0 0 0 ------------------------+------------+-------------------------------+---------------------------------------+----------------- x....3x....5x.... & ♦ 120 0 0 | 60 60 60 0 0 0 0 0 | 20 30 12 0 0 0 0 0 0 0 0 | 2 * * * * * ..... xx...5xo...&#x & ♦ 10 5 0 | 0 5 5 10 0 5 0 0 | 0 0 1 5 5 0 0 0 1 0 0 | * 24 * * * * x.o..3x.x.. .....&#x & ♦ 6 0 3 | 3 3 0 0 6 0 0 3 | 1 0 0 0 0 3 3 0 0 0 1 | * * 40 * * * xFoFx ..... xofox&#xt ♦ 8 4 2 | 4 0 4 8 8 0 2 0 | 0 2 0 0 4 4 0 4 0 0 0 | * * * 30 * * ..... xxxxx .....&#xr ♦ 4 4 2 | 0 2 0 4 4 2 2 1 | 0 0 0 2 0 0 2 2 0 1 0 | * * * * 60 * ..... .x.x.5.o.o.&#x ♦ 0 10 0 | 0 0 0 0 0 10 5 0 | 0 0 0 0 0 0 0 0 2 5 0 | * * * * * 12
© 2004-2025 | top of page |