|
Acronym
|
...
|
|
Name
|
p60-r60,
41st stellation of ti
|
|
|
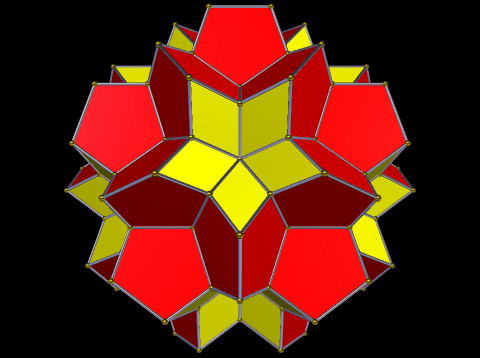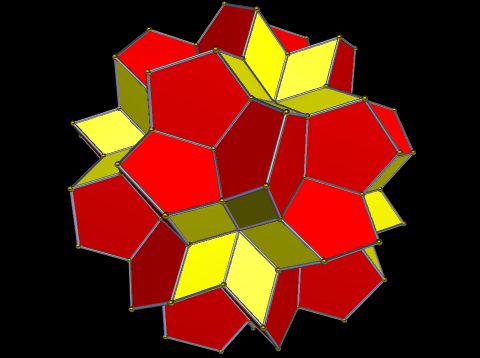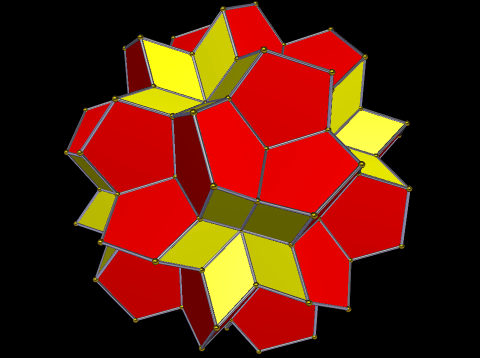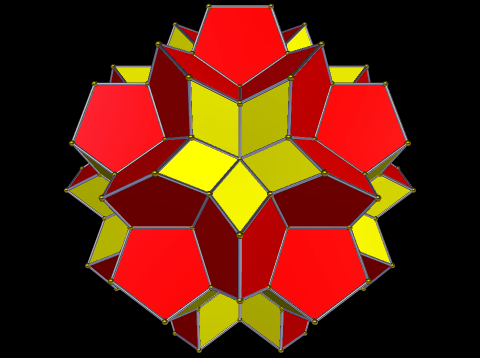 © ©
|
|
Vertex figure
|
[r,52], [R2,52], [r5], [53]
|
|
Dihedral angles
|
- between {5} and {5} at type 2 edges: 360°-arccos(-1/sqrt(5)) = 243.434949°
- between {(r,R)2} and {(r,R)2}: 360°-arccos(-sqrt(5)/3) = 221.810315°
- between {(r,R)2} and {5}: arccos(-sqrt[(5-2 sqrt(5))/15]) = 100.812317°
- between {5} and {5} at type 1 edges: arccos(1/sqrt(5)) = 63.434949°
|
|
Face vector
|
152, 270, 120
|
The rhombs {(r,R)2} have vertex angles r = 60° resp. R = 120°.
Esp. rr : RR = sqrt(3).
–
Alternatively the rhombs could be devided into pairs of coplanar regular triangles, but then asking for an additional edge each.
–
Accordingly, when filling the rhombic dimples halfway with peppies, this polyhedron could be transformed into a non-intersectingly concave polyhedron
xfo3foo5oxf&#zx with regular polygons only.
This concave polyhedron also allows for an expansion (xfoa3fooo5xuFx&#zx), which then still is non-intersectingly concave,
but where all faces then would be regular polygons. Thereby the rhombs will become hexagons.
Incidence matrix according to Dynkin symbol
xfoa3fooo5oxfo&#zx → height = 0
a = sqrt(5) = 2.236068
(tegum sum of (x,f)-ti, (f,x)-srid, f-doe, and a-ike)
o...3o...5o... | 60 * * * | 1 2 0 0 | 2 1 [r,52]
.o..3.o..5.o.. | * 60 * * | 0 2 1 1 | 2 2 [R2,52]
..o.3..o.5..o. | * * 20 * | 0 0 3 0 | 3 0 [53]
...o3...o5...o | * * * 12 | 0 0 0 5 | 0 5 [r5]
-------------------+-------------+--------------+------
x... .... .... | 2 0 0 0 | 30 * * * | 2 0 (type 1)
oo..3oo..5oo..&#x | 1 1 0 0 | * 120 * * | 1 1
.oo.3.oo.5.oo.&#x | 0 1 1 0 | * * 60 * | 2 0 (type 2)
.o.o3.o.o5.o.o&#x | 0 1 0 1 | * * * 60 | 0 2
-------------------+-------------+--------------+------
xfo. .... ....&#xt | 2 2 1 0 | 1 2 2 0 | 60 * {5}
.... .... ox.o&#xt | 1 2 0 1 | 0 2 0 2 | * 60 {(r,R)2}
©