|
Acronym
|
dusidpith
|
|
Name
|
small-prismated-tesseractihexadecachoron dual,
triangular antitegmatic hexacontatetrachoron
|
|
|
 © ©
|
|
Coordinates
|
-
(1, 0, 0, 0) & all permutations, all changes of sign
(vertex-inscribed q3o3o4o (q-hex)
-
(1, 1, 0, 0)/sqrt(2) & all permutations, all changes of sign
(vertex-inscribed o3x3o4o (ico)
-
(1, 1, 1, 0) [1+2 sqrt(2)]/7 & all permutations, all changes of sign
(vertex-inscribed o3o3a4o (a-rit)
-
(1, 1, 1, 1) [2+3 sqrt(2)]/14 & all changes of sign
(vertex-inscribed o3o3o4b (b-tes)
|
|
Dual
|
sidpith
|
|
Face vector
|
80, 208, 192, 64
|
|
Confer
|
- general polytopal classes:
-
Catalan polychora
|
External
links
|


|
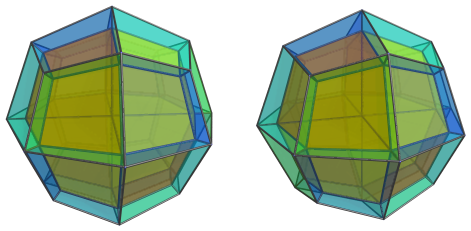
This polychoron can be obtained as the convex hull of a q-hex, a unit ico,
an a-rit, and a b-tes, where a = [4+sqrt(2)]/7 = 0.773459 and b = [2+3 sqrt(2)]/7 = 0.891806.
(All of those sizes so far just describe pseudo edges only.)
Edges then occur between the first two with size c = sqrt[2-sqrt(2)] = 0.765367, between second and third with size d = sqrt[20-2 sqrt(2)]/7 = 0.591980,
and between the last two with size e = sqrt[7+3 sqrt(2)]/7 = 0.479001.
Faces all are deltoidal tetragons with sides c (red) and d (blue) or with sides d (blue) and e (green), while cells all are identical 3-fold axial deltoidal hexahedra (shown on the right)
and have 3 edges of size c, 6 of size d, and 3 of size e.
Incidence matrix according to Dynkin symbol
m3o3o4m =
qooo3oxoo3ooao4ooob&#(c,d,e) → height = 0
a = [4+sqrt(2)]/7 = 0.773459,
b = [2+3 sqrt(2)]/7 = 0.891806,
c = sqrt[2-sqrt(2)] = 0.765367,
d = sqrt[20-2 sqrt(2)]/7 = 0.591980,
e = sqrt[7+3 sqrt(2)]/7 = 0.479001
o...3o...3o...4o... | 8 * * * ♦ 6 0 0 | 12 0 | 8 (type a.)
.o..3.o..3.o..4.o.. | * 24 * * ♦ 2 4 0 | 8 4 | 8 (type b.)
..o.3..o.3..o.4..o. | * * 32 * ♦ 0 3 2 | 3 6 | 6 (type c.)
...o3...o3...o4...o | * * * 16 ♦ 0 0 4 | 0 6 | 4 (type d.)
-----------------------------+------------+----------+-------+---
oo..3oo..3oo..4oo..&#c | 1 1 0 0 | 48 * * | 4 0 | 4 (red)
.oo.3.oo.3.oo.4.oo.&#d | 0 1 1 0 | * 96 * | 2 2 | 4 (blue)
..oo3..oo3..oo4..oo&#e | 0 0 1 1 | * * 64 | 0 3 | 3 (green)
-----------------------------+------------+----------+-------+---
ooo.3ooo.3ooo.4ooo.&#(c,d) | 1 2 1 0 | 2 2 0 | 96 * | 2 {(ccdd)}
.ooo3.ooo3.ooo4.ooo&#(d,e) | 0 1 2 1 | 0 2 2 | * 96 | 2 {(ddee)}
-----------------------------+------------+----------+-------+---
oooo3oooo3oooo4oooo&#(c,d,e) | 1 3 3 1 | 3 6 3 | 3 3 | 64
©