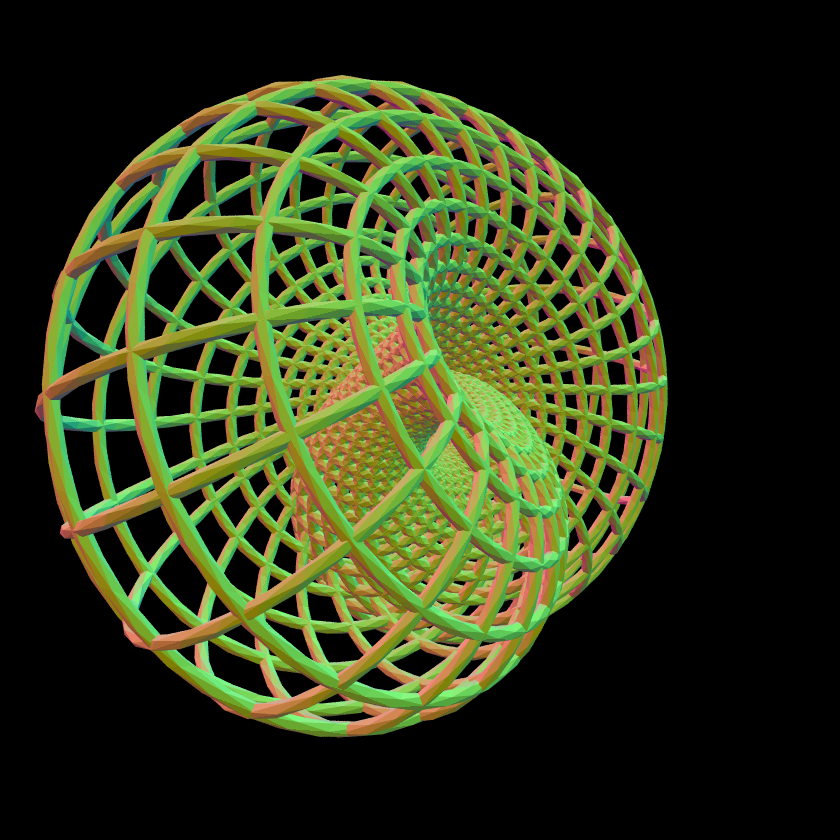
I’ve been designing printable models of the Lawson-Klein surface
x = cos(u) sin(2v)
y = sin(u) cos(v)
z = sin(u) sin(v)
As you can plainly see, this figure lives in S3 (positively curved 3-space), so stereographic projection can bring it into E3 (Euclidean 3-space) without adding more self-intersections. (It crosses itself at u=nπ.)
To minimize the distortion of the projection, I want the projection center to be as far as possible from the surface. One thing I tried was pursuit: starting with an arbitrary point P in S3 and an arbitrary point L(u,v) in the surface, move (u,v) to bring L closer to P while simultaneously moving P away from L. This gets me nowhere so far: either it fails to converge or P converges to the antipodes of L, which is also in the surface (change u by π).
I could take 1e5 samples, generate the convex hull of that point set in E4 (using an existing library), and look for the facet whose hyperplane is nearest to the origin; that hyperplane’s pole is my projection center.
Nelder-Mead optimization has been suggested … and it’s giving me nonsense.