Acronym	skiviphado
Name	small skewverted prismatohexadecadisoctachoron
Cross sections	©
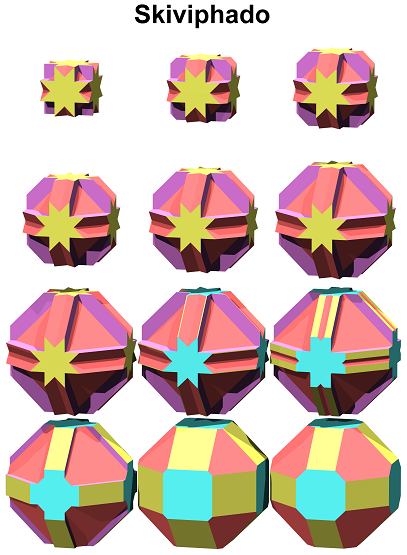Circumradius	sqrt(2) = 1.414214
Coordinates	((1+sqrt(2))/2, 1/2, 1/2, (sqrt(2)-1)/2)   & all permutations, all changes of sign
Colonel of regiment	(is itself locally convex – uniform polychoral members: by cells: co cotco gocco hip oho op querco sirco socco stop skiviphado 16 0 8 0 0 0 0 8 0 24 gikviphado 16 0 0 0 0 24 8 0 8 0 kavahto 0 8 8 0 16 0 0 0 8 0 kaviphdit 0 0 0 32 16 0 8 8 0 0 & others)
Face vector	192, 576, 416, 56
Confer	segmentochora: goccoa cotco   general polytopal classes: Wythoffian polychora
External links

As abstract polytope skiviphado is isomorphic to gikviphado, thereby replacing octagrams by octagons, resp. replacing the sirco by querco, gocco by socco, and stop by op.

Skiviphado itself, in its cubical direction, comes within 6 consecutive vertex layers: gocco || cotco || sirco || sirco || cotco || gocco. The polar caps clearly are goccoa cotco each. The medial tristratic remainder then is the pabdi skiviphado.

Incidence matrix according to Dynkin symbol

x3o3x4/3x4*b

. . .   .    | 192 |   2   2   2 |  1  2  2  1  1  2 |  1 1  2 1
-------------+-----+-------------+-------------------+----------
x . .   .    |   2 | 192   *   * |  1  1  1  0  0  0 |  1 1  1 0
. . x   .    |   2 |   * 192   * |  0  1  0  1  0  1 |  1 0  1 1
. . .   x    |   2 |   *   * 192 |  0  0  1  0  1  1 |  0 1  1 1
-------------+-----+-------------+-------------------+----------
x3o .   .    |   3 |   3   0   0 | 64  *  *  *  *  * |  1 1  0 0
x . x   .    |   4 |   2   2   0 |  * 96  *  *  *  * |  1 0  1 0
x . .   x    |   4 |   2   0   2 |  *  * 96  *  *  * |  0 1  1 0
. o3x   .    |   3 |   0   3   0 |  *  *  * 64  *  * |  1 0  0 1
. o .   x4*b |   4 |   0   0   4 |  *  *  *  * 48  * |  0 1  0 1
. . x4/3x    |   8 |   0   4   4 |  *  *  *  *  * 48 |  0 0  1 1
-------------+-----+-------------+-------------------+----------
x3o3x   .    ♦  12 |  12  12   0 |  4  6  0  4  0  0 | 16 *  * *
x3o .   x4*b ♦  24 |  24   0  24 |  8  0 12  0  6  0 |  * 8  * *
x . x4/3x    ♦  16 |   8   8   8 |  0  4  4  0  0  2 |  * * 24 *
. o3x4/3x4*b ♦  24 |   0  24  24 |  0  0  0  8  6  6 |  * *  * 8

x3/2o3/2x4/3x4/3*b

.   .   .   .      | 192 |   2   2   2 |  1  2  2  1  1  2 |  1 1  2 1
-------------------+-----+-------------+-------------------+----------
x   .   .   .      |   2 | 192   *   * |  1  1  1  0  0  0 |  1 1  1 0
.   .   x   .      |   2 |   * 192   * |  0  1  0  1  0  1 |  1 0  1 1
.   .   .   x      |   2 |   *   * 192 |  0  0  1  0  1  1 |  0 1  1 1
-------------------+-----+-------------+-------------------+----------
x3/2o   .   .      |   3 |   3   0   0 | 64  *  *  *  *  * |  1 1  0 0
x   .   x   .      |   4 |   2   2   0 |  * 96  *  *  *  * |  1 0  1 0
x   .   .   x      |   4 |   2   0   2 |  *  * 96  *  *  * |  0 1  1 0
.   o3/2x   .      |   3 |   0   3   0 |  *  *  * 64  *  * |  1 0  0 1
.   o   .   x4/3*b |   4 |   0   0   4 |  *  *  *  * 48  * |  0 1  0 1
.   .   x4/3x      |   8 |   0   4   4 |  *  *  *  *  * 48 |  0 0  1 1
-------------------+-----+-------------+-------------------+----------
x3/2o3/2x   .      ♦  12 |  12  12   0 |  4  6  0  4  0  0 | 16 *  * *
x3/2o   .   x4/3*b ♦  24 |  24   0  24 |  8  0 12  0  6  0 |  * 8  * *
x   .   x4/3x      ♦  16 |   8   8   8 |  0  4  4  0  0  2 |  * * 24 *
.   o3/2x4/3x4/3*b ♦  24 |   0  24  24 |  0  0  0  8  6  6 |  * *  * 8