©
| Acronym | ... |
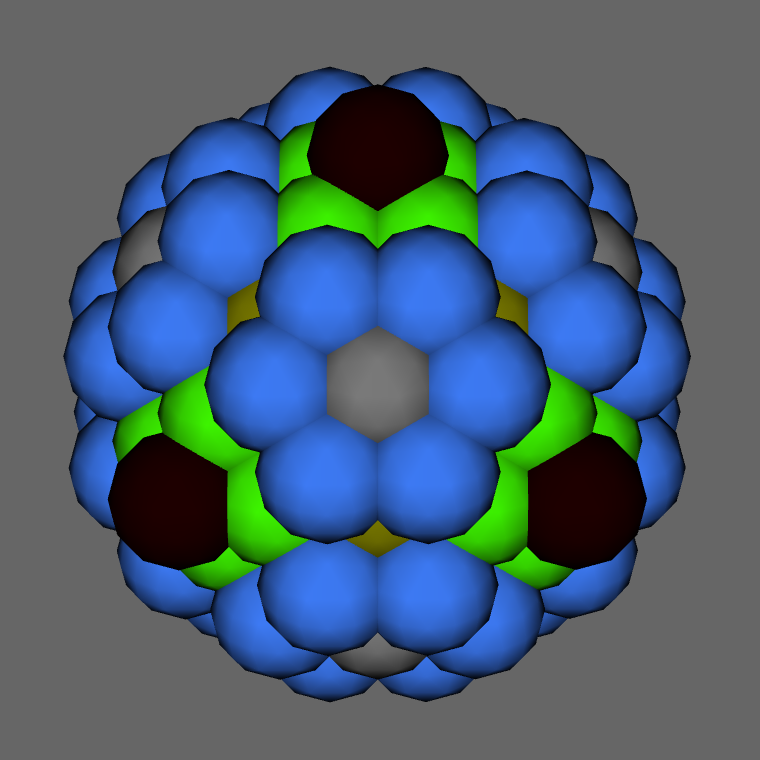
| Name | Waterman polyhedron number 8 wrt. face-centered cubic lattice A3 centered at a lattice point |
| |
| Face vector | 54, 120, 68 |
The unit here was chosen as the root of A3.
By the very definition of Waterman polyhedra, not necessarily all vertices are on the same sphere. In here the 6 maximal ones (black vertices) have a circumradius of sqrt(8) = 2.828427, while the other 48 vertices (blue ones) only are at an radius of sqrt(7) = 2.645751.
Incidence matrix according to Dynkin symbol
ax3ox4oq&#zh → height = 0, where a = 4 (pseudo) (tegum sum of a-oct and (x,x,q)-girco) o.3o.4o. | 6 * | 8 0 0 0 | 4 4 0 0 (black) .o3.o4.o | * 48 | 1 1 1 1 | 1 1 1 1 (blue) ------------+------+-------------+----------- oo3oo4oo&#h | 1 1 | 48 * * * | 1 1 0 0 h .x .. .. | 0 2 | * 24 * * | 0 0 1 1 x .. .x .. | 0 2 | * * 24 * | 1 0 1 0 x .. .. .q | 0 2 | * * * 24 | 0 1 0 1 q ------------+------+-------------+----------- .. ox ..&#h | 1 2 | 2 0 1 0 | 24 * * * .. .. oq&#h | 1 2 | 2 0 0 1 | * 24 * * .x3.x .. | 0 6 | 0 3 3 0 | * * 8 * .x .. .q | 0 4 | 0 2 0 2 | * * * 12
© 2004-2025 | top of page |