Acronym	...
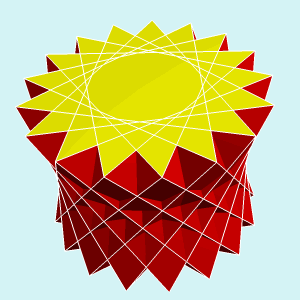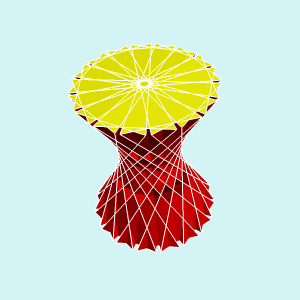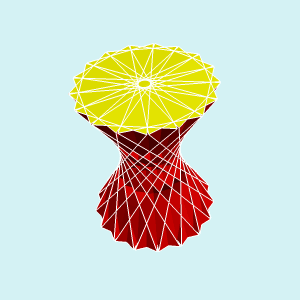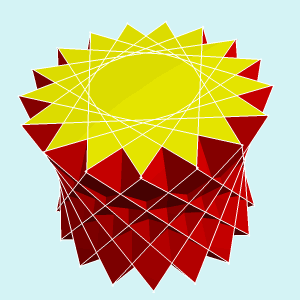
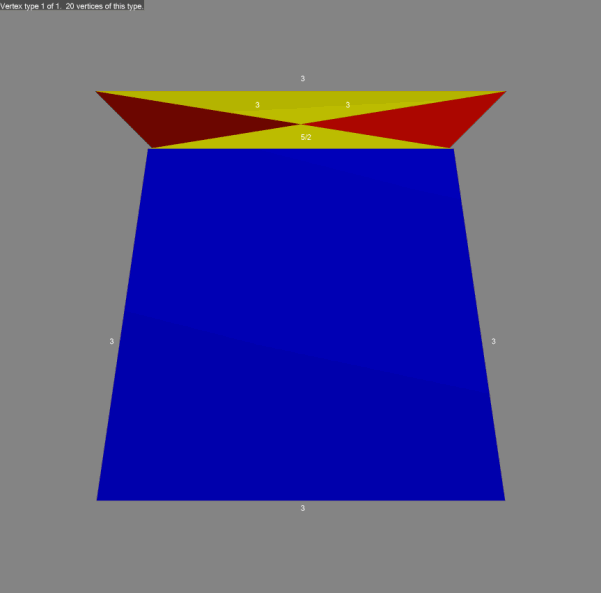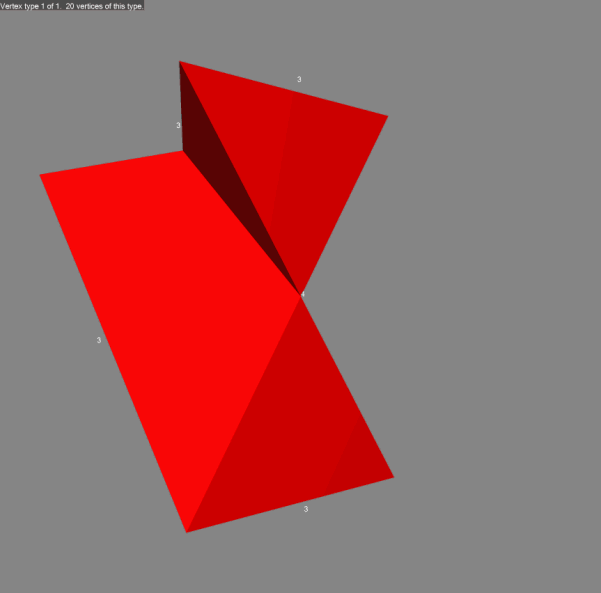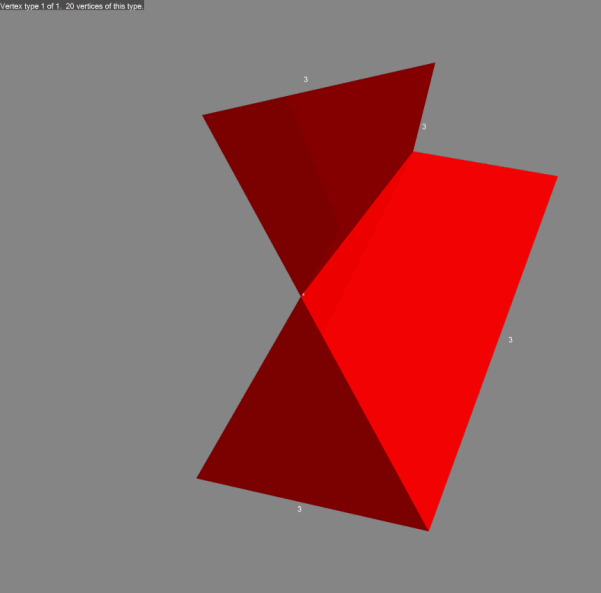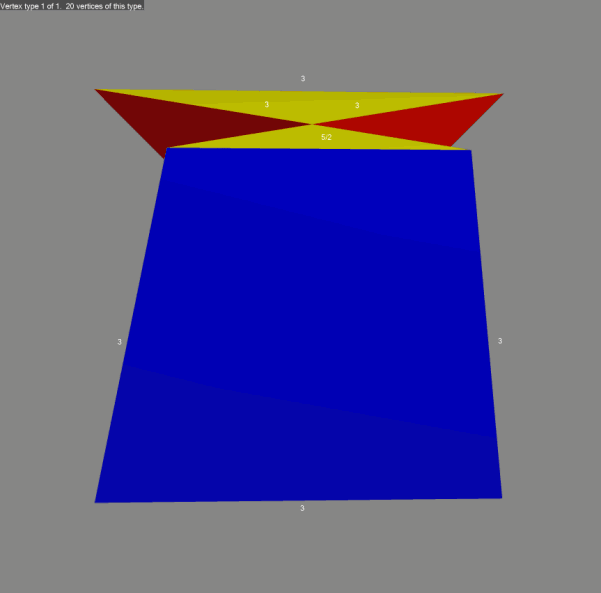
Name	n/d-grammic retroantiprismatic hemiantiprism
	©
Circumradius	1/sqrt(2) = 0.707107
Vertex figure	©
Lace city in approx. ASCII-art	x-n/d-o o-n/d-x -- n/d-ap o-n/d-x x-n/d-o -- n/d-ap | +-- n/(n-d)-ap +-------------- n/(n-d)-ap
Face vector	4n, 12n, 10n+4, 2n+4
Especially	hatho (n/d = 2)

In November 2020 this series of non-convex scaliform polychora has been found, existing for any 2 < n/d < 3. The extremal case n/d = 2 (hatho) would exist as well and so conceptually would belong here too, but has a slightly different incidence matrix (because of degeneracy of the base). In the other extremal case n/d = 3 the height of the retroantiprism would become zero.

By means of this series scaliform polychora finally have shot up to infinity now too.

Its hull will be a digon - n-gon duoantiprism.

Incidence matrix

4n |  2  2  2 |  3  3  2 1 | 1 1  3
---+----------+------------+-------
 2 | 4n  *  * |  2  0  1 0 | 1 0  2  n/d-ap lacings
 2 |  * 4n  * |  0  2  1 0 | 0 1  2  n/(n-d)-ap lacings
 2 |  *  * 4n |  1  1  0 1 | 1 1  1  n/d-sides
---+----------+------------+-------
 3 |  2  0  1 | 4n  *  * * | 1 0  1  n/d-ap triangles
 3 |  0  2  1 |  * 4n  * * | 0 1  1  n/(n-d)-ap triangles
 4 |  2  2  0 |  *  * 2n * | 0 0  2
 n |  0  0  n |  *  *  * 4 | 1 1  0
---+----------+------------+-------
2n | 2n  0 2n | 2n  0  0 2 | 2 *  *  n/d-ap
2n |  0 2n 2n |  0 2n  0 2 | * 2  *  n/(n-d)-ap
 6 |  4  4  2 |  2  2  2 0 | * * 2n  bobipyr